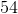Call Now to Set Up Tutoring:

(888) 888-0446

Calculus 1 : Velocity

Study concepts, example questions & explanations for Calculus 1

Create An Account

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2 : Calculus Review

Consider the position function {}p(t) , which describes the positon of an oxygen molecule.

p(t)=14t^3-14t^2+14t-14

Find the function which models the velocity of the oxygen molecule.

Possible Answers:

v(t)=-28t+14

v(t)=42t^2-28t+14

v(t)=84t-28

v(t)=42t^2+14

v(t)=14

Correct answer:

v(t)=42t^2-28t+14

Explanation:

Recall that velocity is the first derivative of position and acceleration is the second derivative of position.

So given:

p(t)=14t^3-14t^2+14t-14

Apply the power rule to each term to find the velocity.

$f(x)=x^n \rightarrow f'(x)=nx^{n-1}$

v(t)=p'(t)=42t^2-28t+14

Report an Error

Example Question #41 : Calculus

Consider the position function {}p(t) , which describes the positon of an oxygen molecule.

p(t)=14t^3-14t^2+14t-14

Find the velocity of the oxygen molecule after seconds.

Possible Answers:

574

2590

57.4

Correct answer:

574

Explanation:

Recall that velocity is the first derivative of position and acceleration is the second derivative of position.

So given:

p(t)=14t^3-14t^2+14t-14

v(t)=p'(t)=42t^2-28t+14

To find the velocity after 4 seconds, find v(4)

$v(4)=42\cdot 4^2-28\cdot 4+14=574$

Report an Error

Example Question #42 : How To Find Velocity

The displacement of an object at time is defined by the function $f(t) = e^{(3t)}$ . What is the velocity at t=1 ?

Possible Answers:

Correct answer:

Explanation:

The velocity is defined as the derivative of the displacement equation. With the displacement equation $f(t) = e^{3t}$ , we can use the power rule to differentiate.

Remember that $f(x)=e^x \rightarrow f'(x)=e^x \cdot {dx}$ .

In our case our $x=3t \rightarrow \frac{dx}{dt}=3$

Thus, the velocity equation is defined as:

$f'(t) = 3e^{3t}$ . Now t=1 can be used in the velocity equation.

$f'(1) = 3e^{3} \approx 3 \cdot 20 = 60$

The velocity at t=1 is 60.

Report an Error

Example Question #42 : Spatial Calculus

The position of an object is given by the equation $f(t) = \sin^2(x)$ . What is the velocity of the object?

Possible Answers:

$\sin(t)$

$2\sin(t)$

$\cos^2(t)$

$2\sin(t)\cos(t)$

Correct answer:

$2\sin(t)\cos(t)$

Explanation:

The velocity of the object can be found by differentiating the equation $f(t) = \sin^2(t)$ . We must use the chain rule and the power rule to differentiate this equation.

We can rewrite the equation as

f(t)=(sin(t))^2 using the power rule we get:

f'(t)=2sin(t) from here we need to use the chain rule and multiply this by the derivative of the inside piece which is the sine function. Since the derivative of sine is cosine we get the following:

$f'(t)=2sin(t)\cdot cos(t)$

Thus, the velocity equation is:

$f'(t) = 2\sin(t)\cos(t)$

Report an Error

Example Question #41 : Velocity

The position of an object is given by the equation $f(t) = 2\sin(t)$ . What is the velocity of the object at t=0 ?

Possible Answers:

Correct answer:

Explanation:

The velocity equation can be found by differentiating the equation for position $f(t) = 2\sin(t)$ .

This gives the equation

$f'(t) = 2\cdot \cos(t)$

At t=0

$f'(0) = 2\cos(0) = 2\cdot 1 = 2$

Report an Error

Example Question #45 : How To Find Velocity

The acceleration of an object is given by the equation f'(t) = t^2 - t^3 . What is the velocity equation of the object if it has an initial velocity of ?

Possible Answers:

$f(t) = \frac{t^3}{3}-\frac{t^4}{4}+2$

f(t) = 6

f(t) = 2t - 3t^2

$f(t) = \frac{t^3}{3}-\frac{t^4}{4}$

Correct answer:

$f(t) = \frac{t^3}{3}-\frac{t^4}{4}+2$

Explanation:

The velocity equation can be found by integrating the acceleration equation.

Differentiating the equation f'(t) = t^2 - t^3 gives

$f(t) = \frac{t^3}{3}- \frac{t^4}{4}+C$

Using the initial velocity of the equation gives us

f(0) = 0 - 0 +C = 2

C = 2

The complete equation for the velocity is

$f(t) = \frac{t^3}{3} - \frac{t^4}{4} +2$

Report an Error

Example Question #46 : How To Find Velocity

If the acceleration of an object is $-3 \frac{m}{s}$ , and its acceleration function is a(t)=4t-6 , what is the velocity of the object?

Possible Answers:

$\frac{17}{54}$

$-\frac{63}{8}$

$-\frac{27}{8}$

Correct answer:

$-\frac{27}{8}$

Explanation:

To find the velocity from the acceleration function, integrate the acceleration function a(t)=4t-6 . To integrate, remember to add an exponent to each term and divide the whole term by the value of the exponent.

v(t)= 2t^2-6t

We will also need to know the time when the object at $-3 \frac{m}{s}$ .

Substitute this value back into the acceleration function and solve for time.

-3=4t-6

3=4t

$t=\frac{3}{4}$

Substitute this value to the velocity function to find the velocity.

$v\left(t=\frac{3}{4}\right)=2\left(\frac{3}{4}\right)^2-6\left(\frac{3}{4}\right)=2\left(\frac{9}{16}\right)-\frac{18}{4}=\frac{9}{8}-\frac{36}{8}$

$v=-\frac{27}{8}$

Report an Error

Example Question #47 : How To Find Velocity

If the position function of a ball is s(t)=3t^2+4t+5 , what is the velocity of the ball when t=0 ?

Possible Answers:

Correct answer:

Explanation:

To find the velocity function, take the derivative of the position function: s(t)=3t^2+4t+5

Remember when integrating, increase the exponent of each term by one and then divide the term by the value of the new exponent.

v(t)=6t+4

Substitute the time into the velocity function.

v(t=0)=6(0)+4=4

Report an Error

Example Question #48 : How To Find Velocity

The acceleration of an object is given by the equation f(t) = -2t . What is the velocity of the object at t=10 if its initial velocity is ?

Possible Answers:

{}-50

150

2600

2400

Correct answer:

{}-50

Explanation:

Using the acceleration equation, f'(t) = -2t we can find the velocity equation by taking the integral.

$f(t) = \frac{-2t^{1+1}}{2} + C = -t^2 +C\cdot$

Now using the initial velocity of 50, we can find

f(0) = -(0)^2+C = 50

C = 50

Now we can solve for the velocity at t=10

f(10) = -(10)^2+50 = -50

Report an Error

Example Question #42 : How To Find Velocity

The position equation for an object is $f(t) = \sin^2(\pi e^t)$ . What is the velocity of the object at t = 0 ?

Possible Answers:

$-2 \pi$

$2 \pi$

Correct answer:

Explanation:

The velocity of the object can be found by differentiating the position equation. To differentiate this equation, the power rule and the chain rule must be used.

The power rule says that

$f(x) = x^{n} \rightarrow f'(x) = nx^{n-1}$ .

The chain rule says that

$f(x) = h(g(x)) \rightarrow f'(x) = h'(g(x))\times g'(x)$ .

Differentiating the equation $f(t) = \sin^2(\pi e^t)$ , gives

$f'(t) = 2\sin^{(2-1)}(\pi e^t)\cos(\pi e^t)\pi e^t =2\sin(\pi e^t)\cos(\pi e^t)\pi e^t = 2\pi e^t\sin(\pi e^t)\cos(\pi e^t)$ .

Using 0 as the value for t gives us

$f'(0) = 2\pi e^0\sin(\pi e^0)\cos(\pi e^0) = 2\cdot\pi\cdot \sin(\pi)\cdot\cos(\pi) = 0$

Report an Error

View Calculus Tutors

Tajrin
Certified Tutor

CUNY Brooklyn College, Bachelor of Science, Computer Science.

View Calculus Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

View Calculus Tutors

Tracy
Certified Tutor

Clarion University of Pennsylvania, Bachelor of Science, Physics.

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

English Tutors in Washington DC, ISEE Tutors in Phoenix, Calculus Tutors in New York City, French Tutors in Boston, GRE Tutors in Houston, GRE Tutors in Chicago, GMAT Tutors in Miami, LSAT Tutors in New York City, SAT Tutors in Houston, Calculus Tutors in Miami

Popular Courses & Classes

SSAT Courses & Classes in Denver, ISEE Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Miami, Spanish Courses & Classes in Chicago, LSAT Courses & Classes in Atlanta, ACT Courses & Classes in New York City, ISEE Courses & Classes in New York City, Spanish Courses & Classes in Washington DC, GMAT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Philadelphia

Popular Test Prep

SAT Test Prep in Houston, ISEE Test Prep in Seattle, ACT Test Prep in New York City, GRE Test Prep in Miami, MCAT Test Prep in Philadelphia, MCAT Test Prep in Chicago, MCAT Test Prep in Washington DC, GRE Test Prep in Dallas Fort Worth, GMAT Test Prep in Philadelphia, GRE Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Calculus 1 : Velocity

Study concepts, example questions & explanations for Calculus 1

All Calculus 1 Resources

Example Questions

Example Question #2 : Calculus Review

Example Question #41 : Calculus

Example Question #42 : How To Find Velocity

Example Question #42 : Spatial Calculus

Example Question #41 : Velocity

Example Question #45 : How To Find Velocity

Example Question #46 : How To Find Velocity

Example Question #47 : How To Find Velocity

Example Question #48 : How To Find Velocity

Example Question #42 : How To Find Velocity

All Calculus 1 Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: