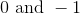All Calculus 1 Resources
Example Questions
Example Question #1662 : Functions
Given
Find the 

Cannot be determined
To find relative maximums, we need to find where our first derivative changes sign. To do this, find your first derivative and then find where it is equal to zero.
Begin with:

This means we have extrema at x=0 and x=-8/3
Because we are only concerned about the interval from -5 to 0, we only need to test points on that interval. Try points between our two extrema, as well as one between -8/3 and -5.
So our first derivative goes from negative to positive at -8/3, thus this is the x coordinate of our relative maximum on this interval.
Example Question #1 : How To Find Relative Maximum On The Interval By Graphing Functions
Where is the maximum value of the function
on the interval ![[-2, 2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/369960/gif.latex)

Though there are relative maxima at 



Example Question #3 : Relative Extremum
Determine the relative maxima for the function:
To determine the relative maxima for the function, we must determine where the first derivative of the function changes from positive to negative.
The first derivative of the function is
and was found using the following rule:
Next, we must find the critical points, the values at which the first derivative is equal to zero:
Using the critical values, we can then create the intervals on which we evaluate the sign of the first derivative:
To check the sign of the first derivative, plug in any value on each interval into the first derivative function. On the first interval, the first derivative is positive, on the second, it is negative, and on the third it is positive. The first derivative changes from positive to negative at 
Example Question #2 : How To Find Relative Maximum On The Interval By Graphing Functions
Find the local max of

using the equation and/or the following graph.

We notice that this function has 2 extrema which are located at 


We know that extrema exist when the slope of the function is zero, hence we take the derivative, set it equal to zero, and than solve for x.
The derivative is the following:

Therefore setting both pieces equal to zero we see the following:


Finding out if one of these x values produce a local min or max however requires either the first derivative test, the second derivative test, or analyzing the graph. We see that for a small neighborhood around 




Therefore when 
Example Question #2 : Relative Extremum
The following is a list of values at certain points for continuous 
Exactly 1
Exactly 2
At least 2
At most 2
At least 1
At least 2
The mean value theorem states that if continuous 


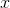

Example Question #6 : Relative Extremum
A relative minimum of a function is all the points x, in the domain of the function, such that it is the smallest value for some neighborhood. These are points in which the first derivative is 0 or it does not exist.
Find the relative minimum of the function

using the following graph and the function.

We notice that this function has 2 extrema which are located at x=-1 and x=0. We could have also of found this by looking at the function itself:

We know that extrema exist when the slope of the function is zero, hence we take the derivative, set it equal to zero, and than solve for x.
The derivative is the following:

Therefore setting both pieces equal to zero we see the following:


Finding out if one of these x values produce a local min or max however requires either the first derivative test, the second derivative test, or analyzing the graph. We see that for a small neighborhood around 
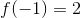



Certified Tutor
All Calculus 1 Resources