All Calculus 1 Resources
Example Questions
Example Question #25 : Intervals
Is the function g(x) increasing or decreasing on the interval ![[-5,0]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/645173/gif.latex)
Decreasing, because g'(x) is positive on the given interval.
Increasing, because g'(x) is negative on the given interval.
Decreasing, because g'(x) is negative on the given interval.
Increasing, because g'(x) is positive on the given interval.
Decreasing, because g'(x) is negative on the given interval.
Is the function g(x) increasing or decreasing on the interval ![[-5,0]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/645175/gif.latex)
To tell if a function is increasing or decreasing, we need to see if its first derivative is positive or negative. let's find g'(x)
Recall that the derivative of a polynomial can be found by taking each term, multiplying by its exponent and then decreasing the exponent by 1.
Next, we need to see if the function is positive or negative over the given interval.
Begin by finding g'(-5)
So, g'(-5) is negative, but what about g'(0)?
Also negative, so our answer is:
Decreasing, because g'(x) is negative on the interval .
Example Question #1 : Increasing Intervals
On what intervals does f(x) = (1/3)x3 + 2.5x2 – 14x + 25 increase?
(–∞, –7) and (2, ∞)
(–∞, –7), (–7, 2), and (2, ∞)
(–∞, –7)
(–7, 2), and (2, ∞)
(2, ∞)
(–∞, –7) and (2, ∞)
We will use the tangent line slope to ascertain the increasing / decreasing of f(x). To this end, let us begin by taking the first derivative of f(x):
f'(x) = x2 + 5x – 14
Solve for the potential relative maxima and minima by setting f'(x) to 0 and solving:
x2 + 5x – 14 = 0; (x – 2)(x + 7) = 0
Potential relative maxima / minima: x = 2, x = –7
We must test the following intervals: (–∞, –7), (–7, 2), (2, ∞)
f'(–10) = 100 – 50 – 14 = 36
f'(0) = –14
f'(10) = 100 + 50 – 14 = 136
Therefore, the equation increases on (–∞, –7) and (2, ∞)
Example Question #2 : Increasing Intervals
Find the interval(s) where the following function is increasing. Graph to double check your answer.
Always
Never
To find when a function is increasing, you must first take the derivative, then set it equal to 0, and then find between which zero values the function is positive.
First, take the derivative:
Set equal to 0 and solve:
Now test values on all sides of these to find when the function is positive, and therefore increasing. I will test the values of -6, 0, and 2.
Since the values that are positive is when x=-6 and 2, the interval is increasing on the intervals that include these values. Therefore, our answer is:
Example Question #3 : Increasing Intervals
Find the interval(s) where the following function is increasing. Graph to double check your answer.
Never
Always
To find when a function is increasing, you must first take the derivative, then set it equal to 0, and then find between which zero values the function is positive.
First, take the derivative:
Set equal to 0 and solve:
Now test values on all sides of these to find when the function is positive, and therefore increasing. I will test the values of 0, 2, and 10.
Since the value that is positive is when x=0 and 10, the interval is increasing in both of those intervals. Therefore, our answer is:
Example Question #21 : Intervals
Is 

Cannot be determined from the information provided
Decreasing. 

Increasing. 

Decreasing. 

Increasing. 

Increasing. 

To find increasing and decreasing intervals, we need to find where our first derivative is greater than or less than zero. If our first derivative is positive, our original function is increasing and if g'(x) is negative, g(x) is decreasing.
Begin with:
If we plug in any number from 3 to 6, we get a positve number for g'(x), So, this function must be increasing on the interval {3,6}, because g'(x) is positive.
Example Question #1 : How To Find Increasing Intervals By Graphing Functions
Is 
![[-5,-8]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/325742/gif.latex)
Increasing, because 
Increasing, because 
Decreasing, because 
Decreasing, because 

Increasing, because 
To find out if a function is increasing or decreasing, we need to find if the first derivative is positive or negative on the given interval.
So starting with:
We get:


Find the function on each end of the interval.
So the first derivative is positive on the whole interval, thus g(t) is increasing on the interval.
Example Question #1 : How To Find Increasing Intervals By Graphing Functions
Is the following function increasing or decreasing on the interval ![[2,3]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/325776/gif.latex)
The function is neither increasing nor decreasing on the interval.
Increasing, because 
Increasing, because 
Decreasing, because 
Decreasing, because 
Increasing, because 
A function is increasing on an interval if for every point on that interval the first derivative is positive.
So we need to find the first derivative and then plug in the endpoints of our interval.
Find the first derivative by using the Power Rule
Plug in the endpoints and evaluate the function.
Both are positive, so our function is increasing on the given interval.
Example Question #4 : How To Find Increasing Intervals By Graphing Functions
On which intervals is the following function increasing?
The first step is to find the first derivative.
Remember that the derivative of
Next, find the critical points, which are the points where 




The final step is to try points in all the regions 

If we plugin in a number from the first range, i.e 
From the second range, 
From the third range, 
From the last range, 
So the second and the last ranges are the ones where 
Example Question #8 : How To Find Increasing Intervals By Graphing Functions
Below is the complete graph of 

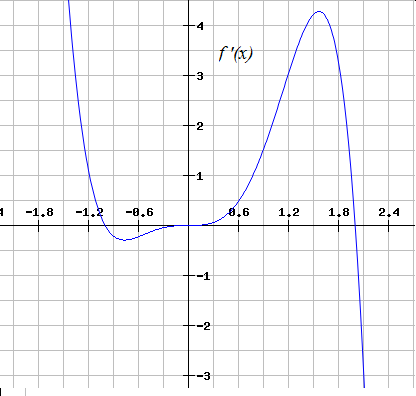




Example Question #9 : How To Find Increasing Intervals By Graphing Functions
Function A
Function B
Function C
Function D
Function E
5 graphs of different functions are shown above. Which graph shows an increasing/non-decreasing function?
Function A
Function E
Function D
Function C
Function B
Function E
A function 


Function E is the only function that has this property. Note that function E is increasing, but not strictly increasing
All Calculus 1 Resources


































































