All Basic Geometry Resources
Example Questions
Example Question #171 : Triangles
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 
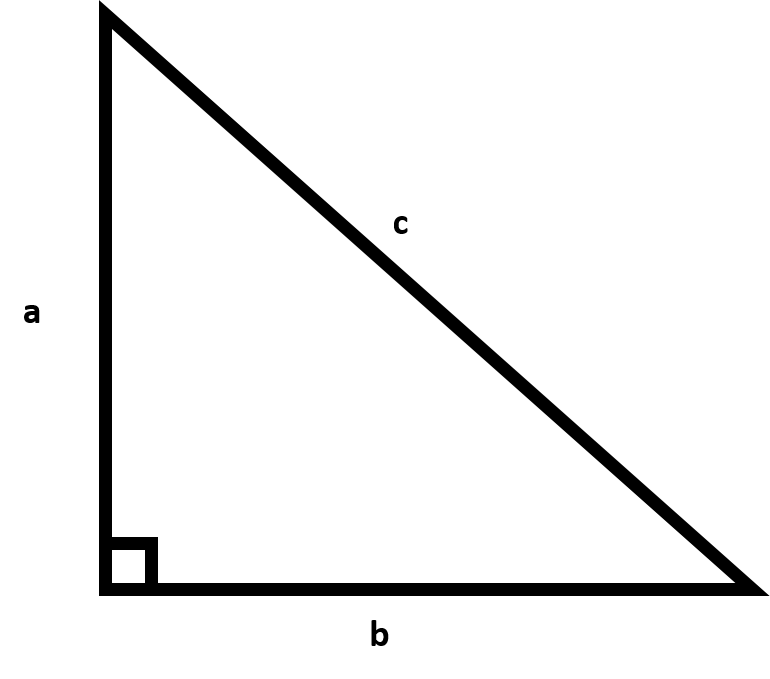
To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #9 : How To Find The Length Of The Side Of A 45/45/90 Right Isosceles Triangle
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #10 : How To Find The Length Of The Side Of A 45/45/90 Right Isosceles Triangle
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #1151 : Basic Geometry
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #1152 : Basic Geometry
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #1153 : Basic Geometry
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #1154 : Basic Geometry
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #1161 : Basic Geometry
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #1162 : Basic Geometry
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
Example Question #1163 : Basic Geometry
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 

To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
All Basic Geometry Resources



















































































