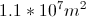AP Physics C: Mechanics : Electric Circuits
Study concepts, example questions & explanations for AP Physics C: Mechanics
All AP Physics C: Mechanics Resources
Example Questions
Example Question #1 : Electric Circuits
A parallel plate capacitor has a capacitance of 
The relationship between capacitance, distance, and area is 
Now, we can use the values given in teh question to solve.
Example Question #1 : Capacitors
Charge is distributed uniformly over the area of the two plates of a parallel plate capacitor, resulting in a surface area charge density of 
Which of the following would not result in an increase in the measure of electric potential difference between the two plates?
Increase the value of the surface charge density, 
Increase the area, 
Increase the distance, 
Replace the material between the two plates with one of a lower dielectric constant,
Increase the area,
The electric potential difference created between the plates of a parallel plate capactor is given by the equation:
The charge can be calculated by using the equation:
The value of the capacitance is related to the dimensions of the capacitor with the equation:
Combining these equations yields:
The area becomes inconsequential, while the potential is directly proportional to the surface charge density and the distance between the plates, and inversely proportional to the dielectric of the material between the plates. Changing the area does not cause any change in the potential difference measured between the plates, and changing any of the other variables would cause a resultant change in the potential difference.
Example Question #1 : Capacitors
You are hired to make a capacitor out of two parallel metal sheets. If someone wanted you to make a thin capacitor of 
For parallel plate capacitors, the equation is 
Solve for 
Now we can plug in our given values.
Example Question #1 : Electric Circuits
The plates of a parallel plate capacitor are 
Capacitance is related to plate area and distance by the equation 
Given the area and distance, we can solve for capacitance. The voltage, in this case, is irrelevant.
Example Question #51 : Electricity And Magnetism Exam
The plates of a parallel plate capacitor are 
Charge on a capacitor is given by the equation 
We can plug this value into the equation for charge.
Example Question #52 : Electricity And Magnetism Exam
The plates of a parallel plate capacitor are 
The electric field given by a capacitor is given by the formula 
The capacitance can be determined by the area of the plates and the distance between them.
This equation simplifies to 
Example Question #4 : Capacitors
If two identical parallel plate capacitors of capacitance 
Relevant equations:
Use the series equation, replacing C1 and C2 with the given constant C:
This agrees with the general rule that the equivalent capacitance in series is less than the capacitance of any of the individual capacitors.
Example Question #4 : Capacitors
Two parallel conducting plates each have an area of 
Relevant equations:
According to the work-energy theorem, work done on the proton is equal to its change in kinetic energy.
1. Find an expression for potential difference, 
2. Multiply the potential difference times the proton charge to find work (and thus kinetic energy):
Example Question #3 : Electric Circuits
A 
Relevant equations:
First, find the equivalent capacitance of the whole circuit:
Use this equivalent capacitance to find the total charge:
Capacitors in series always have the same charge on each unit, so the charge on the 
Example Question #1 : Resistors
Resistors are one of the most important basic components of a circuit. With very few exceptions, all circuits have at least one kind of resistor component. An ammeter is a device that measures current flowing through a circuit. Ammeters are always connected to a circuit in series.
Which of the following accurately explains why ammeters must be connected in series within a circuit, and never in parallel?
An ammeter connected in parallel would give readings of half the current flowing through the circuit because current would flow evenly between the ammeter and the circuit's regular pathway
An ammeter connected in parallel would give high readings of current because the current would be flowing through both the regular current path and the ammeter, but disproportionately through the ammeter
An ammeter connected in parallel would give the current flowing through the circuit a pathway with minimal resistance, creating a very large current that could harm the ammeter
An ammeter connected in parallel would give very low readings of current because current would be flowing through both the regular current path and the ammeter, but disproportionately through the regular path
An ammeter connected in parallel would not read any current because the current would have no driving force through the ammeter
An ammeter connected in parallel would give the current flowing through the circuit a pathway with minimal resistance, creating a very large current that could harm the ammeter
In order to give accurate readings of current, ammeters have very low resistances. If connected in parallel, the voltage pushing current through the circuit would push a very strong current through the ammeter and virtually no current through the circuit's regular path. This would not only lead to a bad reading of current, but more often than not a broken ammeter. This phenomenon is an indication of why resistors are so important: they limit the current such that a circuit does not exceed its current-carrying capacity.
Certified Tutor
All AP Physics C: Mechanics Resources
