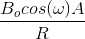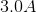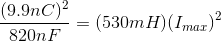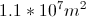Call Now to Set Up Tutoring:

(888) 888-0446

AP Physics C Electricity : Electricity and Magnetism Exam

Study concepts, example questions & explanations for AP Physics C Electricity

Create An Account

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2 : Electromagnetism

A wire loop of area is placed in a magnetic field whose magnitude varies with time according to the equation $B(t) = B_o sin(\omega t)$ . The loop is held in place, perpendicular to the field. If the wire has resistance , what is the magnitude of the maximum instantaneous current induced in the loop?

Possible Answers:

$\frac{B_o A}{\omega R}$

$\frac{B_o\omega A}{R}$

$\frac{B_o sin(\omega) A}{R}$

$\frac{B_o \omega}{AR}$

$\frac{B_o cos(\omega ) A}{R}$

Correct answer:

$\frac{B_o\omega A}{R}$

Explanation:

Relevant equations:

$|\epsilon| = \frac{d\phi}{dt} = \frac{d (B\cdot A)}{dt}$

$\phi = B \cdot A$

$\epsilon = IR$

Write the expression for the flux using the given expression for the magnetic field strength:

$\phi = B\cdot A = B_o sin(\omega t)A$

Take the derivative of this flux to find the induced emf:

$|\epsilon | = \frac{d\phi}{dt} = \frac{d (B_o sin(\omega t) A)}{dt} = B_o \omega cos(\omega t) A$

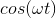
Determine the maximum value of $cos(\omega t)$ , and replace $cos(\omega t)$ with this value, to find the maximum induced emf:

$|\epsilon | _{max} = {B_o \omega * 1 * A} = B_o \omega A$

Plug this maximum induced emf into $\epsilon = IR$ , to find the maximum induced current:

$\epsilon = IR \rightarrow I = \frac{\epsilon}{R} = \frac{B_o \omega A}{R}$

Report an Error

Example Question #201 : Ap Physics C

A wire loop carrying no current is placed near another very long straight wire carrying current . The loop is then moved directly away from the straight wire at a constant speed.

Ps0_lenz

In which direction will the current induced in the small wire loop flow?

Possible Answers:

Counterclockwise

No current will be induced in the wire loop

Clockwise

Not enough information is given to solve

Correct answer:

Clockwise

Explanation:

The magnetic field due to the long straight wire points into the page (right-hand rule) in the region below it in the picture. The magnitude of this magnetic field is given by:

$B=\frac{\mu _{oI}}{2\pi r}$

The magnetic field thus decreases in value with increasing distance, . As such, the amount of flux passing through the wire loop decreases steadily as it is moved away from the wire.

According to Lenz's Law, an induced current within the wire loop will counteract this loss of magnetic flux from the straight wire. The magnetic field from the induced current in the wire loop will consequently point in the same direction as that from the current in the straight wire. The right-hand rule for a magnetic field into the page yields an induced current direction in the clockwise direction.

Report an Error

Example Question #4 : Electromagnetism

A capacitor and an inductor mH are connected in series to complete a simple circuit. The capacitor is initially charged to 9.9 nC.

Ps0_lccircuit

$C=820nF,\ L=530mH$

What is the value of the maximum current that passes through the circuit?

Possible Answers:

$8.4*10^{-7}A$

3.0A

$6.1 * 10^{-4}A$

$1.5 * 10^{-5 }A$

Correct answer:

$1.5 * 10^{-5 }A$

Explanation:

Since there are no dissipative circuit elements (such as a resistor) present in the circuit, the energy originally held by the electric field of the capacitor oscillates between electric energy and magnetic energy over time. As such, the maximum electric energy of the capacitor is equal in value to the maximum magnetic energy of the inductor.

$E_{C_{max}}=\frac{1}{2}\frac{(Q_{max})^2}{C}$

$E_{L_{max}}=\frac{1}{2}L(I_{max})^2$

$\frac{1}{2}\frac{(Q_{max})^{2}}{C}=\frac{1}{2}L(I_{max})^{2}$

$\frac{(9.9nC)^2}{820nF}=(530mH)(I_{max})^2$

$I_{max}=1.5*10^{-5}A$

Report an Error

Example Question #1 : Electromagnetism

A uniform magnetic field is created in a region in space, as represented by the grey arrows in the diagram below. Four circular loops A, B, C, and D are placed in the magnetic field. The planes of loops A and B are oriented perpendicularly to the direction of the field; the planes of loops C and D are oriented parallel to the field.

Ps0_fluxcomparison

Loops A and C are the same size, with radius . Loops B and D are the same size, with radius . Note that R>r .

Which of the statements below is true about the values of magnetic flux through the loops due to the magnetic field?

Possible Answers:

$\phi_{A}=\phi_{C}<\phi_{B}=\phi_{D}$

$\phi_{C}=\phi_{D}<\phi_{A}<\phi_{B}$

$\phi_{A}<\phi_{B}<\phi_{C}<\phi_{D}$

$\phi_{C}<\phi_{A}<\phi_{D}<\phi_{B}$

Correct answer:

$\phi_{C}=\phi_{D}<\phi_{A}<\phi_{B}$

Explanation:

Magnetic flux is a measure of the amount of magnetic field passing through a given area and is given by the equation:

$\phi_{m} =\int \overrightarrow{B}\cdot \overrightarrow{dA}$

The magnetic field is constant for this problem, and the areas are all circular loops. As such, the vector product integral simplifies to:

$\phi_{m}=BA\;cos\, \theta$

The angle theta is the angle between a vector normal to the surface of the area and the given magnetic field.

For loops C and D, theta is 90 degrees, so the magnetic flux for both of these loops is equal to zero.

$\phi_{m}=BA\;cos(90^\circ)=BA(0)=0$

For loops A and B, the angle theta is 0 degrees, so the magnetic flux for both of these loops is nonzero and proportional to the area of each loop. Therefore, the flux for loop B is larger than that for loop A because loop B has the larger radius.

R>r

A_B>A_A

$\phi_{m_B}>\phi_{m_A}$

Report an Error

Example Question #1 : Electric Circuits

A parallel plate capacitor has a capacitance of . If the plates are 1cm apart, what is the area of the plates?

$\epsilon_0=8.85* 10^{-12} \frac{F}{m}$

Possible Answers:

1.1*10^7m^2

$1.1*10^{11}m^2$

$1.1*10^{10}m^2$

1.1*10^9m^2

Correct answer:

1.1*10^9m^2

Explanation:

The relationship between capacitance, distance, and area is $C=\epsilon_0 \frac{A}{d}$ . We can rearrange this equation to solve for area.

$A=\frac{Cd}{\epsilon_0}$

Now, we can use the values given in teh question to solve.

$A=\frac{1F(1*10^{-2}m)}{8.85* 10^{-12} \frac{F}{m}}=1.1*10^9m^2$

Report an Error

Example Question #1 : Capacitors

Charge is distributed uniformly over the area of the two plates of a parallel plate capacitor, resulting in a surface area charge density of $\pm \sigma$ on the plates (the top plate is positive and the bottom is negative, as shown below). Each plate has area and are separated by distance . A material of dielectric constant $\kappa_{material}$ has been placed between the two plates.

Ps0_capacitor

Which of the following would not result in an increase in the measure of electric potential difference between the two plates?

Possible Answers:

Increase the value of the surface charge density, $\sigma$ , on each plate

Increase the area, , of the plates

Increase the distance, , between the plates

Replace the material between the two plates with one of a lower dielectric constant, $\kappa$

Correct answer:

Increase the area, , of the plates

Explanation:

The electric potential difference created between the plates of a parallel plate capactor is given by the equation:

$V = \frac{Q}{C}$

The charge can be calculated by using the equation:

$Q=\sigma A$

The value of the capacitance is related to the dimensions of the capacitor with the equation:

$C=\kappa \frac{\varepsilon _{o}A}{d}$

Combining these equations yields:

$V=\frac{\sigma A}{\frac{\kappa \epsilon_0A}{d}}=\frac{\sigma d}{\varepsilon_{o} \kappa }$

The area becomes inconsequential, while the potential is directly proportional to the surface charge density and the distance between the plates, and inversely proportional to the dielectric of the material between the plates. Changing the area does not cause any change in the potential difference measured between the plates, and changing any of the other variables would cause a resultant change in the potential difference.

Report an Error

Example Question #203 : Ap Physics C

You are hired to make a capacitor out of two parallel metal sheets. If someone wanted you to make a thin capacitor of $700\mu F$ out of those metal sheets, and the sheets needed to be 0.5mm apart, what area do the two metal plates need to be?

$\epsilon_0=8.85*10^{-12}\frac{C^2}{Nm^2}$

Possible Answers:

6.21*10^6m^2

$3.95*10^{-4}m^2$

2.77*10^3m^2

5.47*10^8m^2

3.95*10^4m^2

Correct answer:

3.95*10^4m^2

Explanation:

For parallel plate capacitors, the equation is $C=\frac{\epsilon_0A}{d}$ .

Solve for .

$A=\frac{dC}{\epsilon_0}$

Now we can plug in our given values.

$A=\frac{(0.5*10^{-3})(700*10^{-6})}{8.85*10^{-12}\frac{\text{C}^2}{\text{Nm}^2}}$

$A=3.95*10^4\ \text{m}^2$

Report an Error

Example Question #1 : Capacitors

The plates of a parallel plate capacitor are 3mm apart and 5m^2 in area. A potential difference of 1000V is applied across the capacitor. Find the capacitance.

$\epsilon_0=8.85*10^{-12} \frac{F}{m}$

Possible Answers:

$1.5*10^{-8}F$

$1.5*10^{-10}F$

$1.5*10^{-7}F$

$1.5*10^{-9}F$

Correct answer:

$1.5*10^{-8}F$

Explanation:

Capacitance is related to plate area and distance by the equation $C=\epsilon_0\frac{A}{d}$ .

Given the area and distance, we can solve for capacitance. The voltage, in this case, is irrelevant.

$C=8.85* 10^{-12} \frac{F}{m}(\frac{5 m^2}{3*10^{-3}m})=8.85* 10^{-12} \frac{F}{m}(1.67*10^{3}m)=1.5*10^{-8}F$

Report an Error

Example Question #2 : Capacitors

The plates of a parallel plate capacitor are 3mm apart and 5m^2 in area. A potential difference of 1000V is applied across the capacitor. Compute the charge on each plate.

$\epsilon_0=8.85* 10^{-12} \frac{F}{m}$

Possible Answers:

$1.5*10^{-5}C$

$1.5*10^{-6}C$

$1.5*10^{-8}C$

$1.5*10^{-7}C$

Correct answer:

$1.5*10^{-5}C$

Explanation:

Charge on a capacitor is given by the equation Q=CV . We know that the voltage is 1000V , but we need to determine the capacitance based off of the area and distance between the plates.

$C=\epsilon_0\frac{A}{d}$

$C=(8.85*10^{-12}\frac{F}{m})(\frac{5m^2}{3*10^{-3}m})$

$C=(8.85*10^{-12}\frac{F}{m})(1.67*10^{3}m)=1.5*10^{-8}F$

We can plug this value into the equation for charge.

$Q=CV=(1.5*10^{-8}F)(1000V)$

$Q=1.5*10^{-5}C$

Report an Error

Example Question #1 : Electric Circuits

The plates of a parallel plate capacitor are 3mm apart. A potential difference of 1000V is applied across the capacitor. Compute the magnitude of the generated electric field.

$\epsilon_0=8.85*10^{-12}\frac{F}{m}$

Possible Answers:

$3.33*10^6\frac{N}{C}$

$3.33*10^5\frac{N}{C}$

$3.33*10^4\frac{N}{C}$

$3.33*10^3\frac{N}{C}$

Correct answer:

$3.33*10^5\frac{N}{C}$

Explanation:

The electric field given by a capacitor is given by the formula $E=\frac{Q}{\epsilon_0 A}$ . We do not have these variables, so we will have to adjust the equation.

$Q=CV\rightarrow E=\frac{CV}{\epsilon_0A}$

The capacitance can be determined by the area of the plates and the distance between them.

$C=\epsilon_0\frac{A}{d}\rightarrow E=\frac{\epsilon_0\frac{A}{d}V}{\epsilon_0A}$

This equation simplifies to $E=\frac{V}{d}$ , allowing us to solve using the values given in the question.

$E=\frac{1000V}{3*10^{-3}m}=3.33*10^{5}\frac{N}{C}$

Report an Error

View Tutors

Nafis
Certified Tutor

University of Leipzig, Germany., Bachelor of Science, Physics. University of Pittsburgh-Pittsburgh Campus, Doctor of Philosop...

View Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

View Tutors

Cameo
Certified Tutor

University of Oklahoma Norman Campus, Bachelor of Science, Environmental Engineering.

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Spanish Tutors in Phoenix, LSAT Tutors in San Francisco-Bay Area, SSAT Tutors in Miami, Computer Science Tutors in Denver, Statistics Tutors in Dallas Fort Worth, MCAT Tutors in San Diego, Computer Science Tutors in Dallas Fort Worth, SSAT Tutors in Phoenix, Statistics Tutors in Washington DC, French Tutors in Washington DC

Popular Courses & Classes

GMAT Courses & Classes in New York City, GMAT Courses & Classes in Denver, SAT Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Philadelphia, SSAT Courses & Classes in Philadelphia, ACT Courses & Classes in Denver, SAT Courses & Classes in San Diego, SSAT Courses & Classes in Washington DC, SAT Courses & Classes in Los Angeles, MCAT Courses & Classes in Seattle

Popular Test Prep

MCAT Test Prep in Los Angeles, SAT Test Prep in Denver, SSAT Test Prep in Phoenix, LSAT Test Prep in Atlanta, MCAT Test Prep in Philadelphia, ISEE Test Prep in Washington DC, MCAT Test Prep in San Diego, GMAT Test Prep in Phoenix, MCAT Test Prep in Boston, ACT Test Prep in Philadelphia

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Physics C Electricity : Electricity and Magnetism Exam

Study concepts, example questions & explanations for AP Physics C Electricity

All AP Physics C Electricity Resources

Example Questions

Example Question #2 : Electromagnetism

Example Question #201 : Ap Physics C

Example Question #4 : Electromagnetism

Example Question #1 : Electromagnetism

Example Question #1 : Electric Circuits

Example Question #1 : Capacitors

Example Question #203 : Ap Physics C

Example Question #1 : Capacitors

Example Question #2 : Capacitors

Example Question #1 : Electric Circuits

All AP Physics C Electricity Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: