Call Now to Set Up Tutoring:

(888) 888-0446

AP Physics C Electricity : Calculating Electric Potential

Study concepts, example questions & explanations for AP Physics C Electricity

Create An Account

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #11 : Calculating Electric Potential

Ap physics c e m potential problems 2 6 16 1 2

Three equal point charges are placed at the vertices of an equilateral triangle of side length . Calculate the potential at the center of the triangle, labeled P.

Possible Answers:

$\frac{\sqrt{3}Q}{4\pi\epsilon_0a}$

$\frac{3\sqrt{3}Q}{2\pi\epsilon_0a}$

$\frac{3\sqrt{3}Q}{4\pi\epsilon_0a}$

$\frac{3\sqrt{3}Q}{8\pi\epsilon_0a}$

$\frac{\sqrt{3}Q}{2\pi\epsilon_0a}$

Correct answer:

$\frac{3\sqrt{3}Q}{4\pi\epsilon_0a}$

Explanation:

Draw a line from the center perpendicular to any side of the triangle. This line divides the side into two equal pieces of length $\frac{a}{2}$ . From the center, draw another line to one of the vertices at the end of this side. This produces a 30-60-90 triangle with longer leg $\frac{a}{2}$ , so the hypotenuse (the distance from the vertex to the center) is $\frac{a}{\sqrt{3}}$ . The potential at the center is due to three of these charges, so it must be

$V=3\times \left ( \frac{Q}{4\pi\epsilon_0\left(\frac{a}{\sqrt{3}} \right )} \right)$

$=\frac{3\sqrt{3}Q}{4\pi\epsilon_0a}$

Report an Error

Example Question #171 : Ap Physics C

Ap physics c e m potential problems 2 6 16 1 1

A uniformly charged hollow disk has inner radius R_1 and outer radius R_2 , and carries a total charge . Calculate the potential a distance from the center, on the axis of the disk.

Possible Answers:

$\frac{1}{8\pi\epsilon_0}\frac{Q}{R_2^2-R_1^2}\left(\sqrt{R_2^2+a^2}-\sqrt{R_1^2+a^2} \right )$

$\frac{1}{4\pi\epsilon_0}\frac{Q}{R_2^2}\left(\sqrt{R_2^2+a^2}-\sqrt{R_1^2+a^2} \right )$

$\frac{1}{4\pi\epsilon_0}\frac{Q}{R_2^2-R_1^2}\left(\sqrt{R_2^2+a^2}-\sqrt{R_1^2+a^2} \right )$

$\frac{1}{2\pi\epsilon_0}\frac{Q}{R_2^2-R_1^2}\left(\sqrt{R_2^2+a^2}-\sqrt{R_1^2+a^2} \right )$

$\frac{1}{2\pi\epsilon_0}\frac{Q}{R_2^2}\left(\sqrt{R_2^2+a^2}-\sqrt{R_1^2+a^2} \right )$

Correct answer:

$\frac{1}{2\pi\epsilon_0}\frac{Q}{R_2^2-R_1^2}\left(\sqrt{R_2^2+a^2}-\sqrt{R_1^2+a^2} \right )$

Explanation:

Use a polar coordinate system with surface charge density $\frac{Q}{\pi(R_2^2-R_1^2)}$ and area element $Rdrd\theta$ . The distance from the point of interest to a point a distance from the center is $\sqrt{r^2+a^2}$ , so the potential is

$V=\frac{1}{4\pi\epsilon_0}\int_{0}^{2\pi}\int_{R_1}^{R_2}\left(\frac{Q}{\pi(R_2^2-R_1^2)}\right )\frac{rdrd\theta}{\sqrt{r^2+a^2}}$

$=\frac{1}{4\pi\epsilon_0}(2\pi)\left(\frac{Q}{\pi(R_2^2-R_1^2)}\right )\int_{R_1}^{R_2}\frac{r}{\sqrt{r^2+a^2}}dr$

$=\frac{1}{2\pi\epsilon_0}\frac{Q}{R_2^2-R_1^2}\left[\sqrt{r^2+a^2} \right ]_{R_1}^{R_2}$

$=\frac{1}{2\pi\epsilon_0}\frac{Q}{R_2^2-R_1^2}\left(\sqrt{R_2^2+a^2} -\sqrt{R_1^2+a^2}\right )$

Report an Error

Example Question #21 : Electricity

Ap physics c e m potential problems 2 6 16 1

Two point charges and are separated by a distance . Calculate the potential at point P, a distance from charge in the direction perpendicular to the line connecting the two charges.

Possible Answers:

$\frac{-Q}{48\pi\epsilon_0a}$

$\frac{Q}{24\pi\epsilon_0a}$

$\frac{-Q}{30\pi\epsilon_0a}$

$\frac{Q}{60\pi\epsilon_0a}$

$\frac{11Q}{60\pi\epsilon_0a}$

Correct answer:

$\frac{Q}{60\pi\epsilon_0a}$

Explanation:

By the Pythagorean theorem, the distance from point P to charge is . Because point P is also from charge , it follows that the potential is

$V=\frac{1}{4\pi\epsilon_0}\left( \frac{2Q}{5a}-\frac{Q}{3a}\right )$

$=\frac{Q}{60\pi\epsilon_0a}$

Report an Error

Example Question #181 : Ap Physics C

Ap physics c e m potential problems 2 6 16 5

A nonuniformly charged ring of radius carries a linear charge density of $\lambda(\theta) = b\sin^2\theta$ . Calculate the potential at the center of the ring.

Possible Answers:

$\frac{b}{4\pi\epsilon_0}$

$\frac{b}{4\epsilon_0R}$

$\frac{b}{2\pi\epsilon_0}$

$\frac{b}{2\epsilon_0}$

$\frac{b}{4\epsilon_0}$

Correct answer:

$\frac{b}{4\epsilon_0}$

Explanation:

Use a polar coordinate system, the given linear charge density $\lambda(\theta)=b\sin^2\theta$ , and length element $Rd\theta$ . Since every point on the ring is the same distance from the center, we calculate the potential as

$V = \frac{1}{4\pi\epsilon_0}\int_{0}^{2\pi}b\sin^2\theta\left (\frac{1}{R} \right )R d\theta$

$=\frac{b}{4\pi\epsilon_0}\int_{0}^{2\pi}\sin^2\theta d\theta$

$=\frac{b}{4\pi\epsilon_0}\int_{0}^{2\pi}\frac{1-\cos2\theta}{2} d\theta$

$=\frac{b}{4\pi\epsilon_0}\left [\frac{\theta}{2}-\frac{\sin2\theta}{4} \right ]_{0}^{2\pi}$

$=\frac{b}{4\epsilon_0}$

Report an Error

Example Question #11 : Calculating Electric Potential

Ap physics c e m potential problems 2 6 16 6

In this model of a dipole, two charges and are separated by a distance as shown in the figure, where the charges lie on the x-axis at $+\frac{a}{2}$ and $-\frac{a}{2}$ respectively. Calculate the exact potential a distance from the origin at angle $\theta$ from the axis of the dipole.

Possible Answers:

$\frac{q}{4\pi\epsilon_0} \left ( \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 +2Ra \cos\theta}} - \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 - 2Ra\cos\theta}} \right )$

$\frac{q}{4\pi\epsilon_0} \left ( \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 +Ra \cos\theta}} - \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 - Ra\cos\theta}} \right )$

$\frac{q}{4\pi\epsilon_0} \left ( \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 -Ra \cos\theta}} - \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 + Ra\cos\theta}} \right )$

$\frac{q}{4\pi\epsilon_0} \left ( \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 -2Ra \cos\theta}} - \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 + 2Ra\cos\theta}} \right )$

$\frac{q}{4\pi\epsilon_0} \left ( \frac{1}{\sqrt{R^2 + a^2 -2Ra \cos\theta}} - \frac{1}{\sqrt{R^2 + a^2 + 2Ra\cos\theta}} \right )$

Correct answer:

$\frac{q}{4\pi\epsilon_0} \left ( \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 -Ra \cos\theta}} - \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 + Ra\cos\theta}} \right )$

Explanation:

By the law of cosines, the distance from the point to charge is

$\sqrt{R^2+\left(\frac{a}{2} \right )^2 - 2R\left(\frac{a}{2} \right )\cos\theta}$ .

The distance to charge can be found by using the law of cosines using the supplementary angle $\pi-\theta$ , for which $\cos(\pi-\theta) = \cos(\pi)\cos\theta - \sin(\pi)\sin\theta = -\cos\theta$ . Therefore the distance to is

$\sqrt{R^2+\left(\frac{a}{2} \right )^2 - 2R\left(\frac{a}{2} \right )(-\cos\theta)}$ .

Lastly, the exact potential is given by

$\frac{q}{4\pi\epsilon_0} \left ( \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 -Ra \cos\theta}} - \frac{1}{\sqrt{R^2 + \left (\frac{a}{2} \right)^2 + Ra\cos\theta}} \right )$ .

Remark: Far from the dipole (approximating $R\gg a$ ) gives the much simpler equation for the potential of an ideal electric dipole $\frac{qa\cos\theta}{4\pi\epsilon_0 R^2}$ .

Report an Error

Example Question #21 : Electricity And Magnetism Exam

Ap physics c e m potential problems 2 6 16 4

A uniformly charged hollow spherical shell of radius carries a total charge . Calculate the potential a distance (where a>R ) from the center of the sphere.

Possible Answers:

$\frac{Q}{8\pi\epsilon_0a}$

$\frac{Q}{4\pi\epsilon_0R}$

$\frac{Q}{4\pi\epsilon_0a^2}$

$\frac{Q}{4\pi\epsilon_0a}$

$\frac{Q}{8\pi\epsilon_0R}$

Correct answer:

$\frac{Q}{4\pi\epsilon_0a}$

Explanation:

Use a spherical coordinate system and place the point of interest a distance from the center on the z-axis. By the law of cosines, the distance from this point to any point on the sphere is $\sqrt{R^2 + a^2 -2Ra\cos\theta}$ . Using surface charge density $\frac{Q}{4\pi R^2}$ and area element $R^2\sin\theta d\theta d\phi$ , we evaluate the potential as:

$V =\frac{1}{4\pi\epsilon_0} \int_{0}^{2\pi}\int_{0}^{\pi}\left (\frac{Q}{4\pi R^2} \right )\frac{R^2\sin\theta d\theta d\phi}{\sqrt{R^2 + a^2-2Ra\cos\theta}}$

. $=\frac{1}{4\pi\epsilon_0} \left (2\pi \right )\left (\frac{Q}{4\pi} \right )\int_{0}^{\pi}\frac{\sin\theta d\theta d\phi}{\sqrt{R^2 + a^2-2Ra\cos\theta}}$

$=\frac{1}{4\pi\epsilon_0} \left (\frac{Q}{2} \right ) \left [\frac{1}{Ra}\sqrt{R^2 + a^2-2Ra\cos\theta} \right ]_{0}^{\pi}$

$=\frac{1}{4\pi\epsilon_0} \left (\frac{Q}{2} \right ) \frac{1}{Ra}\left (|R+a|-|R-a| \right )$

$=\frac{1}{4\pi\epsilon_0} \left (\frac{Q}{2} \right ) \frac{1}{Ra}(2R)$

$=\frac{Q}{4\pi\epsilon_0a}$

Remarkably, this is the same potential that would exist a distance from a point charge.

Report an Error

Example Question #31 : Electricity

Ap physics c e m potential problems 2 6 16 3

A thin bar of length L lies in the xy plane and carries linear charge density $\lambda(x) = bx$ , where ranges from 0 to . Calculate the potential at the point (0, a) on the y-axis.

Possible Answers:

$\frac{b}{4\pi\epsilon_0}\sqrt{L^2+a^2}$

$\frac{b}{2\pi\epsilon_0}\left (\sqrt{L^2+a^2}-a \right )$

$\frac{b}{8\pi\epsilon_0}\sqrt{L^2+a^2}$

$\frac{b}{8\pi\epsilon_0}\left (\sqrt{L^2+a^2}-a \right )$

$\frac{b}{4\pi\epsilon_0}\left (\sqrt{L^2+a^2}-a \right )$

Correct answer:

$\frac{b}{4\pi\epsilon_0}\left (\sqrt{L^2+a^2}-a \right )$

Explanation:

Use the linear charge density $\lambda(x)=bx$ and length element , where each point is $\sqrt{x^2+a^2}$ from the point (0,a) . The potential is therefore

$V=\frac{1}{4\pi\epsilon_0}\int_{0}^{L}\frac{bx}{\sqrt{x^2+a^2}}dx$

$= \frac{b}{4\pi\epsilon_0}\left [ \sqrt{x^2+a^2}\right ]_{0}^{L}$

$=\frac{b}{4\pi\epsilon_0}\left (\sqrt{L^2+a^2}-a \right )$

Report an Error

Example Question #32 : Electricity

Ap physics c e m potential problems 2 6 16 2

A uniformly charged ring of radius carries a total charge . Calculate the potential a distance from the center, on the axis of the ring.

Possible Answers:

$\frac{Q}{4\pi\epsilon_0(R^2 + a^2)}$

$\frac{Qa}{4\pi\epsilon_0(R^2+a^2)^{\frac{3}{2}}}$

$\frac{Qa}{4\pi\epsilon_0(R^2 + a^2)}$

$\frac{Q}{4\pi\epsilon_0\sqrt{R^2 + a^2}}$

$\frac{Q}{4\pi\epsilon_0R\sqrt{R^2 + a^2}}$

Correct answer:

$\frac{Q}{4\pi\epsilon_0\sqrt{R^2 + a^2}}$

Explanation:

Use the linear charge density $\frac{Q}{2\pi R}$ and length element $Rd\theta$ . The distance from each point on the ring to the point on the axis is $\sqrt{R^2+a^2}$ . Lastly, integrate over $\theta$ from to $2\pi$ to obtain

$V=\frac{1}{4\pi\epsilon_0}\int_{0}^{2\pi}\left (\frac{Q}{2\pi R} \right )\frac{1}{\sqrt{R^2+a^2}}Rd\theta$

$=\frac{Q}{4\pi\epsilon_0\sqrt{R^2+a^2}}$

Report an Error

Example Question #12 : Calculating Electric Potential

Ap physics c e m potential problems 2 6 16

A uniformly charged square frame of side length carries a total charge . Calculate the potential at the center of the square.

You may wish to use the integral:

$\int{\frac{dx}{\sqrt{x^2+b^2}}} = \ln {(\sqrt{x^2+b^2} + x)} + C$

Possible Answers:

$\frac{Q}{2\pi\epsilon_{0}a}\ln{(1+\sqrt{2})}$

$\frac{Q}{4\pi\epsilon_{0}a}\ln{(1+\sqrt{2})}$

$\frac{Q}{\pi\epsilon_{0}a}\ln{(1+\sqrt{2})}$

$\frac{Q}{8\pi\epsilon_{0}a}\ln{(1+\sqrt{2})}$

$\frac{2Q}{\pi\epsilon_{0}a}\ln{(1+\sqrt{2})}$

Correct answer:

$\frac{Q}{2\pi\epsilon_{0}a}\ln{(1+\sqrt{2})}$

Explanation:

Calculate the potential due to one side of the bar, and then multiply this by to get the total potential from all four sides. Orient the bar along the x-axis such that its endpoints are at $\pm\frac{a}{2}$ , and use the linear charge density $\frac{Q}{4a}$ . The potential is therefore

$V=4\times\left (\frac{1}{4\pi\epsilon_{0}}\int_{-a/2}^{a/2} \left (\frac{Q}{4a} \right )\frac{1}{\sqrt{x^2+\frac{a^2}{4}}}dx\right )$

$=\frac{Q}{4\pi\epsilon_{0}a}\int_{-a/2}^{a/2} \frac{1}{\sqrt{x^2+\frac{a^2}{4}}}dx$

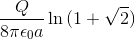
$=\frac{Q}{4\pi\epsilon_{0}a}\left[ \ln\left (\sqrt{x^2+\frac{a^2}{4}} +x\right )\right]_{-a/2}^{a/2}$

$=\frac{Q}{4\pi\epsilon_0a}\ln\left (\frac{\sqrt{2}+1}{\sqrt{2}-1} \right )$

$=\frac{Q}{2\pi\epsilon_0a}\ln(1+\sqrt{2})$

Report an Error

View Tutors

Nafis
Certified Tutor

University of Leipzig, Germany., Bachelor of Science, Physics. University of Pittsburgh-Pittsburgh Campus, Doctor of Philosop...

View Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

View Tutors

Cameo
Certified Tutor

University of Oklahoma Norman Campus, Bachelor of Science, Environmental Engineering.

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

English Tutors in San Diego, Physics Tutors in Seattle, English Tutors in Miami, Computer Science Tutors in Washington DC, ACT Tutors in Denver, Calculus Tutors in Phoenix, Physics Tutors in Boston, Spanish Tutors in Boston, Biology Tutors in Phoenix, GRE Tutors in San Francisco-Bay Area

Popular Courses & Classes

ISEE Courses & Classes in Boston, SSAT Courses & Classes in Phoenix, SAT Courses & Classes in Los Angeles, SAT Courses & Classes in New York City, GRE Courses & Classes in San Francisco-Bay Area, SSAT Courses & Classes in Denver, GRE Courses & Classes in Washington DC, LSAT Courses & Classes in Houston, Spanish Courses & Classes in San Diego, Spanish Courses & Classes in San Francisco-Bay Area

Popular Test Prep

SSAT Test Prep in Boston, SAT Test Prep in San Diego, LSAT Test Prep in San Diego, ISEE Test Prep in Philadelphia, MCAT Test Prep in San Francisco-Bay Area, LSAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in Chicago, LSAT Test Prep in Washington DC, SSAT Test Prep in Seattle, ISEE Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Physics C Electricity : Calculating Electric Potential

Study concepts, example questions & explanations for AP Physics C Electricity

All AP Physics C Electricity Resources

Example Questions

Example Question #11 : Calculating Electric Potential

Example Question #171 : Ap Physics C

Example Question #21 : Electricity

Example Question #181 : Ap Physics C

Example Question #11 : Calculating Electric Potential

Example Question #21 : Electricity And Magnetism Exam

Example Question #31 : Electricity

Example Question #32 : Electricity

Example Question #12 : Calculating Electric Potential

All AP Physics C Electricity Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: