All AP Physics 1 Resources
Example Questions
Example Question #211 : Specific Forces
A scuba diver and his equipment have a total mass of 


None of the other answers
If the diver stays at a constant depth, we know that all the forces on him must cancel out:
We can write out all of the forces on the diver, using force of the flippers to denote the vertical force he exerts to stay at constant depth:
The force of gravity and bouyancy counteract each other, so they will have opposite signs. If we assume that the force of flippers will be down, we can write:
The bouyancy force is simply the weight of the water displaced by the diver. Plugging in expressions for the latter two forces:
We have values for all of these, allowing us to solve:
Example Question #212 : Forces
A spring is attached to the top of a box. The constant of the spring is 


Since the spring is initially in equilibrium, we can write:
There are three forces in play: spring force, gravitational force, and the additional force resulting from the acceleration of the box. If we say that any forces pointing downward are positive, we can write:
The force resulting from the additional acceleration must be subtracted since all forces cancel out and both of the other two forces are postive. This means that the resulting force from acceleration is pointing upward; Thus, the box is accelerating downward (think about riding in an elevator).
Substituting expressions for each force, we get:
Rearrange to solve for the acceleration of the box:
Example Question #213 : Forces
Consider the following scenario:
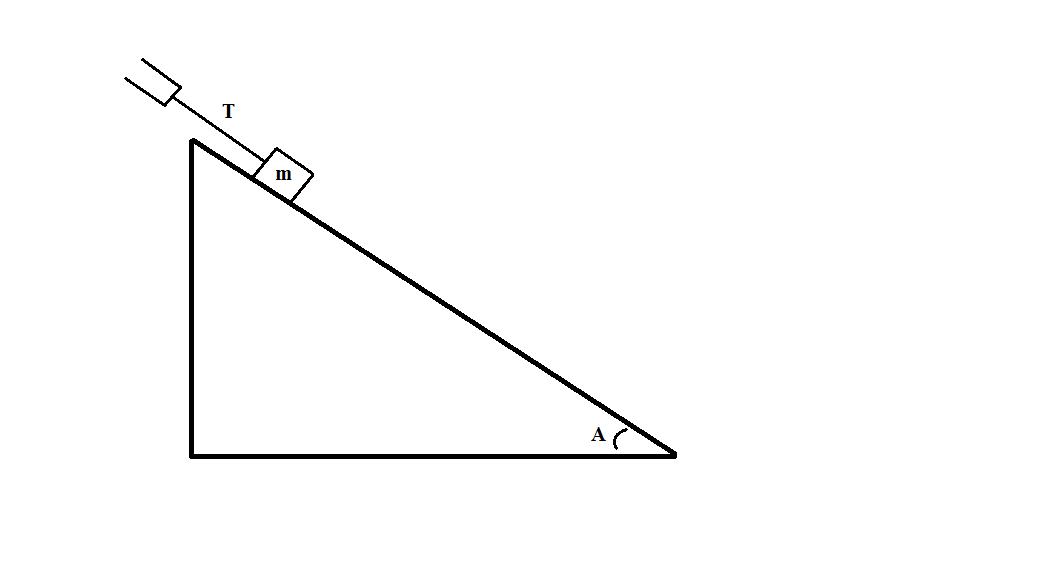
If the force of tension is 


There are three relevant forces in play in this scenario: friction, tension, and gravity.
We can use Newton's second law to create an expression for the net force:
Substituting expressions in for the forces, we get:
Rearrange to solve for the mass:
Use our given values to solve:
Example Question #821 : Ap Physics 1
An astronaut is in orbit around the earth. The feeling of weightlessness that he experiences is due to the absence of which force?
Normal force
Frictional force
Gravitational force
Buoyant force
Normal force
The correct answer is normal force. Normal force will occur when the astronaut is standing on a surface, which will push back on him. Gravity is present since the astronaut is in orbit (gravity provides the centripetal force). The feeling of weightlessness he experiences is due to the absence of a surface to push back on him/her.
Example Question #215 : Forces
Consider a 


To solve this problem, we need to look at all of the forces acting on the sphere, this includes the force of gravity, the normal force provided by the scale, and the buoyancy force. If the object is in equilibrium, the net force acting on the sphere must be zero.
Example Question #821 : Ap Physics 1
Suppose that a student pushes his physics textbook across the table with his hand. In doing so, what type of force is acting on the book?
Strong force
Weak force
Electromagnetic force
Gravitational force
Electromagnetic force
If a person were to use their hand to push a book across the table, the type of force that their hand is applying to the book is classified as an electromagnetic force. Even though this may not seem intuitive, the reason is that the electrostatic repulsion of the atoms in the person's hand repel the atoms in the book. Due to this electrostatic repulsion between the atoms in the person's hand and the atoms in the book, the force is electromagnetic.
Gravitational forces occur due to gravity, that is, from an attraction between any two objects with mass. The strong and weak forces are both nuclear forces that act only across very, very short distances. Generally, these forces are only significant at the sub-atomic level in the atom's nucleus, and thus do not have a role at greater distances (such as between the person's hand and the book).
Example Question #217 : Forces
Mass of Mars:
Universal gravitation constant:
Radius of Mars:
A buggy on the surface of Mars locks up it's break and slides on Martian ice. If the buggy was traveling at 

None of these
The normal force will be equal to the magnitude of the force of gravity pushing the buggy into the ground.
Using definition of frictional force and work equation:
Combining equations:
Canceling out the mass of the buggy and plugging in values:
Solving for
Example Question #213 : Forces
An adventurous teenager has decided to pick up skydiving. If the teen has a mass of 



When a diver reaches terminal velocity, we know that the net force on the diver is equal to 0. There are two forces acting on the diver, gravity and drag, so we can say that:
Plugging in expressions, we get:
Rearranging for density, we get:
We have all of these values, so we can plug and chug:
Example Question #2 : Other Forces
A 


This question tests your understanding of the concept of centripetal force. Centripetal force is a force that maintains a body's circular travel along a curved surface and points inward towards the center of the curve from each point that the body travels along. Centripetal force is calculated as follows:
Therefore the centripetal force on the car is 
Example Question #222 : Forces
Nicola is attempting to create a single tiered mobile as shown. She has a wooden dowel (




If Nicola places ornament 



Note: figure not drawn to scale.





Let's use the midpoint as our point of reference. With that said, 



Our main equation is this:
Where 


Now let's plug in our numbers, remember about our negative/positive sides!
This should give us
Now, because this number is negative, we know it's to the left of our midpoint (if you chose to set up your -/+ sides opposite of how we did it, your answer will be positive). Regardless of the outcome, this measurement is meant to be taken from object A to the midpoint. Well, do any of our answers have either (+) or (-) 

One side is

Think about it logically too (use your pencil). The heavier end of a pencil usually has a bulky eraser on it (just like the bulkier object on the left). Try holding the pencil at the tip's end, and then gradually try balancing it in the same manner as you move closer to the eraser.
ALSO NOTE: the question states the weight of the dowel as well. But look we didn't even need it. Sometimes the AP exam will give you some aspects in the question to distract you.
All AP Physics 1 Resources




















































































