All AP Physics 1 Resources
Example Questions
Example Question #501 : Newtonian Mechanics
A passenger jet is beginning its decent to its final destination. If it is at a height of 3,000m and traveling at a rate of 
More information is needed to solve
We know that the plane needs to drop 3,000 meters in 10 minutes. Therefore, we can calculate the vertical velocity that the plane needs to be traveling at:
Knowing the plane's velocity, we can caluclate what angle at which it needs to decend to achieve this vertical velocity:
Rearranging for theta:
Plugging in our values:
Example Question #1 : Fundamentals Of Displacement, Velocity, And Acceleration
Upon ignition, a bottle rocket accelerates at a rate of 

Neglect air resistance and assume
We can use our kinematics equations to determine the height of the rocket the moment it loses thrust:
If we clarify the initial state being the moment the rocket loses thrust and the final state being the point of its maximum height, we can use the expression for conservation of energy to calculate the final height:
Removing final kinetic energy (which will be zero) and substituting in our expressions for each variable:
Cancel out mass and rearrange for final height:
From the problem statement, we can determine the initial velocity:
Now that we have values for all of our variables, we can solve:
Example Question #2 : Fundamentals Of Displacement, Velocity, And Acceleration
A cannon is packed with gunpowder and a ball of mass 10kg. The cannon is angled at 30 degrees. When fired, the gunpowder releases 500J of energy, which is all transferred to the cannon ball. Neglecting air resistance and friction within the cannon, how far does the ball travel before hitting the ground?
Assume
There are two ways to solve this problem. The first and much easier way is to use the range equation. The second is using your kinematics equations.
Method 1: Range Equation
The range equation is the following:
We know everything ecvept for the initial velocity. However, we can calculate it knowing that the cannon transfers 500 J of energy to the ball. Therefore:
Rearranging for velocity:
Now we can plug everything into the range equation:
Method 2: Kinematics Equations
As in the first method, we can calculate the initial velocity of the ball:
Rearranging for velocity:
We can then split this into it's components:
We can use the y-component to calculate how long the ball is in the air. We can do one of two things:
- Calculate how long the ball takes to reach it's peak and then multiply by 2
- Calculate how lon gthe ball takes to get a velocity of -5
We'll go with method 2:
Rearranging for t:
Then we can multiply this time by the horizontal velocity (which stays constant because we are neglecting air resistance).
Example Question #3 : Newtonian Mechanics
A box is placed on a 30o frictionless incline. What is the acceleration of the box as it slides down the incline?
To find the acceleration of the box traveling down the incline, the mass is not needed. Using the incline of the plane as the x-direction, we can see that there is no movement in the y-direction; therefore, we can use Newton's second, F = ma, in the x-direction.
There is only one force in the x-direction (gravity), however gravity is not just equal to “mg” in this case. Since the box is on an incline, the gravitational force will be equal to mgsin(30o). Substituting force into F =ma we find that mgsin(30o) = ma. We can now cancel out masses and solve for acceleration.
Example Question #272 : Linear Motion And Momentum
Consider the following system:
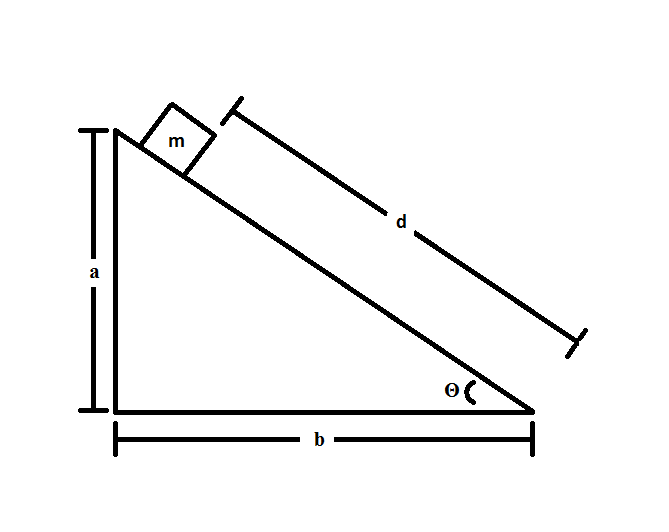
If 

There are two forces in play in this scenario: friction and gravity. We can use Newton's second law to develop an expression for the acceleration of the block:
Substituting in expressions for each force, we get:
Canceling out mass and rearranging for acceleration, we get:
We know all of our values, allowing us to solve for the acceleration:
Example Question #512 : Newtonian Mechanics
A young boy is aiming a slingshot straight upward. If the sling shot applies 

We can use the equation for conservation of energy to solve this problem:
If we assume the initial state is when the slingshot is fully loaded and the final state is at the ball's maxmium height, we can completely eliminate kinetic energy to get:
Substituting in our value for initital potential energy and an expression for final potential energy, we get:
Rearranging for final height we get:
Example Question #513 : Newtonian Mechanics
A sports car can accelerate from 

The basic kinematics equation for motion under constant acceleration is:
We do not have the acceleration, but we can find it:
Apply this to the first equation and solve.
Example Question #1 : Fundamentals Of Displacement, Velocity, And Acceleration
A walker is walking at a constant rate of 

The equation for speed is:
The runner has gone around the track 1.5 times, thus his total distance traveled is:
We can find the elapsed time from the walker: Rearrange the speed equation to find time:
Now we have all the information we need to find the runner's speed:
Example Question #515 : Newtonian Mechanics
An object is moving to the right and is speeding up. Which of the following is false?
The magnitude of the object's velocity increases
The object experiences a positive net force
The object's acceleration is positive
The object's velocity is positive
The object experiences a net force of 0N
The object experiences a net force of 0N
If an object is moving to the right, then its displacement is positive. If its displacement is positive then its velocity must be positive as well. The object is speeding up. This implies that the magnitude of its velocity is increasing. If this is true we have that the velocity of the object, 



So we have that the object's acceleration is positive. If the object's acceleration is positive, then there must be a positive net force causing the acceleration, due to Newton's first law: 
Example Question #2 : Fundamentals Of Displacement, Velocity, And Acceleration
Which of the following statements is false regarding the relationship between an object's velocity and acceleration?
If both the object's acceleration and initial velocity vectors are positive, then the object will speed up
If both the object's acceleration and initial velocity vectors are negative, then the object will slow down
If an object experiences a nonzero acceleration, then its velocity will change
If the object's acceleration is positive and its initial-velocity is negative, then the object will slow down
If an object experiences constant velocity, then its acceleration is 0
If both the object's acceleration and initial velocity vectors are negative, then the object will slow down
By definition, acceleration is the rate of change of velocity with respect to time. The average acceleration of an object is given by:
Therefore, if velocity is constant it must be the case that acceleration is 0, since the initial and final velocities of the object are equal. Similarly, if acceleration is non-zero, then it must be the case that the initial and final velocities of the object are different (so velocity is changing). Now, when an object's acceleration and initial-velocity have the same sign (i.e. the same direction) then the object will speed up. In other words, the absolute value of its velocity (the magnitude) increases. This is easy to see if we solve for the final velocity in our equation:
If both 




Example:
Example:
Remember that the positive and negative signs indicate the direction of the vectors. So when it comes to slowing down or speeding up, we want to look at the magnitude of the velocity. Therefore, the only statement that is false is the one that says that if an object has both negative initial velocity and acceleration it will slow down. The object will speed up and continue to move to the left.
All AP Physics 1 Resources