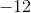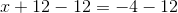All Algebra 1 Resources
Example Questions
Example Question #198 : Systems Of Equations
Find the solution set for this system of equations:
None of these
To solve this system of equations, multiply each equation by an integer that would allow us to cancel one variable. We then substitute that value back into either original equation to find the other variable. In this case we will cancel the x variable by multiplying each equation by 3 and 4 respectively.
1)
2)
Multiply equation 1 by 3 and equation 2 by 4:
Add the new equations:
The result is
Solve for y:
Now substitute this value back into equation 1:
Example Question #781 : Psat Mathematics
Give the solution to the system of equations below.
No solution
Solve the second equation for 
Substitute for 

Now, substitute for 

Now we can write the solution in the notation 

Example Question #203 : Grade 8
Solve this system of equations for 
None of the other choices are correct.
Multiply the bottom equation by 
Divide both sides by
Example Question #11 : Solve Systems Of Two Linear Equations: Ccss.Math.Content.8.Ee.C.8b
Solve the following system of equations:
no solution
infinitely many solutions
When we add the two equations, the 
Solving for 
We can then substitute our value for 

Example Question #12 : Solve Systems Of Two Linear Equations: Ccss.Math.Content.8.Ee.C.8b
Solve the following system of equations.
We are given
We can solve this by using the substitution method. Notice that you can plug 
Add 
Add 9 to both sides
Divide both sides by 5
So 

So the solution is
Example Question #76 : New Sat Math Calculator
Adult tickets to the zoo sell for 



Let 

The amount of money raised from adult tickets is 


To find the number of adult tickets sold, solve for 

Example Question #4 : Translating Words To Linear Equations
Solve the following story problem:
Jack and Aaron go to the sporting goods store. Jack buys a glove for 







Let's call "
Let's call the amount of money Jack starts with "
Let's call the amount of money Aaron starts with "
We know Jack buys a glove for 



simplifying, 
We know Aaron buys 



The last important piece of information from the problem is Aaron starts with 
From before we know:
Plugging in:
so Aaron started with
Finally we plug 
Thus one jersey costs
Example Question #51 : How To Find The Solution For A System Of Equations
Solve for 
Use the elimination process to determine the value of 
Subtract the second equation with the first equation. This will eliminate the x-variable in order to find the y-variable.
The equation becomes:
Use this value and substitute into either the first or second equation and solve for the x-variable.
Let's select the first equation and solve for 
Add six on both sides.
The result will be the same if we substitute 
The answer is:
Example Question #321 : Equations / Inequalities
Solve only for 
In order to solve for 
The first equation becomes:
The second equation remains the same:
Subtract the second equation with the first equation:
The result is:
Divide by two on both sides.
Simplify both sides.
The answer is:
Example Question #53 : How To Find The Solution For A System Of Equations
Solve for 
In order to solve for the 
Subtract the first equation with the second equation in order to eliminate the 

After subtracting equation one with equation two:
Use this value and either equation to solve for 
Subtract twelve from both sides.
Simplify both sides.
The answer is:
Certified Tutor
All Algebra 1 Resources