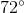We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

ACT Math : Quadrilaterals

Study concepts, example questions & explanations for ACT Math

Create An Account

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #51 : Kites

A kite has a side length of $15\textup{ inches}$ and another side length of $30\textup{ inches}$ . Find the perimeter of the kite.

Possible Answers:

$45\textup{ inches}$

$7.5\textup{ feet}$

$87\textup{ inches}$

$90 \textup{ cm}$

$12\textup{ feet}$

Correct answer:

$7.5\textup{ feet}$

Explanation:

By definition a kite must have two sets of equivalent sides. Since we know that this kite has a side length of and another side with a length of , each of these two sides must have one equivalent side.

The perimeter of this kite can be found by applying the formula:

p=2(a+b)

p=2(15+30)

$p=2(45)=90\textup{ inches}$

Note, though, that $90\textup{ inches}$ does not appear as an answer choice. Thus, convert $90\textup{ inches}$ into $\textup{feet}$ by: $90\div12=7.5\textup{ feet}$

Report an Error

Example Question #51 : Quadrilaterals

A kite has a side length of $88\textup{mm}$ and another side length of $36\textup{mm}$ . Find the perimeter of the kite.

Possible Answers:

$209\textup{cm}$

$264\textup{mm}$

$176\textup{mm}$

$248\textup{mm}$

$209\textup{mm}$

Correct answer:

$248\textup{mm}$

Explanation:

a kite must have two sets of equivalent sides. Since we know that this kite has a side length of $88\textup{mm}$ and another side with a length of $36\textup{mm}$ , each of these two sides must have one equivalent side.

The perimeter of this kite can be found by applying the formula:

p=2(a+b)

p=2(88+36)

$p=2(124)=248\textup{mm}$

Note: the correct solution can also be found by:

p=88+88+36+36=176+72=248

Report an Error

Example Question #52 : Quadrilaterals

A kite has a side length of $12\textup{cm}$ and another side length of $16\textup{cm}$ . Find the perimeter of the kite.

Possible Answers:

$24\textup{cm}$

$96\textup{in}$

$60\textup{cm}$

$56\textup{cm}$

$90\textup{cm}$

Correct answer:

$56\textup{cm}$

Explanation:

A kite must have two sets of equivalent sides. Since we know that this kite has a side length of and another side length of , each of these two sides must have one equivalent side.

The perimeter of this kite can be found by applying the formula:

p=2(a+b)

p=2(12+16)

$p=2(28)=56\textup{cm}$

Report an Error

Example Question #31 : Kites

Find the area of a kite with the diagonal lengths of and .

Possible Answers:

4a+4b

2ab

2a+2b

4ab

Correct answer:

2ab

Explanation:

Write the formula to find the area of a kite. Substitute the diagonals and solve.

$A=\frac{d1 \cdot d2}{2} = \frac{2a \cdot 2b}{2} =2ab$

Report an Error

Example Question #1 : How To Find The Area Of A Kite

Find the area of a kite with diagonal lengths of a+b and 2a-2b .

Possible Answers:

a^2-b^2

2a^2+2b^2

2a^2-2b^2

a^2-ab-b^2

a^2+b^2

Correct answer:

a^2-b^2

Explanation:

Write the formula for the area of a kite.

$A= \frac{d1\cdot d2}{2}$

Plug in the given diagonals.

$A= \frac{(a+b)\cdot (2a-2b)}{2}$

Pull out a common factor of two in 2a-2b and simplify.

$A= \frac{(a+b)\cdot2(a-b)}{2}$

A=(a+b)(a-b)

Use the FOIL method to simplify.

A=(a+b)(a-b)= (a)(a)+(a)(-b)+(b)(a)+(b)(-b)

A= a^2-b^2

Report an Error

Example Question #31 : Kites

Find the area of a kite if one diagonal is $2\:cm$ long, and the other diagonal is $10\:cm$ long.

Possible Answers:

$20\:cm^2$

$10\sqrt2\:cm^2$

$3\sqrt{10}$ cm^2

$10\sqrt3\:cm^2$

$10\:cm^2$

Correct answer:

$10\:cm^2$

Explanation:

The formula for the area of a kite is

$A=\frac{d_1\cdot d_2}{2}$

Plug in the values for each of the diagonals and solve.

$A = \frac{2\cdot 10}{2}=\frac{20}{2}=10\:cm^2$

Report an Error

Example Question #51 : Quadrilaterals

If a trapezoid has a height of 14, base lengths of 10 and 12, and side lengths of 13, what is its perimeter?

Possible Answers:

Correct answer:

Explanation:

Use the formula for perimeter of a trapezoid:

$P=b_{1}+b_{2}+s+s$

Where is the perimeter, b_1 and b_2 are the lengths of the bases, and is the length of the side.

In this case:

P=10+12+13+13=48

Report an Error

Example Question #1 : How To Find An Angle In A Trapezoid

Find the measure of angle in the isosceles trapezoid pictured below.

Screen_shot_2013-03-18_at_3.32.40_pm

Possible Answers:

$108^{\circ}$

$18^{\circ}$

$72^{\circ}$

$36^{\circ}$

$118^{\circ}$

Correct answer:

$108^{\circ}$

Explanation:

The sum of the angles in any quadrilateral is 360°, and the properties of an isosceles trapezoid dictate that the sets of angles adjoined by parallel lines (in this case, the bottom set and top set of angles) are equal. Subtracting 2(72°) from 360° gives the sum of the two top angles, and dividing the resulting 216° by 2 yields the measurement of x, which is 108°.

Report an Error

Example Question #2 : How To Find An Angle In A Trapezoid

Trapezoid ABCD is an isosceles trapezoid with angle $\angle C = 35^{\circ}$ . If $\angle A$ and $\angle B$ are paired, what is the measure of $\angle A$ ?

Possible Answers:

$55^{\circ}$

$215^{\circ}$

$30^{\circ}$

$145^{\circ}$

$35^{\circ}$

Correct answer:

$145^{\circ}$

Explanation:

As a rule, adjacent (non-paired) angles in a trapezoid are supplementary. Thus, we know that if $\angle C = 35^{\circ}$ , then $\angle B = 145^{\circ}$ . Since we are told that $\angle A$ and $\angle B$ are paired and trapezoid ABCD is isosceles, $\angle A$ must also equal $145^{\circ}$ .

Report an Error

Example Question #2 : How To Find An Angle In A Trapezoid

In the isosceles trapezoid above,

$\overline{AB}\left | \right | \overline{DC}$ . $\angle BDC = 50^{\circ}$ and $\angle BCA = 15^{\circ}$ .

In degrees, what is the measure of $\angle DAC$ ?

Possible Answers:

$75^\circ$

$80^\circ$

$65^\circ$

$115^\circ$

$35^\circ$

Correct answer:

$65^\circ$

Explanation:

To find the measure of angle DAC, we must know that the interior angles of all triangles sum up to 180 degrees. Also, as this is an isosceles trapezoid, $\angle ADC$ and $\angle BCD$ are equal to each other. The two diagonals within the trapezoid bisect angles $\angle ADC$ and $\angle BCD$ at the same angle.

Thus, $\angle ACD$ must also be equal to 50 degrees.

Thus, $\angle ADC = 50 + 15 = 65$ .

Now that we know two angles out of the three in the triangle on the left, we can subtract them from 180 degrees to find $\angle DAC$ :

180-65-50=65

Report an Error

View ACT Math Tutors

Dejuan
Certified Tutor

University of Illinois at Urbana-Champaign, Bachelor of Science, Electrical Engineering.

View ACT Math Tutors

Leonard
Certified Tutor

Florida State University, Bachelor of Science, Environmental Science.

View ACT Math Tutors

Yucheng
Certified Tutor

The University of Texas at Austin, Bachelor of Science, Mechanical Engineering.

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

ACT Math Tutors in Top Cities:

Atlanta ACT Math Tutors, Austin ACT Math Tutors, Boston ACT Math Tutors, Chicago ACT Math Tutors, Dallas Fort Worth ACT Math Tutors, Denver ACT Math Tutors, Houston ACT Math Tutors, Kansas City ACT Math Tutors, Los Angeles ACT Math Tutors, Miami ACT Math Tutors, New York City ACT Math Tutors, Philadelphia ACT Math Tutors, Phoenix ACT Math Tutors, San Diego ACT Math Tutors, San Francisco-Bay Area ACT Math Tutors, Seattle ACT Math Tutors, St. Louis ACT Math Tutors, Tucson ACT Math Tutors, Washington DC ACT Math Tutors

Popular Courses & Classes

LSAT Courses & Classes in Houston, LSAT Courses & Classes in Los Angeles, SAT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in San Francisco-Bay Area, ISEE Courses & Classes in Denver, GMAT Courses & Classes in Atlanta, GMAT Courses & Classes in Houston, GRE Courses & Classes in Boston, GRE Courses & Classes in Houston, ISEE Courses & Classes in Dallas Fort Worth

Popular Test Prep

SAT Test Prep in San Francisco-Bay Area, GMAT Test Prep in Seattle, GMAT Test Prep in Houston, MCAT Test Prep in Chicago, MCAT Test Prep in Miami, GMAT Test Prep in Denver, LSAT Test Prep in Houston, MCAT Test Prep in Denver, GRE Test Prep in San Diego, LSAT Test Prep in San Diego

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ACT Math : Quadrilaterals

Study concepts, example questions & explanations for ACT Math

All ACT Math Resources

Example Questions

Example Question #51 : Kites

Example Question #51 : Quadrilaterals

Example Question #52 : Quadrilaterals

Example Question #31 : Kites

Example Question #1 : How To Find The Area Of A Kite

Example Question #31 : Kites

Example Question #51 : Quadrilaterals

Example Question #1 : How To Find An Angle In A Trapezoid

Example Question #2 : How To Find An Angle In A Trapezoid

Example Question #2 : How To Find An Angle In A Trapezoid

All ACT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: