We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

ACT Math : Plane Geometry

Study concepts, example questions & explanations for ACT Math

Create An Account

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #4 : How To Find The Length Of The Diameter

A circle has an area of $39\pi$ . What is the diameter of the circle?

Possible Answers:

$2\sqrt{39\pi }$

$\sqrt{39}$

$2\sqrt{39}\pi$

$2\sqrt{39}$

$\sqrt{39\pi }$

Correct answer:

$2\sqrt{39}$

Explanation:

The equation for the area of a circle is $\pi r^2$ , which in this case equals $39\pi$ . Therefore, r^2 = 39. The only thing squared that equals an integer (which is not a perfect root) is that number under a square root. Therefore, $r=\sqrt{39}$ . Since diameter is twice the radius, $d=2\sqrt{39}.$

Report an Error

Example Question #1 : Diameter

Find the diameter given the radius is .

Possible Answers:

$\frac{3}{2}$

Correct answer:

Explanation:

Diameter is simply twice the radius. Therefore, 2*3=6 .

Report an Error

Example Question #2 : Diameter

Find the length of the diameter of a circle given the area is $4\pi$ .

Possible Answers:

Correct answer:

Explanation:

To solve, simply use the formula for the area of a circle, solve for r, and multiply by 2 to get the diameter. Thus,

$A=\pi{r^2}\Rightarrow r=\sqrt{\frac{A}{\pi}}$

$r=\sqrt{\frac{4\pi}{\pi}}=\sqrt{4}=2$

d=2r=2*2=4

Report an Error

Example Question #6 : How To Find The Length Of The Diameter

In a group of students, it was decided that a pizza would be divided according to its crust size. Every student wanted 3 inches of crust (measured from the outermost point of the pizza). If the pizza in question had a diameter of 14 inches, what percentage of the pizza was wasted by this manner of cutting the pizza? Round to the nearest hundredth.

Possible Answers:

$66.08\%$

$4.51\%$

$14.66\%$

$5.71\%$

$2.81\%$

Correct answer:

$4.51\%$

Explanation:

What we are looking at is a way of dividing the pizza according to arc lengths of the crust. Thus, we need to know the total circumference first. Since the diameter is , we know that the circumference is $14\pi$ . Now, we want to ask how many ways we can divide up the pizza into pieces of inch crust. This is:

$\frac{14\pi}{3}$ or approximately 14.66... pieces.

What you need to do is take this amount and subtract off . This is the amount of crust that is wasted. You can then merely divide it by the original amount of divisions:

$\frac{0.66076571675237...}{14.66076571675237....}=0.04507034144863....$

(You do not need to work in exact area or length. These relative values work fine.)

This is about $4.51\%$ of the pizza that is wasted.

Report an Error

Example Question #2 : How To Find The Length Of The Diameter

A circle has an area of $\textup{in}^{2}$ . What is its diameter?

Possible Answers:

5.62 $\textup{in}$

2.81 $\textup{in}$

8.14 $\textup{in}$

7.57 $\textup{in}$

3.78 $\textup{in}$

Correct answer:

7.57 $\textup{in}$

Explanation:

To solve a question like this, first remember that the area of a circle is defined as:

$A=\pi r^2$

For your data, this is:

$45 = \pi r^2$

To solve for , first divide both sides by $\pi$ . Then take the square root of both sides. Thus you get:

r =3.78469878303024...

The diameter of the circle is just double that:

d=7.56939756606048

Rounding to the nearest hundredth, you get 7.57 .

Report an Error

Example Question #1 : Diameter And Chords

Let represent the area of a circle and represent its circumference. Which of the following equations expresses in terms of ?

Possible Answers:

C^2

Correct answer:

Explanation:

The formula for the area of a circle is $A=\pi r^2$ , and the formula for circumference is $C=2\pi r$ . If we solve for C in terms of r, we get
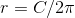
$r=C/2\pi$ .

We can then substitute this value of r into the formula for the area:

$A=\pi r^2$

$=\pi (C/2\pi )^2$

$=C^2\pi /4\pi ^2$

$=C^2/4\pi$

Report an Error

Example Question #531 : Plane Geometry

A circle has a radius of . What is the ratio of its circumference to its area?

Possible Answers:

$4\pi$

$2\pi$

$\pi$

Correct answer:

Explanation:

1. Find the circumference:

$C=\pi d=2\pi r$

$C=2\cdot \pi \cdot 2=4\pi$

2. Find the area:

$A=\pi r^{2}$

$A=\pi \cdot (2^{2})=4\pi$

3. Divide the circumference by the area:

$\frac{C}{A}=\frac{4\pi }{4\pi }=1$

Report an Error

Example Question #532 : Plane Geometry

How far must a racehorse run in a lap race where the course is a circle with an area of $36\pi$ ?

Possible Answers:

$24\pi$

$36\pi$

$48\pi$

$6\pi$

$12\pi$

Correct answer:

$48\pi$

Explanation:

To find the answer, we need to first find the circumference of the circle then mulitply that by 4 because the racehorse is running 4 laps.

We know that the area of the circle is $36\pi$ . We can then find the radius.

$A=\pi r^2$

$36\pi=\pi r^2$

r^2=36

r=6

Because d=2r , our diameter is 12.

We can now find the circumference.

$C=d\pi$

$C=12 \pi$

Multiply the circumference by 4 (our racehorse is running 4 laps) to find the answer.

$12 \pi \cdot 4=48\pi$

Report an Error

Example Question #1 : How To Find The Area Of A Circle

If the equation of a circle is (x – 7)²+ (y + 1)²= 81, what is the area of the circle?

Possible Answers:

81π

6561π

49π

2π

18π

Correct answer:

81π

Explanation:

The equation is already in a circle equation, and the right side of the equation stands for r^2 →r²= 81 and r = 9

The area of a circle is πr², so the area of this circle is 81π.

Report an Error

Example Question #1 : How To Find The Area Of A Circle

Assume π = 3.14

A man would like to put a circular whirlpool in his backyard. He would like the whirlpool to be six feet wide. His backyard is 8 feet long by 7 feet wide. By state regulation, in order to put a whirlpool in a backyard space, the space must be 1.5 times bigger than the pool. Can the man legally install the whirlpool?

Possible Answers:

No, because the area of the backyard is 30 square feet and therefore the whirlpool is too big to meet the legal requirement.

Yes, because the area of the whirlpool is 28.26 square feet and 1.5 times its area would be less than the area of the backyard.

Yes, because the area of the whirlpool is 18.84 square feet and 1.5 times its area would be less than the area of the backyard.

No, because the area of the backyard is smaller than the area of the whirlpool.

No, because the area of the whirlpool is 42.39 square feet and 1.5 times its area would be greater than the area of the backyard.

Correct answer:

Yes, because the area of the whirlpool is 28.26 square feet and 1.5 times its area would be less than the area of the backyard.

Explanation:

If you answered that the whirlpool’s area is 18.84 feet and therefore fits, you are incorrect because 18.84 is the circumference of the whirlpool, not the area.

If you answered that the area of the whirlpool is 56.52 feet, you multiplied the area of the whirlpool by 1.5 and assumed that that was the correct area, not the legal limit.

If you answered that the area of the backyard was smaller than the area of the whirlpool, you did not calculate area correctly.

And if you thought the area of the backyard was 30 feet, you found the perimeter of the backyard, not the area.

The correct answer is that the area of the whirlpool is 28.26 feet and, when multiplied by 1.5 = 42.39, which is smaller than the area of the backyard, which is 56 square feet.

Report an Error

View ACT Math Tutors

MEER
Certified Tutor

IIT , Master's/Graduate, Microelectronics .

View ACT Math Tutors

Margaret
Certified Tutor

View ACT Math Tutors

Sarah
Certified Tutor

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

ACT Math Tutors in Top Cities:

Atlanta ACT Math Tutors, Austin ACT Math Tutors, Boston ACT Math Tutors, Chicago ACT Math Tutors, Dallas Fort Worth ACT Math Tutors, Denver ACT Math Tutors, Houston ACT Math Tutors, Kansas City ACT Math Tutors, Los Angeles ACT Math Tutors, Miami ACT Math Tutors, New York City ACT Math Tutors, Philadelphia ACT Math Tutors, Phoenix ACT Math Tutors, San Diego ACT Math Tutors, San Francisco-Bay Area ACT Math Tutors, Seattle ACT Math Tutors, St. Louis ACT Math Tutors, Tucson ACT Math Tutors, Washington DC ACT Math Tutors

Popular Courses & Classes

ACT Courses & Classes in Philadelphia, GRE Courses & Classes in Los Angeles, ISEE Courses & Classes in Houston, GMAT Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Los Angeles, Spanish Courses & Classes in Washington DC, SAT Courses & Classes in Seattle, SAT Courses & Classes in Miami, Spanish Courses & Classes in Seattle, Spanish Courses & Classes in Los Angeles

Popular Test Prep

GRE Test Prep in San Francisco-Bay Area, GRE Test Prep in Atlanta, SAT Test Prep in San Diego, LSAT Test Prep in New York City, LSAT Test Prep in Denver, ACT Test Prep in Philadelphia, LSAT Test Prep in Chicago, LSAT Test Prep in San Francisco-Bay Area, ACT Test Prep in Denver, ACT Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ACT Math : Plane Geometry

Study concepts, example questions & explanations for ACT Math

All ACT Math Resources

Example Questions

Example Question #4 : How To Find The Length Of The Diameter

Example Question #1 : Diameter

Example Question #2 : Diameter

Example Question #6 : How To Find The Length Of The Diameter

Example Question #2 : How To Find The Length Of The Diameter

Example Question #1 : Diameter And Chords

Example Question #531 : Plane Geometry

Example Question #532 : Plane Geometry

Example Question #1 : How To Find The Area Of A Circle

Example Question #1 : How To Find The Area Of A Circle

All ACT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: