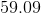ACT Math : Plane Geometry
Study concepts, example questions & explanations for ACT Math
All ACT Math Resources
Example Questions
Example Question #1 : Acute / Obtuse Triangles
Points A, B, C, D are collinear. The measure of ∠ DCE is 130° and of ∠ AEC is 80°. Find the measure of ∠ EAD.
60°
80°
70°
50°
50°
To solve this question, you need to remember that the sum of the angles in a triangle is 180°. You also need to remember supplementary angles. If you know what ∠ DCE is, you also know what ∠ ECA is. Hence you know two angles of the triangle, 180°-80°-50°= 50°.
Example Question #1 : How To Find An Angle In An Acute / Obtuse Triangle
Points A, B, and C are collinear (they lie along the same line). The measure of angle CAD is 
Find the measure of 
The measure of 
Because the measures of the three angles in a triangle must add up to 
Example Question #171 : Act Math
Observe the following image and answer the question below:
Are triangles 
No
Yes
Maybe
Not enough information to decide.
Yes
Two triangles are only congruent if all of their sides are the same length, and all of the corresponding angles are of the same degree. Luckily, we only need three of these six numbers to completely determine the others, as long as we have at least one angle and one side, and any other combination of the other numbers.
In this case, we have two adjacent angles and one side, directly across from one of our angles in both triangles. This can be called the AAS case. We can see from our picture that all of our angles match, and the two sides match as well. They're all in the same position relative to each other on the triangle, so that is enough information to say that the two triangles are congruent.
Example Question #1 : How To Find The Perimeter Of An Acute / Obtuse Triangle
Two similiar triangles have a ratio of perimeters of 
If the smaller triangle has sides of 3, 7, and 5, what is the perimeter of the larger triangle.
Adding the sides gives a perimeter of 15 for the smaller triangle. Multipying by the given ratio of 
Example Question #2 : Acute / Obtuse Triangles
Two similiar triangles exist where the ratio of perimeters is 4:5 for the smaller to the larger triangle. If the larger triangle has sides of 6, 7, and 12 inches, what is the perimeter, in inches, of the smaller triangle?
20
25
18
23
20
The larger triangle has a perimeter of 25 inches. Therefore, using a 4:5 ratio, the smaller triangle's perimeter will be 20 inches.
Example Question #2 : How To Find The Perimeter Of An Acute / Obtuse Triangle
Two similar triangles' perimeters are in a ratio of 
1. Find the perimeter of the larger triangle:
2. Use the given ratio to find the perimeter of the smaller triangle:
Cross multiply and solve:
Example Question #4 : How To Find The Perimeter Of An Acute / Obtuse Triangle
There are two similar triangles. Their perimeters are in a ratio of 
Use proportions to solve for the perimeter of the larger triangle:
Cross multiply and solve:
Example Question #171 : Act Math
Two similar triangles have perimeteres in the ratio 
Since the perimeter of the smaller triangle is 
In cross multiplying this identity, we get 
Example Question #11 : Acute / Obtuse Triangles
What is the value of 
Cannot be calculated
Begin by filling in the missing angle for your triangle. Since a triangle has a total of 
Draw out the figure:
Now, to solve this, you will need some trigonometry. Use the Law of Sines to calculate the value:
Solving for 
Rounding, this is 
Example Question #171 : Act Math
The base of a triangle is 
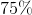
The formula for the area of a triangle is
If the area is equal to 48 cm2 and the base is 8 cm, then the initial height is:
If 12 is decreased by 75% then
Therefore the final area is
Certified Tutor
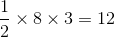
Certified Tutor
All ACT Math Resources
