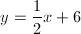All ACT Math Resources
Example Questions
Example Question #1 : Coordinate Geometry
What line is parallel to 

Start by converting the original equation to slop-intercept form.
The slope of this line is 
Plug the y-intercept into the slope-intercept equation to get the final answer.
Example Question #1 : How To Find The Equation Of A Parallel Line
What is the equation of a line that is parallel to the line 

The line parallel to 



Therefore, the equation of the line is 
Example Question #2 : Coordinate Geometry
What line is parallel to 

Converting the given line to slope-intercept form we get the following equation:
For parallel lines, the slopes must be equal, so the slope of the new line must also be 
Use the y-intercept in the slope-intercept equation to find the final answer.
Example Question #391 : Sat Mathematics
What line is parallel to 

None of the answers are correct
Find the slope of the given line: 

Parallel lines have the same slope, so now we need to find the equation of a line with slope 

So,
Thus, the new equation is
Example Question #1 : How To Find The Equation Of A Parallel Line
Which of these formulas could be a formula for a line perpendicular to the line 
This is a two-step problem. First, the slope of the original line needs to be found. The slope will be represented by "


So the slope of the original line is 

So, the slope is 
Example Question #4 : How To Find The Equation Of A Parallel Line
Which of the following is a line that is parallel to the line defined by the equation 
Since parallel lines have equal slopes, you should find the slope of the line given to you. The easiest way to do this is to solve the equation so that its form is 

Take your equation:
First, subract 
Next, subtract 
Finally, divide by 

Thus, your slope is 
Among the options provided only 

First, subtract 
Then, divide by 
Example Question #1 : How To Find The Equation Of A Parallel Line
Which of the following answer choices gives the equation of a line parallel to the line:
Parallel lines have the same slope but different y-intercepts. When the equations of two lines are the same they have infinitely many points in common, whereas parallel lines have no points in common.
Our equation is given in slope-intercept form,
where 

Therefore we want to find an equation that has the same 

Thus,

Example Question #1 : How To Find The Equation Of A Parallel Line
What is the equation of a line parallel to the line given by the equation:
Parallel lines have the same slope and differing y-intercepts. Since 
Example Question #11 : How To Find The Equation Of A Parallel Line
Which of the following is the equation of a line parallel to the line given by the equation:
Parallel lines have the same slope and different y-intercepts. If their y-intercepts and slopes are the same they are the same line, and therefore not parallel. Thus the only one that fits the description is:
Example Question #22 : Algebra
A line passes through 


The first step in solving for a parallel line is to find the slope of the original line. In this case, 
Our new line has an equal slope (the definition of parallel) and passes through 



Thus, the slope intercept equation for our parallel line is 
Certified Tutor
All ACT Math Resources