Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Middle Level Math : Plane Geometry

Study concepts, example questions & explanations for SSAT Middle Level Math

Create An Account

All SSAT Middle Level Math Resources

10 Diagnostic Tests 225 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2 : How To Find The Area Of A Triangle

The hypotenuse of a right triangle is 25 inches; it has one leg 15 inches long. Give its area in square feet.

Possible Answers:

$2 \frac{29}{48} \textrm{ ft}^{2}$

$1 \frac{13}{24}\textrm{ ft} ^{2}$

$2 \frac{5}{48} \textrm{ ft}^{2}$

$1 \frac{1}{24} \textrm{ ft}^{2}$

$1 \frac{1}{12} \textrm{ ft}^{2}$

Correct answer:

$1 \frac{1}{24} \textrm{ ft}^{2}$

Explanation:

The area of a right triangle is half the product of the lengths of its legs, so we need to use the Pythagorean Theorem to find the length of the other leg. Set c = 25, b = 15 :

$a^{2} = c^{2} - b^{2}$

$a^{2} = 25^{2} - 15^{2}$

$a^{2} = 625-225$

$a^{2} =400$

$a = \sqrt{400} = 20$

The legs are 15 and 20 inches long. Divide both dimensions by 12 to convert from inches to feet:

$20 \div 12 = \frac{5}{3}$ feet

$15 \div 12 = \frac{5}{4}$ feet

Now find half their product:

$A = \frac{1}{2} \times \frac{5}{3}\times \frac{5}{4} = \frac{25}{24} = 1 \frac{1}{24}$ square feet

Report an Error

Example Question #3 : How To Find The Area Of A Triangle

Rectangles

Note: Figure NOT drawn to scale.

What percent of the above figure is green?

Possible Answers:

$68 \frac{8}{9} \%$

$62\frac{1}{2} \%$

$78 \frac{3}{4} \%$

The correct answer is not given among the other choices.

$73 \frac{1}{3} \%$

Correct answer:

$73 \frac{1}{3} \%$

Explanation:

The area of the entire rectangle is the product of its length and width, or

$120 \times 50 = 6,000$ .

The area of the right triangle is half the product of its legs, or

$\frac{1}{2} \times 40 \times 80 = 1,600$

The area of the green region is therefore the difference of the two, or

6,000 - 1,600 = 4,400 .

The green region is therefore

$\frac{4,400}{6,000} \times 100 = 73 \frac{1}{3} \%$

of the rectangle.

Report an Error

Example Question #4 : How To Find The Area Of A Triangle

Rectangles

Note: Figure NOT drawn to scale.

Refer to the above diagram. Give the ratio of the area of the green region to that of the white region.

Possible Answers:

2:1

21:9

11:4

23:7

The correct answer is not given among the other choices.

Correct answer:

11:4

Explanation:

The area of the entire rectangle is the product of its length and width, or

$120 \times 50 = 6,000$ .

The area of the right triangle is half the product of its legs, or

$\frac{1}{2} \times 40 \times 80 = 1,600$

The area of the green region is therefore the difference of the two, or

6,000 - 1,600 = 4,400 .

The ratio of the area of the green region to that of the white region is

$\frac{4,400}{1,600} = \frac{4,400 \div 400}{1,600\div 400} = \frac{11}{4}$

That is, 11 to 4.

Report an Error

Example Question #3 : Area Of A Triangle

A triangle has a height of 9 inches and a base that is one third as long as the height. What is the area of the triangle, in square inches?

Possible Answers:

None of these

$\frac{27}{2}$

Correct answer:

$\frac{27}{2}$

Explanation:

The area of a triangle is found by multiplying the base times the height, divided by 2.

$\text{Area} =\frac{\text{base}\times \text{height}}{2}$

Given that the height is 9 inches, and the base is one third of the height, the base will be 3 inches.

$b=h\div3=9\div3=3$

We now have both the base (3) and height (9) of the triangle. We can use the equation to solve for the area.

$\text{Area} =\frac{9\times 3}{2}$

$\text{Area} =\frac{27}{2}$

The fraction cannot be simplified.

Report an Error

Example Question #241 : Ssat Middle Level Quantitative (Math)

The hypotenuse of a right triangle is feet; it has one leg feet long. Give its area in square inches.

Possible Answers:

$101,088 \textrm{ in}^{2}$

$42,120 \textrm{ in}^{2}$

$43,120 \textrm{ in}^{2}$

$77,760 \textrm{ in}^{2}$

$38,880 \textrm{ in}^{2}$

Correct answer:

$38,880 \textrm{ in}^{2}$

Explanation:

The area of a right triangle is half the product of the lengths of its legs, so we need to use the Pythagorean Theorem to find the length of the other leg. Set c = 39 , b = 36 :

$a^{2} = c^{2} - b^{2}$

$a^{2} = 39 ^{2} - 36^{2}$

$a^{2} = 1,521 - 1,296$

$a^{2} = 225$

$a = \sqrt{225} = 15$

The legs have length and feet; multiply both dimensions by to convert to inches:

$15 \times 12 = 180$ inches

$36 \times 12 = 432$ inches.

Now find half the product:

$A = \frac{1}{2} \cdot 180 \cdot 432 = 38,880\ in^{2}$

Report an Error

Example Question #242 : Ssat Middle Level Quantitative (Math)

What is the area (in square feet) of a triangle with a base of feet and a height of feet?

Possible Answers:

Correct answer:

Explanation:

The area of a triangle is found by multiplying the base times the height, divided by .

$Area =\frac{base\cdot height}{2}$

$Area =\frac{4\cdot 7}{2}$

Area=14

Report an Error

Example Question #23 : Area Of A Triangle

What is the area of a triangle with a base of and a height of ?

Possible Answers:

Correct answer:

Explanation:

The formula for the area of a triangle is $\dpi{100} Area=\frac{1}{2}\times base\times height$ .

Plug the given values into the formula to solve:

$\dpi{100} Area=\frac{1}{2}\times 12\times 3$

$\dpi{100} Area=\frac{1}{2}\times 36$

$\dpi{100} Area=18$

Report an Error

Example Question #15 : How To Find The Area Of A Triangle

Right triangle 2

Give the perimeter of the above triangle in feet.

Possible Answers:

$2 \frac{2}{3}\textrm{ ft }$

$3 \frac{1}{3}\textrm{ ft }$

$3 \frac{1}{2}\textrm{ ft }$

$2 \frac{1}{2}\textrm{ ft }$

Correct answer:

$2 \frac{1}{2}\textrm{ ft }$

Explanation:

The perimeter of the triangle - the sum of the lengths of its sides - is

5 + 12 + 13 = 30 inches.

Divide by 12 to convert to feet:

$30 \div 12 = 2 \textrm{ R }6$

As a fraction, this is $2 \frac{6}{12}$ or $2 \frac{1}{2}$ feet,

Report an Error

Example Question #244 : Ssat Middle Level Quantitative (Math)

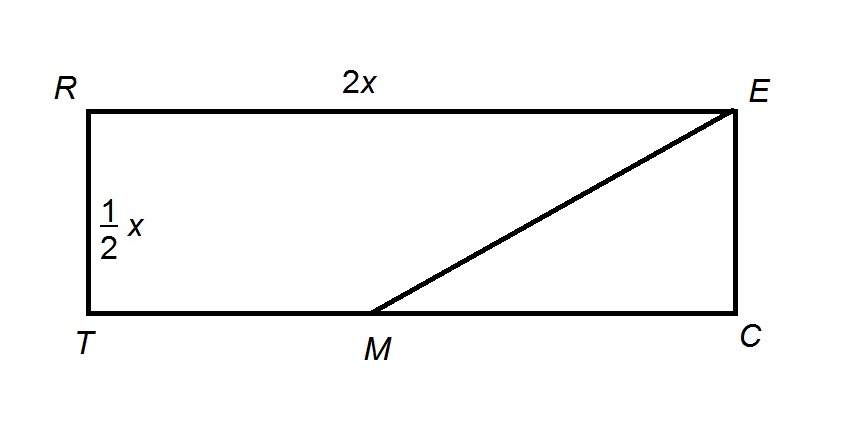
Rectangles 3

The above diagram shows Rectangle RECT , with midpoint of $\overline{CT}$ .

The area of $\bigtriangleup ECM$ is 225. Evaluate

Possible Answers:

Correct answer:

Explanation:

is the midpoint of $\overline{CT}$ , so $\bigtriangleup ECM$ has as its base $CM= \frac{1}{2} \cdot CT = \frac{1}{2} \cdot 2x =x$ ; its height is $EC = \frac{1}{2} x$ .

Its area is half their product, or

$\frac{1}{2} \cdot x \cdot \frac{1}{2} x = \frac{1}{4} x^{2}$

Set this equal to 225:

$\frac{1}{4} x^{2} = 225$

$4 \cdot \frac{1}{4} x^{2} = 4 \cdot 225$

$x^{2} = 900$

$x = \sqrt{900}= 30$ .

Report an Error

Example Question #17 : Plane Geometry

Find the area of a triangle with a height of 12in and a base that is half the height.

Possible Answers:

$30\text{in}^2$

$6\text{in}^2$

$18\text{in}^2$

$24\text{in}^2$

$36\text{in}^2$

Correct answer:

$36\text{in}^2$

Explanation:

To find the area of a triangle, we will use the following formula:

$A = \frac{1}{2} \cdot b \cdot h$

where b is the base and h is the height of the triangle.

Now, we know the height of the triangle is 12in. We also know the base of the triangle is half of the height. Therefore, the base of the triangle is 6in.

So, we can substitute. We get

$A = \frac{1}{2} \cdot 6\text{in} \cdot 12\text{in}$

$A = 3\text{in} \cdot 12\text{in}$

$A = 36\text{in}^2$

Report an Error

View SSAT- Middle Level Tutors

Daniel
Certified Tutor

Stony Brook University, Bachelors, Biology, General. SUNY College of Optometry, PHD, Optometry.

View SSAT- Middle Level Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

View SSAT- Middle Level Tutors

Gabby
Certified Tutor

University of Oregon, Bachelor in Arts, Journalism. University of Oregon, Current Grad Student, Communication, General.

All SSAT Middle Level Math Resources

10 Diagnostic Tests 225 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Algebra Tutors in Seattle, Statistics Tutors in San Francisco-Bay Area, ACT Tutors in Dallas Fort Worth, Math Tutors in Denver, ISEE Tutors in Dallas Fort Worth, LSAT Tutors in Philadelphia, Spanish Tutors in Phoenix, Physics Tutors in Seattle, Spanish Tutors in New York City, Chemistry Tutors in Miami

Popular Courses & Classes

SSAT Courses & Classes in Houston, SSAT Courses & Classes in Seattle, SAT Courses & Classes in Boston, Spanish Courses & Classes in Houston, LSAT Courses & Classes in Phoenix, LSAT Courses & Classes in Philadelphia, ISEE Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Los Angeles, GRE Courses & Classes in Miami

Popular Test Prep

GRE Test Prep in Los Angeles, MCAT Test Prep in Chicago, SAT Test Prep in Washington DC, SSAT Test Prep in Los Angeles, LSAT Test Prep in New York City, GMAT Test Prep in Washington DC, LSAT Test Prep in Miami, LSAT Test Prep in Houston, MCAT Test Prep in Philadelphia, ISEE Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Middle Level Math : Plane Geometry

Study concepts, example questions & explanations for SSAT Middle Level Math

All SSAT Middle Level Math Resources

Example Questions

Example Question #2 : How To Find The Area Of A Triangle

Example Question #3 : How To Find The Area Of A Triangle

Example Question #4 : How To Find The Area Of A Triangle

Example Question #3 : Area Of A Triangle

Example Question #241 : Ssat Middle Level Quantitative (Math)

Example Question #242 : Ssat Middle Level Quantitative (Math)

Example Question #23 : Area Of A Triangle

Example Question #15 : How To Find The Area Of A Triangle

Example Question #244 : Ssat Middle Level Quantitative (Math)

Example Question #17 : Plane Geometry

All SSAT Middle Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: