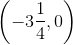Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : Coordinate Geometry

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #11 : How To Find X Or Y Intercept

What are the -intercept(s) of the following line:

y=x^2+12x+27

Possible Answers:

x=3,9

x=-3,9

x=-3,-9

x=3,-9

x=27,1

Correct answer:

x=-3,-9

Explanation:

We can factor y=x^2+12x+27 and set equal to zero to determine the -intercepts.

y=(x+3)(x+9) satisfies this equation.

Therefore our -intercepts are and .

Report an Error

Example Question #11 : How To Find X Or Y Intercept

Which of the following lines does not intersect the line 4x + y = 7 ?

Possible Answers:

$y=\frac{1}{4}x-7$

y=4x-7

y = 4x + 7

y = -4x + 5

$y=-\frac{1}{4}x+11$

Correct answer:

y = -4x + 5

Explanation:

Parallel lines never intersect, so you are looking for a line that has the same slope as the one given. The slope of the given line is –4, and the slope of the line in y = –4x + 5 is –4 as well. Since these two lines have equal slopes, they will run parallel and can never intersect.

Report an Error

Example Question #12 : How To Find X Or Y Intercept

Find the y-intercept of y = 3x^2 +2x + 7 .

Possible Answers:

Correct answer:

Explanation:

To find the y-intercept, set x equal to zero and solve for y.

This gives y = 3(0)² + 2(0) +7 = 7.

Report an Error

Example Question #173 : Geometry

The slope of a line is $m=\frac{4}{3}$ . The line passes through $(2,7)$ . What is the x-intercept?

Possible Answers:

$(0,9\frac{2}{3})$

None of the available answers

$\left ( -3\frac{1}{4},0\right )$

$(4\frac{1}{3},0)$

$(0,4.3)$

Correct answer:

$\left ( -3\frac{1}{4},0\right )$

Explanation:

The equation for a line is:

$y=mx+b$ , or in this case

$y=\frac{4}{3}x+b$

We can solve for $b$ by plugging in the values given

$7=\frac{4}{3}\times 2+b$

$7=2\frac{2}{3}+b$

$b=7-2\frac{2}{3}=4\frac{1}{3}$

Our line is now

$y=\frac{4}{3}x+4\frac{1}{3}$

Our x-intercept occurs when $\dpi{100} y=0$ , so plugging in and solving for $\dpi{100} x$ :

$\dpi{100} 0=\frac{4}{3}x+4\frac{1}{3}$

$\dpi{100} -\frac{13}{3}=\frac{4}{3}x$

$\dpi{100} x=-\frac{13}{4}$

Report an Error

Example Question #15 : How To Find X Or Y Intercept

Determine the x-intercept for the equation: y=3x+9

Possible Answers:

$-\frac{1}{9}$

$\frac{1}{3}$

Correct answer:

Explanation:

The x-intercept is the x-value when the value of y=0 . Substitute this value and solve for .

0=3x+9

-9=3x

$x=\frac{-9}{3}=-3$

The x-intercept is .

Report an Error

Example Question #14 : How To Find X Or Y Intercept

What is the y intercept of y=12x+3?

Possible Answers:

y=-3

y=3

$x=\frac{-3}{12}$

y=12

The line does not cross the y axis.

Correct answer:

y=3

Explanation:

To find the y intercept, substitute x=0

y=12(0)+3

y=3

Report an Error

Example Question #15 : How To Find X Or Y Intercept

At what point does the line $\pi x + \frac{\pi}{2}y - 2\pi = 0$ intersect the y-axis?

Possible Answers:

(0.5, 0.5)

None of the given answers

(0, 4)

$(\pi, \frac{1}{2}\pi)$

$(\pi, \pi)$

Correct answer:

(0, 4)

Explanation:

We know that in slope-intercept form, y=mx+b , that represents the y-intercept. So, let's rewrite this line and put it in slope-intercept form.

$\pi x + \frac{\pi}{2}y - 2\pi = 0$

$\frac{\pi}{2}y = -\pi x + 2\pi$

$y = \frac{2}{\pi}(-\pi x+2\pi)$

y = -2x +4

Therefore, when x=0 , y=4 . With this in mind, our y-intercept is (0, 4) .

Report an Error

Example Question #16 : How To Find X Or Y Intercept

Give the area of the triangle on the coordinate plane that is bounded by the -axis, and the lines of the equations y = x and $y = -\frac{4}{3} x + 3$ .

Possible Answers:

$5\frac{11}{14}$

$3 \frac{6}{7}$

$1 \frac{13}{14}$

$2 \frac{25}{28}$

None of the other choices gives the correct response.

Correct answer:

$1 \frac{13}{14}$

Explanation:

It is necessary to find the vertices of the triangle, which can be done by finding the three points at which two of the three lines intersect.

The intersection of the -axis - the line x = 0 - and the line of the equation y = x , is found by noting that if x = 0 , then, by substitution, y = 0 ; this point of intersection is at (0,0) , the origin.

The intersection of the -axis and the line of the equation $y = -\frac{4}{3} x + 3$ is the -intercept of the latter line. Since its equation is written in slope-intercept form y = mx+b , with the -coordinate of the -intercept, this intercept is (0, 3) .

The intersection of the lines with equations y = x and $y = -\frac{4}{3} x + 3$ can be found using the substitution method, setting y = x in the latter equation and solving for :

$x = -\frac{4}{3} x + 3$

$x+ \frac{4}{3} x= -\frac{4}{3} x + 3 + \frac{4}{3} x$

$\frac{7}{3} x=3$

$\frac{3} {7} \cdot \frac{7}{3} x= \frac{3} {7} \cdot 3$

$x= \frac{9} {7} =1\frac{2}{7}$

Since y = x , $y =1\frac{2}{7}$ , making $\left ( 1\frac{2}{7}, 1\frac{2}{7} \right )$ the point of intersection.

The lines in question are graphed below, and the triangle they bound is shaded:

Triangle z

If we take the vertical side as the base, its length is seen to be 3; the height is the horizontal distance to the opposite vertex, which is its -coordinate $1 \frac{2}{7}$ . The area is half the product of the two, or

$A = \frac{1}{2} \cdot 3 \cdot 1 \frac{2}{7} = 1 \frac{13}{14}$ .

Report an Error

Example Question #26 : X And Y Intercept

Give the area of the triangle on the coordinate plane that is bounded by the lines of the equations x = -4 , y = x and y = 4x- 7 .

Possible Answers:

$72 \frac{5}{6}$

$54 \frac{5}{8}$

$60\frac{1}{6}$

Correct answer:

$60\frac{1}{6}$

Explanation:

It is necessary to find the coordinates of the vertices of the triangle, each of which is the intersection of two of the three lines.

The intersection of the lines of the equations x = -4 and y = x can easily be found by noting that since x = -4 , by substitution, y = -4 , making the point of intersection (-4. -4) .

The intersection of the lines of the equations x = -4 and y = 4x- 7 can be found by substituting for in the latter equation and evaluating :

y = 4 (-4)- 7 = -16 - 7 = -23

The point of intersection is (-4, -23) .

The intersection of the lines of the equations y = x and y = 4x- 7 can be found by substituting for in the latter equation and solving for :

4x- 7 = x

4x- 7 - x + 7 = x - x + 7

3x = 7

$\frac{3x }{3}= \frac{7}{3}$

$x = 2\frac{1}{3}$

y = x , so $y = 2\frac{1}{3}$ , and this point of intersection is $\left ( 2\frac{1}{3}, 2\frac{1}{3} \right )$ .

The lines in question are graphed below, and the triangle they bound is shaded:

Triangle z

We can take the vertical side as the base of the triangle; its length is the difference of the -coordinates:

b = -4 - (-23) = 19

The height is the horizontal distance from this side to the opposite side, which is the difference of the -coordinates:

$h= 2 \frac{1}{3} - (-4) = 6 \frac{1}{3}$

The area is half their product:

$A = \frac{1}{2} bh = \frac{1}{2} \cdot 19 \cdot 6 \frac{1}{3} =60\frac{1}{6}$

Report an Error

Example Question #21 : How To Find X Or Y Intercept

Give the area of the triangle on the coordinate plane that is bounded by the axes and the line of the equation y = 2 x - 15 .

Possible Answers:

$56 \frac{1}{4}$

$112\frac{1}{2}$

225

Correct answer:

$56 \frac{1}{4}$

Explanation:

It is necessary to find the vertices of the triangle, each of which is a point at which two of the three lines intersect.

The two axes intersect at the origin, making this one vertex.

The other two points of intersection are the intercepts of the line of equation y = 2 x - 15 . Since this equation is in slope-intercept form y = mx+ b , where is the -coordinate of the -intercept, then b = -15 , and the -intercept is the point (0, -15) . The -coordinate of the -intercept, , can be found by setting y = 0 , x= a and solving for :

2 x - 15 = y

2 a - 15 = 0

2 a = 15

$a = \frac{15}{2} = 7 \frac{1}{2}$ .

The three vertices are located at $(0,0), \left ( 7\frac{1}{2}, 0 \right ), (0. -15)$

The line in question is shown below, with the bounded triangle shaded in:

Triangle z

The lengths of its legs are equal to the absolute values of the nonzero coordinates of its two intercepts - $a' = 7 \frac{1}{2}, b' = 15$ . The area of this right triangle is half their product:

$A = \frac{1}{2} a 'b' = \frac{1}{2} \cdot 7\frac{1}{2} \cdot 15 = 56 \frac{1}{4}$ .

Report an Error

View SAT Mathematics Tutors

Kenneth
Certified Tutor

North Georgia University, Bachelors, Accounting. Brenau University, Masters, Business.

View SAT Mathematics Tutors

Troy
Certified Tutor

Rice University, Bachelor in Arts, Kinesiology.

View SAT Mathematics Tutors

Irina
Certified Tutor

New York University, Bachelors, Biochemistry.

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

GMAT Courses & Classes in San Diego, Spanish Courses & Classes in Dallas Fort Worth, GRE Courses & Classes in San Diego, ACT Courses & Classes in Seattle, SAT Courses & Classes in Los Angeles, SAT Courses & Classes in Seattle, ACT Courses & Classes in Phoenix, SSAT Courses & Classes in Boston, GMAT Courses & Classes in Atlanta, Spanish Courses & Classes in Denver

Popular Test Prep

MCAT Test Prep in Denver, SSAT Test Prep in Seattle, ACT Test Prep in Seattle, GRE Test Prep in New York City, SSAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in New York City, SAT Test Prep in Dallas Fort Worth, ACT Test Prep in Phoenix, SSAT Test Prep in San Diego, MCAT Test Prep in Seattle

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : Coordinate Geometry

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #11 : How To Find X Or Y Intercept

Example Question #11 : How To Find X Or Y Intercept

Example Question #12 : How To Find X Or Y Intercept

Example Question #173 : Geometry

Example Question #15 : How To Find X Or Y Intercept

Example Question #14 : How To Find X Or Y Intercept

Example Question #15 : How To Find X Or Y Intercept

Example Question #16 : How To Find X Or Y Intercept

Example Question #26 : X And Y Intercept

Example Question #21 : How To Find X Or Y Intercept

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: