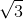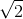MAP 8th Grade Math : The Real and Complex Number Systems
Study concepts, example questions & explanations for MAP 8th Grade Math
All MAP 8th Grade Math Resources
Example Questions
Example Question #1 : The Real And Complex Number Systems
Which of the following answer choices displays a rational number?
Our answer choices consist of two types of numbers: rational numbers and irrational numbers. In order to correctly answer this question, we need to know the difference between the two types of numbers.
Rational numbers are numbers that we use most often, and can be written as a simple fraction.
Irrational numbers cannot be written as fractions, and are numbers that have decimal places that never repeat or end.
In this case, 
Example Question #2 : The Real And Complex Number Systems
Which of the following answer choices displays an irrational number?
Our answer choices consist of two types of numbers: rational numbers and irrational numbers. In order to correctly answer this question, we need to know the difference between the two types of numbers.
Rational numbers are numbers that we use most often, and can be written as a simple fraction.
Irrational numbers cannot be written as fractions, and are numbers that have decimal places that never repeat or end.
In this case, 
Example Question #3 : The Real And Complex Number Systems
Solve and leave your answer in scientific notation:
The first step to solving this problem is to combine like terms:
Next, we can solve our two separate multiplication problems starting with the expression on the left:
In order to solve the next expression, we need to recall our exponent rules from a previous lesson:
When our base numbers are equal to each other, like in this problem, we can add our exponents together using the following formula:
Let's apply this rule to our problem:
The question asked us to leave our answer in scientific notation; thus, 
Certified Tutor
All MAP 8th Grade Math Resources
