Call Now to Set Up Tutoring:

(888) 888-0446

ISEE Upper Level Quantitative : How to find the missing part of a list

Study concepts, example questions & explanations for ISEE Upper Level Quantitative

Create An Account

All ISEE Upper Level Quantitative Resources

8 Diagnostic Tests 234 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #181 : Data Analysis And Probability

An arithmetic sequence begins

$\square, \bigtriangleup , \bigcirc, 90, 107,...$

What number replaces the square?

Possible Answers:

141

158

Correct answer:

Explanation:

Since this is an arithmetic sequence, each entry in the sequence is obtained by adding the same number to the previous entry - this number is

107-90 = 17

Let be the number in the square. Then

$n + 3 \cdot 17 = 90$

n + 51 = 90

n + 51 - 51 = 90 - 51

n = 39

Report an Error

Example Question #31 : How To Find The Missing Part Of A List

The first two terms of an arithmetic sequence are

5, G,...

Which of the following expressions is equivalent to the fifth term?

Possible Answers:

5G - 25

4G-15

5G-20

4G-20

3G-15

Correct answer:

4G-15

Explanation:

An arithmetic sequence is formed by adding the same expression to each term to get the next term; this common difference is

G - 5 .

To obtain the fifth term, add G - 5 to the second term three times - equivalently, add three times this to the second term;

G + 3 (G - 5) = G + 3G - 15 = 4G -15

Report an Error

Example Question #31 : How To Find The Missing Part Of A List

A geometric sequence begins

$5, 35, \square, \bigtriangleup , \bigcirc...$ .

What number replaces the circle?

Possible Answers:

1,715

275

12,005

125

Correct answer:

12,005

Explanation:

Since this is a geometric sequence, each entry in the sequence is obtained by multiplying the previous entry by the same number - this number is

$35 \div 5 = 7$ .

Now we can find the next three entries in the sequence:

$35 \times 7 = 245$

This replaces the square.

$245 \times 7 = 1,715$

1,715 replaces the triangle.

$1,715\times 7 = 12,005$

12,005 replaces the circle and is therefore the correct answer.

Report an Error

Example Question #184 : Data Analysis And Probability

An arithmetic sequence begins

$5, 35, \square, \bigtriangleup , \bigcirc...$

What number replaces the circle?

Possible Answers:

1,715

12,005

125

275

Correct answer:

125

Explanation:

Since this is an arithmetic sequence, each entry in the sequence is obtained by adding the same number to the previous entry - this number is

35- 5 = 30 .

The next three entries in the sequence are computed as follows:

35 + 30 = 65 , which replaces the square

65 + 30 = 95 , which replaces the triangle

95 + 30 = 125 , which replaces the circle

Report an Error

Example Question #31 : How To Find The Missing Part Of A List

A geometric sequence begins

$\square, \bigtriangleup , \bigcirc, 400, 8000,...$

What number replaces the square?

Possible Answers:

-14,800

-22,400

0.05

Correct answer:

0.05

Explanation:

Each term of a geometric sequence is obtained by multiplying the previous one by the same number (common ratio); this number is

$8000 \div 400 = 20$ .

Let be the number in the square.

$s \cdot 20 ^{3} = 400$

$s \cdot 8000 = 400$

$s \cdot 8000 \div 8000= 400 \div 8000$

s = 0.05

Report an Error

Example Question #31 : How To Find The Missing Part Of A List

The Fibonacci sequence is formed as follows:

$F_{1} = 1$

$F_{2}= 1$

For all integers n > 2 , $F_{n}= F_{n-1}+ F_{n-2}$

Which of the following is true of $F_{1,000}$ , the one-thousandth number in this sequence?

Possible Answers:

$F_{1,000} =2 F_{997}+3F_{998}$

$F_{1,000} = 3F_{997}+ 2F_{998}$

$F_{1,000} = F_{997}+ F_{998}$

$F_{1,000} = 2 F_{997}+ F_{998}$

$F_{1,000} = F_{997}+ 2F_{998}$

Correct answer:

$F_{1,000} = F_{997}+ 2F_{998}$

Explanation:

To express $F_{1,000}$ , the one-thousandth term of the sequence, in terms of $F_{997}$ and $F_{998}$ alone, we note that, by definition of the sequence, each term, except for the first two, is equal to the sum of the previous two. Therefore,

$F_{999} = F_{997}+ F_{998}$

Also

$F_{1,000} = F_{998}+ F_{999}$ , and, substituting:

$F_{1,000} = F_{998}+ \left ( F_{997}+ F_{998} \right )$

and

$F_{1,000} = F_{997}+ 2 F_{998}$ ,

the correct choice.

Report an Error

Example Question #181 : Data Analysis

The Fibonacci sequence is defined as follows:

$F_{1} = F_{2} = 1$

For integers n > 2 , $F_{n} = F_{n-1}+ F_{n-2}$ .

Which is the greater quantity?

(a) $2 \cdot F_{50}$

(b) $F_{51}$

Possible Answers:

(b) is greater

It is impossible to determine which is greater from the information given.

(a) is greater

(a) and (b) are equal

Correct answer:

(a) is greater

Explanation:

The Fibonacci sequence begins as follows:

1,1,2,3,5,8,13,...

This sequence is seen to be an increasing sequence. Therefore, each term is greater than its preceding term. In particular,

$F_{50 }> F_{49}$

If we substitute 51 for in the rule of the sequence, we get

$F_{n} = F_{n-1}+ F_{n-2}$

$F_{51} = F_{51-1}+ F_{51-2}$

$F_{51} = F_{50}+ F_{49}$

$F_{50 }> F_{49}$ , so

$F_{50 }+ F_{50} > F_{49} + F_{50 }$

$2 \cdot F_{50} > F_{51 }$

This makes (a) greater.

Report an Error

Example Question #191 : Data Analysis And Probability

Define a sequence as follows:

$a_{1} = 3$

$a_{2} = 1$

For all integers n > 2 , $a_{n} = 3a_{n-1} - a_{n-2}$ .

Which of the following expressions is equal to 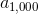$a_{1,000}$ ?

Possible Answers:

$a_{998 } -9a_{997}$

$8a_{998 } - a_{997}$

$8a_{998 } - 3 a_{997}$

$9a_{998 } - 2a_{997}$

$9a_{998 } - a_{997}$

Correct answer:

$8a_{998 } - 3 a_{997}$

Explanation:

Setting 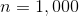n = 1,000 :

$a_{n} = 3a_{n-1} - a_{n-2}$

$a_{1,000} = 3a_{1,000-1} - a_{1,000-2}$

$a_{1,000} = 3a_{999} - a_{998}$

Similarly,

$a_{999} = 3a_{998 } - a_{997}$

Substituting:

$a_{1,000} = 3 (3a_{998 } - a_{997}) - a_{998}$

$=9a_{998 } - 3 a_{997} - a_{998}$

$=8a_{998 } - 3 a_{997}$

Report an Error

View Tutors

Kaitlyn
Certified Tutor

Fairfield University, Bachelor of Science, Biology, General. NUI Galway Ireland, Master of Science, Neuroscience.

View Tutors

Magda
Certified Tutor

Heritage College, Bachelor of Science, Education.

View Tutors

Mary
Certified Tutor

Oklahoma Wesleyan University, Bachelor of Science, Business Teacher Education. University of Phoenix-Oklahoma City Campus, Ma...

All ISEE Upper Level Quantitative Resources

8 Diagnostic Tests 234 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Computer Science Tutors in Atlanta, Math Tutors in Philadelphia, Spanish Tutors in Phoenix, English Tutors in Phoenix, Reading Tutors in San Diego, SSAT Tutors in Philadelphia, LSAT Tutors in Denver, Algebra Tutors in Seattle, English Tutors in Denver, Math Tutors in Seattle

Popular Courses & Classes

LSAT Courses & Classes in Chicago, ISEE Courses & Classes in Philadelphia, ISEE Courses & Classes in Seattle, GMAT Courses & Classes in Atlanta, ACT Courses & Classes in Philadelphia, GRE Courses & Classes in Washington DC, SSAT Courses & Classes in Dallas Fort Worth, Spanish Courses & Classes in San Diego, GRE Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Seattle

Popular Test Prep

ISEE Test Prep in Seattle, MCAT Test Prep in Dallas Fort Worth, ISEE Test Prep in Denver, ACT Test Prep in Houston, MCAT Test Prep in Boston, LSAT Test Prep in Houston, LSAT Test Prep in Los Angeles, ISEE Test Prep in New York City, LSAT Test Prep in Chicago, SAT Test Prep in Seattle

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ISEE Upper Level Quantitative : How to find the missing part of a list

Study concepts, example questions & explanations for ISEE Upper Level Quantitative

All ISEE Upper Level Quantitative Resources

Example Questions

Example Question #181 : Data Analysis And Probability

Example Question #31 : How To Find The Missing Part Of A List

Example Question #31 : How To Find The Missing Part Of A List

Example Question #184 : Data Analysis And Probability

Example Question #31 : How To Find The Missing Part Of A List

Example Question #31 : How To Find The Missing Part Of A List

Example Question #181 : Data Analysis

Example Question #191 : Data Analysis And Probability

All ISEE Upper Level Quantitative Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: