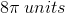We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Intermediate Geometry : Solid Geometry

Study concepts, example questions & explanations for Intermediate Geometry

Create An Account

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #33 : Spheres

A sphere with a radius of $\frac{6}{5}$ $\displaystyle \frac{6}{5}$ is cut out of a cube that has a side length of $\displaystyle 5$. What is the volume of the resulting figure?

Possible Answers:

102.38 $\displaystyle 102.38$

117.76 $\displaystyle 117.76$

114.41 $\displaystyle 114.41$

111.09 $\displaystyle 111.09$

Correct answer:

117.76 $\displaystyle 117.76$

Explanation:

Since the cube is bigger, we will be subtracting the volume of the sphere from the volume of the cube.

Start by recalling how to find the volume of a sphere.

$\text{Volume of Sphere}=\frac{4}{3}\pi r^3$ $\displaystyle \text{Volume of Sphere}=\frac{4}{3}\pi r^3$

Plug in the given radius to find the volume.

$\text{Volume of Sphere}=\frac{4}{3}\pi(\frac{6}{5})^3=\frac{288}{125}\pi$ $\displaystyle \text{Volume of Sphere}=\frac{4}{3}\pi(\frac{6}{5})^3=\frac{288}{125}\pi$

Next, recall how to find the volume of a cube:

$\text{Volume of Cube}=(\text{edge})^3$ $\displaystyle \text{Volume of Cube}=(\text{edge})^3$

Plug in the given side length to find the volume of the cube.

$\text{Volume of Cube}=5^3=125$ $\displaystyle \text{Volume of Cube}=5^3=125$

Finally, subtract the volume of the sphere from the volume of the cube.

$\text{Volume of Figure}=125-\frac{288}{125}\pi=117.76$ $\displaystyle \text{Volume of Figure}=125-\frac{288}{125}\pi=117.76$

Make sure to round to $\displaystyle 2$ places after the decimal.

Report an Error

Example Question #34 : Spheres

A sphere with a radius of $\frac{8}{3}$ $\displaystyle \frac{8}{3}$ is cut out of a cube that has a side length of $\displaystyle 6$. What is the volume of the resulting figure?

Possible Answers:

136.57 $\displaystyle 136.57$

129.05 $\displaystyle 129.05$

134.59 $\displaystyle 134.59$

122.45 $\displaystyle 122.45$

Correct answer:

136.57 $\displaystyle 136.57$

Explanation:

Since the cube is bigger, we will be subtracting the volume of the sphere from the volume of the cube.

Start by recalling how to find the volume of a sphere.

$\text{Volume of Sphere}=\frac{4}{3}\pi r^3$ $\displaystyle \text{Volume of Sphere}=\frac{4}{3}\pi r^3$

Plug in the given radius to find the volume.

$\text{Volume of Sphere}=\frac{4}{3}\pi(\frac{8}{3})^3=\frac{2048}{81}\pi$ $\displaystyle \text{Volume of Sphere}=\frac{4}{3}\pi(\frac{8}{3})^3=\frac{2048}{81}\pi$

Next, recall how to find the volume of a cube:

$\text{Volume of Cube}=(\text{edge})^3$ $\displaystyle \text{Volume of Cube}=(\text{edge})^3$

Plug in the given side length to find the volume of the cube.

$\text{Volume of Cube}=6^3=216$ $\displaystyle \text{Volume of Cube}=6^3=216$

Finally, subtract the volume of the sphere from the volume of the cube.

$\text{Volume of Figure}=216-\frac{2048}{81}\pi=136.57$ $\displaystyle \text{Volume of Figure}=216-\frac{2048}{81}\pi=136.57$

Make sure to round to $\displaystyle 2$ places after the decimal.

Report an Error

Example Question #1235 : Intermediate Geometry

A sphere with a radius of $\frac{9}{2}$ $\displaystyle \frac{9}{2}$ is cut out of a cube that has a side length of $\displaystyle 8$. What is the volume of the resulting figure?

Possible Answers:

144.17 $\displaystyle 144.17$

130.30 $\displaystyle 130.30$

156.89 $\displaystyle 156.89$

195.56 $\displaystyle 195.56$

Correct answer:

130.30 $\displaystyle 130.30$

Explanation:

Since the cube is bigger, we will be subtracting the volume of the sphere from the volume of the cube.

Start by recalling how to find the volume of a sphere.

$\text{Volume of Sphere}=\frac{4}{3}\pi r^3$ $\displaystyle \text{Volume of Sphere}=\frac{4}{3}\pi r^3$

Plug in the given radius to find the volume.

$\text{Volume of Sphere}=\frac{4}{3}\pi(\frac{9}{2})^3=\frac{243}{2}\pi$ $\displaystyle \text{Volume of Sphere}=\frac{4}{3}\pi(\frac{9}{2})^3=\frac{243}{2}\pi$

Next, recall how to find the volume of a cube:

$\text{Volume of Cube}=(\text{edge})^3$ $\displaystyle \text{Volume of Cube}=(\text{edge})^3$

Plug in the given side length to find the volume of the cube.

$\text{Volume of Cube}=8^3=512$ $\displaystyle \text{Volume of Cube}=8^3=512$

Finally, subtract the volume of the sphere from the volume of the cube.

$\text{Volume of Figure}=512-\frac{243}{2}\pi=130.30$ $\displaystyle \text{Volume of Figure}=512-\frac{243}{2}\pi=130.30$

Make sure to round to $\displaystyle 2$ places after the decimal.

Report an Error

Example Question #31 : Spheres

True or false: A sphere with radius 1 has volume $\frac{4}{3} \pi$ $\displaystyle \frac{4}{3} \pi$.

Possible Answers:

True

False

Correct answer:

True

Explanation:

Given radius $\displaystyle r$, the volume $\displaystyle V$ of a sphere can be calculated according to the formula

$V = \frac{4}{3} \pi r ^{3}$ $\displaystyle V = \frac{4}{3} \pi r ^{3}$

Set r = 1 $\displaystyle r = 1$:

$V = \frac{4}{3} \pi \cdot 1 ^{3}= \frac{4}{3} \pi \cdot 1= \frac{4}{3} \pi$ $\displaystyle V = \frac{4}{3} \pi \cdot 1 ^{3}= \frac{4}{3} \pi \cdot 1= \frac{4}{3} \pi$

The statement is true.

Report an Error

Example Question #36 : Spheres

What is the volume of a sphere with surface area 1,000 square centimeters?

Possible Answers:

$3,028 \; cm^{3}$ $\displaystyle 3,028 \; cm^{3}$

$2,974 \; cm^{3}$ $\displaystyle 2,974 \; cm^{3}$

$3,523 \; cm^{3}$ $\displaystyle 3,523 \; cm^{3}$

$3,187 \; cm^{3}$ $\displaystyle 3,187 \; cm^{3}$

$3,532 \; cm^{3}$ $\displaystyle 3,532 \; cm^{3}$

Correct answer:

$2,974 \; cm^{3}$ $\displaystyle 2,974 \; cm^{3}$

Explanation:

Use the surface area formula to find the radius, then use the volume formula to find the volume.

$A=4\pi r^{2}$ $\displaystyle A=4\pi r^{2}$

$4\pi r^{2} =1,000$ $\displaystyle 4\pi r^{2} =1,000$

$r^{2} =\frac{1,000}{4\pi }=\frac{250}{\pi }$ $\displaystyle r^{2} =\frac{1,000}{4\pi }=\frac{250}{\pi }$

$r =\sqrt{\frac{250}{\pi }}$ $\displaystyle r =\sqrt{\frac{250}{\pi }}$

$V=\frac{4}{3} \pi r^{3} = \frac{4}{3} \pi \left (\sqrt{\frac{250}{\pi }} \right )^{3} \approx 2,974$ $\displaystyle V=\frac{4}{3} \pi r^{3} = \frac{4}{3} \pi \left (\sqrt{\frac{250}{\pi }} \right )^{3} \approx 2,974$

Report an Error

Example Question #1 : How To Find The Surface Area Of A Sphere

The diameter of a sphere is $8\:m$ $\displaystyle 8\:m$. What is the sphere's surface area?

Possible Answers:

$202.3\:m^2$ $\displaystyle 202.3\:m^2$

$201.0\:m^2$ $\displaystyle 201.0\:m^2$

$201.1\:m^2$ $\displaystyle 201.1\:m^2$

$202.7\:m^2$ $\displaystyle 202.7\:m^2$

$201.6\:m^2$ $\displaystyle 201.6\:m^2$

Correct answer:

$201.1\:m^2$ $\displaystyle 201.1\:m^2$

Explanation:

The equation to find the surface area of a sphere is: $Area = 4 \cdot \pi \cdot r^2$ $\displaystyle Area = 4 \cdot \pi \cdot r^2$

The only information required to solve for the area is the radius. This information is given to us in a sense. If the diameter of the sphere is $8\:m$ $\displaystyle 8\:m$, the radius must be $4\:m$ $\displaystyle 4\:m$. This is because the radius is equivalent to half of the diameter.

Now that we know the radius, we can solve for the area by substituting in $4\:m$ $\displaystyle 4\:m$ for the value of $\displaystyle r$, the radius.

$Area = 4 \cdot \pi \cdot (4^2)$ $\displaystyle Area = 4 \cdot \pi \cdot (4^2)$

$A = 4 \cdot \pi \cdot 16$ $\displaystyle A = 4 \cdot \pi \cdot 16$

$A = 64 \cdot \pi$ $\displaystyle A = 64 \cdot \pi$

$\displaystyle A = 201.062 m^2$

Report an Error

Example Question #271 : Solid Geometry

If the radius of a sphere is $1\:unit$ $\displaystyle 1\:unit$, what is the sphere's surface area?

Possible Answers:

$8\pi\:units$ $\displaystyle 8\pi\:units$

$4\pi\:units$ $\displaystyle 4\pi\:units$

$2\pi\:units$ $\displaystyle 2\pi\:units$

$\frac{16}{9}\pi\:units$ $\displaystyle \frac{16}{9}\pi\:units$

$\frac{4}{3}\pi\:units$ $\displaystyle \frac{4}{3}\pi\:units$

Correct answer:

$4\pi\:units$ $\displaystyle 4\pi\:units$

Explanation:

Write the formula for surface area of a sphere:

$A=4\pi r^2$ $\displaystyle A=4\pi r^2$

Plug in the value of radius and solve for the surface area:

$A=4\pi (1)^2 = 4\pi$ $\displaystyle A=4\pi (1)^2 = 4\pi$

Report an Error

Example Question #3 : How To Find The Surface Area Of A Sphere

The diameter of a sphere is $18\:cm$ $\displaystyle 18\:cm$. What is its surface area?

Possible Answers:

$1017.3\:cm^2$ $\displaystyle 1017.3\:cm^2$

$1017.9\:cm^2$ $\displaystyle 1017.9\:cm^2$

$972.0\:cm^2$ $\displaystyle 972.0\:cm^2$

$1017.8\:cm^2$ $\displaystyle 1017.8\:cm^2$

$1017.4\:cm^2$ $\displaystyle 1017.4\:cm^2$

Correct answer:

$1017.9\:cm^2$ $\displaystyle 1017.9\:cm^2$

Explanation:

The surface area of a sphere is given by the equation: $SA=4\pi r^2$ $\displaystyle SA=4\pi r^2$

The only given information is the diameter: $18\:cm$ $\displaystyle 18\:cm$. In order to solve for the surface area, the only necessary information is the radius. The missing variable $\displaystyle r$ (radius) can be calculated through the given diameter because diameter is twice the length of the radius. That is: d= 2(r) $\displaystyle d= 2(r)$

Using that information, radius can be solved for by
18=2(r) $\displaystyle 18=2(r)$
$(\frac{18\:cm}{2})=r$ $\displaystyle (\frac{18\:cm}{2})=r$
$9\:cm=r$ $\displaystyle 9\:cm=r$

Given that the radius is $9\:cm$ $\displaystyle 9\:cm$, this value can be substituted in to solve for the final surface area:

$SA= 4 \pi (9\:cm)^2$ $\displaystyle SA= 4 \pi (9\:cm)^2$

$SA= 4(81\:cm^2)\pi$ $\displaystyle SA= 4(81\:cm^2)\pi$

$SA= 324\pi\:cm^2$ $\displaystyle SA= 324\pi\:cm^2$

$SA=1017.88\:cm^2$ $\displaystyle SA=1017.88\:cm^2$

Report an Error

Example Question #4 : How To Find The Surface Area Of A Sphere

Find the surface area of a sphere with a circumference of $\small \small 10\pi\,cm$ $\displaystyle \small \small 10\pi\,cm$.

Possible Answers:

$\small \small 100\pi\,cm^2$ $\displaystyle \small \small 100\pi\,cm^2$

$\small 200\pi\,cm^2$ $\displaystyle \small 200\pi\,cm^2$

$\small \small 400\pi\,cm^2$ $\displaystyle \small \small 400\pi\,cm^2$

$\small \small 20\pi\,cm^2$ $\displaystyle \small \small 20\pi\,cm^2$

$\small \small 25\pi\,cm^2$ $\displaystyle \small \small 25\pi\,cm^2$

Correct answer:

$\small \small 100\pi\,cm^2$ $\displaystyle \small \small 100\pi\,cm^2$

Explanation:

The formula for the surface area of a sphere is simply

$\small A=4\pi r^2$ $\displaystyle \small A=4\pi r^2$

However, the problem is that we are given the circumference rather than the radius. Nonetheless, this isn't too big of an obstacle, as the formula for circumference in terms of radius is simply.

$\small C=2\pi r$ $\displaystyle \small C=2\pi r$

Substituting or value gives

$\small 10\pi=2\pi r$ $\displaystyle \small 10\pi=2\pi r$

Solving for the radius gives

$\small r=5$ $\displaystyle \small r=5$

We can then substitute this value into our formula for surface area.

$\small A=4\pi(5^2)$ $\displaystyle \small A=4\pi(5^2)$

$\small A=100\pi$ $\displaystyle \small A=100\pi$

Therefore, our surface area is $\small 100\pi\,cm^2$ $\displaystyle \small 100\pi\,cm^2$

Report an Error

Example Question #271 : Solid Geometry

If the radius of a sphere is $\displaystyle 15$, what is the surface area of the sphere?

Possible Answers:

$225\pi$ $\displaystyle 225\pi$

$300\pi$ $\displaystyle 300\pi$

$675\pi$ $\displaystyle 675\pi$

$13500\pi$ $\displaystyle 13500\pi$

$900\pi$ $\displaystyle 900\pi$

Correct answer:

$900\pi$ $\displaystyle 900\pi$

Explanation:

Write the equation for the surface area of a sphere.

$A=4\pi r^2$ $\displaystyle A=4\pi r^2$

Substitute the radius and find the area.

$A=4\pi (15)^2 = 4\pi(225)=900\pi$ $\displaystyle A=4\pi (15)^2 = 4\pi(225)=900\pi$

Report an Error

View Tutors

Aiay
Certified Tutor

The University of Texas at Austin, Bachelor of Engineering, Electrical Engineering.

View Tutors

Tracy
Certified Tutor

Florida Memorial University, Bachelor in Arts, English.

View Tutors

Noah
Certified Tutor

Bard College, Bachelors, Philosophy and Creative Writing (Dual Major). Sarah Lawrence College, Master of Fine Arts, Creative ...

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Computer Science Tutors in Los Angeles, English Tutors in San Diego, English Tutors in Denver, MCAT Tutors in Los Angeles, Statistics Tutors in Boston, French Tutors in Chicago, ACT Tutors in San Francisco-Bay Area, Spanish Tutors in Dallas Fort Worth, Physics Tutors in Houston, Biology Tutors in Chicago

Popular Courses & Classes

SAT Courses & Classes in Houston, SSAT Courses & Classes in Los Angeles, GRE Courses & Classes in Seattle, SSAT Courses & Classes in Seattle, Spanish Courses & Classes in Boston, ISEE Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in New York City, SAT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Houston, ACT Courses & Classes in San Francisco-Bay Area

Popular Test Prep

SSAT Test Prep in Miami, ISEE Test Prep in Atlanta, GRE Test Prep in Houston, GMAT Test Prep in Houston, SAT Test Prep in Seattle, GMAT Test Prep in Philadelphia, ACT Test Prep in San Francisco-Bay Area, SAT Test Prep in Washington DC, LSAT Test Prep in New York City, ACT Test Prep in Atlanta

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Intermediate Geometry : Solid Geometry

Study concepts, example questions & explanations for Intermediate Geometry

All Intermediate Geometry Resources

Example Questions

Example Question #33 : Spheres

Example Question #34 : Spheres

Example Question #1235 : Intermediate Geometry

Example Question #31 : Spheres

Example Question #36 : Spheres

Example Question #1 : How To Find The Surface Area Of A Sphere

Example Question #271 : Solid Geometry

Example Question #3 : How To Find The Surface Area Of A Sphere

Example Question #4 : How To Find The Surface Area Of A Sphere

Example Question #271 : Solid Geometry

All Intermediate Geometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: