All Intermediate Geometry Resources
Example Questions
Example Question #1 : How To Find The Surface Area Of A Cube
One side of a cube is 
To find the surface area of a cube, we find the area of a face by multiplying two of its sides together; then, we multiply by 


We know that for this problem, 
Example Question #1 : How To Find The Surface Area Of A Cube
The volume of a cube is 
What is the surface area of the cube?






Using the volume given, we take it's cube to find the length of the cube: 
Therefore, the length of the cube is 8 cm.
Knowing the properties of a cube, this implies that the width and height of the cube is also 8 cm.
Since all sides are identical, the formula for the surface area is length times width times the number of sides: 
Example Question #2 : How To Find The Surface Area Of A Cube
If a side of a cube has a length of 
Write the formula to find the surface of a cube, where 
Substitute and solve.
Example Question #1 : How To Find The Surface Area Of A Cube
Find the surface area of a cube with a side length of 
Write the formula for the surface area of a cube, substitute the length provided in the question, and simplify.
Example Question #32 : Solid Geometry
if the side length of a cube is 
The formula for the surface area of a cube is:


We are given the length of one side of the cube in question, so we can substitute that value into the surface area equation and solve:
Example Question #1 : How To Find The Surface Area Of A Cube
A cube has a sphere inscribed inside it with a diameter of 4 meters. What is the surface area of the cube?
None of these
Since the sphere is inscribed within the cube, its diameter is the same length as an edge of the cube. Since cubes have identical side lengths we find the area of one side and then multiply by the number of sides to find the total surface area.
Area of one side:
Total surface area:
Example Question #2 : How To Find The Surface Area Of A Cube
A geometric cube has a volume of 
We first need to know the edge length before we can solve for surface area. Since we are provided the volume and all edges are of equal length, we can use the formula for volume to get the length of sides:
Now that we know the length of sides, we can plug this value into our surface area formula:
Certified Tutor
Certified Tutor
All Intermediate Geometry Resources

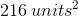








































![\\volume=length\cdot width\cdot height\\ \\27\;cm^3=a^3\\ \\a=\sqrt[3]{27\;cm^3}\\ \\a=3\;cm](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/751208/gif.latex)




