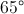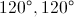HSPT Math : HSPT Mathematics
Study concepts, example questions & explanations for HSPT Math
All HSPT Math Resources
Example Questions
Example Question #2001 : Hspt Mathematics
What is the measure of a right angle?
The measure of a right angle is 
Example Question #1405 : Concepts
Figure NOT drawn to scale.
If 
More information is needed to solve the problem.
The measure of an exterior angle of a triangle is the sum of the measures of its remote interior angles, so
Example Question #106 : Properties Of Triangles
Figure NOT drawn to scale.
If 
The measure of an exterior angle of a triangle is the sum of the measures of its remote interior angles, so
Example Question #2002 : Hspt Mathematics
If the vertex angle of an isoceles triangle is 
In an isosceles triangle, the base angles are the same. Also, the three angles of a triangle add up to 
So, subtract the vertex angle from 
Because there are two base angles you divide 
Example Question #2003 : Hspt Mathematics
Note: Figure NOT drawn to scale.
Refer to the above diagram.
Which of the following could be a measure of 
All of the other choices give a possible measure of 
The measure of an exterior angle of a triangle is the sum of the measures of its remote interior angles, so
We also have the following constraints:
Then, by the addition property of inequalities,
Therefore, the measure of 
Example Question #109 : Properties Of Triangles
Refer to the above diagram.
Which of the following could be a measure of 
All of the other responses are correct.
All of the other responses are correct.
The measure of an exterior angle of a triangle is the sum of the measures of its remote interior angles, so
or
Therefore, the maximum value of 
The minimum value of 
Therefore,
Since all of the choices fall in this range, all are possible measures of 
Example Question #1411 : Concepts
One angle of a right triangle has measure 
This triangle cannot exist.
This triangle cannot exist.
A right triangle must have one right angle and two acute angles; this means that no angle of a right triangle can be obtuse. But since 
Example Question #2 : How To Find An Angle In A Right Triangle
One angle of a right triangle has measure 
This triangle cannot exist.
One of the angles of a right triangle is by definition a right, or 
The other two angles measure 
Example Question #2004 : Hspt Mathematics
AB and CD are two parrellel lines intersected by line EF. If the measure of angle 1 is 
The angles are equal. When two parallel lines are intersected by a transversal, the corresponding angles have the same measure.
Example Question #2 : How To Find An Angle Of A Line
Lines A and B in the diagram below are parallel. The triangle at the bottom of the figure is an isosceles triangle.
What is the degree measure of angle 
Since A and B are parallel, and the triangle is isosceles, we can use the supplementary rule for the two angles, 
All HSPT Math Resources
