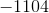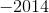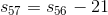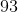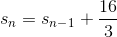For this problem, you definitely do not want to "count upwards" to the full value of the sequence.  Therefore, the best approach is to consider the general pattern that arises from the general definition:

This means that for every element in the list, each one is  less than the one preceding it.  For instance:

Now, notice that the first element is:

The second is:

The third could be represented as:

And so forth...

Now, notice that for the third element, there are only two instances of .  We could rewrite our sequence:

This value will always "lag behind" by one.  Therefore, for the th element, you will have:

For this sequence, you do not have a starting point (i.e. 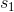); however, you are able to interpret it relatively easily. The sequence is merely one in which each number is twenty larger than the one preceding it. Therefore, if  were , you would have:

Now, to find difference between the 20th and the 30th element, it is merely necessary to count the number of twenties that would be added for each of those elements. For instance, the difference between the 21st and the 20th elements is . Thus, since you would add a total of ten twenties from the 20th to the 30th element, you know that the difference between these two values is .

Since this sequence is linear, we know that it will add the same amount for each element. This means that you can evenly divide the difference between the first and the ninth term. Be careful! There will be eight total increases between these terms. (Think this through: 1 to 2, 2 to 3, 3 to 4, etc.)

Thus, we know that the total difference between these terms is:

Now, dividing this among the eight increases that happen, we know:

This means that for each element, we add  to the one prior to it. This means that our general sequence is defined as:

First we need to find out how many possible numbers there are. The number of possible four-digit numbers with four different digits is simply 4 * 4 * 4 * 4 = 256.  

To find the sum, the formula we must remember is sum = average * number of values. The last piece that's missing in this formula is the average. To find this, we can average the first and last number, since the numbers are consecutive. The smallest number that can be created from 1, 2, 3, and 4 is 1111, and the largest number possible is 4444. Then the average is (1111 + 4444)/2.

So sum = 5555/2 * 256 = 711,040.

For each quantity, only count the integers that aren't in the other quantity. Both quantities include the numbers 51 to 98, so those numbers won't affect which is greater. Only Quantity A has 49 and 50 (for a total of 99) and only Quantity B has 99. Since the excluded numbers from both quantities equal 99, you can conclude that the 2 quantities are equal.

The formula here is sum = average value * number of values.  Since this is a consecutive series, the average can be found by averaging only the first and last terms: (1 + 69)/2 = 35.  

sum = average * number of values = 35 * 69 = 2415

The sum of all integers from 1 to 30 can be found using the formula

, where  is 30. In this case, the sum equals 465.

We can represent our numbers as:

 will have to be an odd number since the whole sequence is odd.  However, this will work out when we do the math.  Now, we know that all of these added up will be .  We have  and the sum of the set , the sum of which is .  

Thus, we know:

Solve for :

Therefore, the fourth element will be :

There are two ways to figure out this list of integers.  On the one hand, you might know that the average of a set of consecutive integers is the "middle value" of that set.  So, if the average is  and the size , the list must be:

Another way to figure this out is to represent your integers as:

The average of these values will be all of these numbers added together and then divided by .  This gives us:

Multiply both sides by :

Finish solving:

This means that the largest value is:

The sum of 4 consecutive numbers being 38 can be written as the following equation.

We can simplify this to solve for x.

This tells us that the smallest number is 8 and the largest is (8 + 3) = 11. From this, we can find their mean.