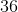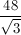Two sides of a triangle must add up to greater than the third side. 25, 37, 66 cannot be the lengths of the sides of a triangle as 25 + 37 < 66. 

If two sides of a triangle are 6 and 12, the third must be greater than 12-6 and less than 12+6 since two sides cannot be summed to be greater than the third side in a triangle.  There are 11 possible values for x: 7, 8, 9, 10,11, 12, 13, 14, 15, 16, 17. 

12: The sum of two sides of a triangle must be greater than the third side. Therefore, the length of the third side would have to be less than 12 and greater than 2. 

For a triangle where the length of two sides,  and , is the only information known, the third side, , is limited in the following matter:

For the triangle given:

.

Both choices A and B satisfy this criteria.

If two sides of a triangle are known and all angles are unknown, the length of the third side is limited by the difference and sum of the other two sides.

The missing side must be greater than .

Quantity A is greater.

Seeing the sides  and  may bring to mind a  triangle. However, we've been told nothing about the angles of the triangle. It could be right, or it could be obtuse or acute.

Since the angles are unknown, the side is bounded as follows:

There are plenty of potential lengths that fall above and below . The relationship cannot be determined.

If two sides of a triangle are known and the angles of the triangle are unknown, the length of the missing side is limited by the difference and sum of the other two sides.

For a triangle with sides  and , there is no way a side could be .

Quantity B is greater.

Perimeter is found by adding up all sides of the triangle. All sides in an equilateral triangle are equal, so we need to find the value of just one side to know the values of all sides. 

The height of an equilateral triangle divides it into two equal 30:60:90 triangles, which will have side ratios of 1:2:√3. The height here is the √3 ratio, which in this case is equivalent to 8, so to get the length of the other two sides, we put 8 over √3 (8/√3) and 2 * 8/√3 = 16/√3, which is the hypotenuse of our 30:60:90 triangle.

The perimeter is then 3 * 16/√3, or 48/√3.

By having a height in an equilateral triangle, the angle is bisected therefore creating two  triangles.

The height is opposite the angle . We can set-up a proportion.

Side opposite  is  and the side of equilateral triangle which is opposite  is .

 Cross multiply.

 Divide both sides by 

 Multiply top and bottom by  to get rid of the radical.

Since each side is the same and there are three sides, we just multply the answer by three to get . 

The area of an equilateral triangle is .

So let's set-up an equation to solve for . 

 Cross multiply.

The  cancels out and we get .

Then take square root on both sides and we get . Since we have three equal sides, we just multply  by three to get  as the final answer.