All GRE Math Resources
Example Questions
Example Question #1 : How To Find The Length Of The Diagonal Of A Square
If a square has a side length of 4, how long is the diagonal of the square?
4√2
2√2
8√2
4
16
4√2
A diagonal divides a square into two 45-45-90 triangles, which have lengths adhering to the ratio of x: x: x√2. Therefore, 4√2 is the correct answer as the diagonal represents the hypotenuse of the triangle. the Pythagorean theorem can also be used: 42+ 42 = c2.
Example Question #2 : How To Find The Length Of The Diagonal Of A Square
If a square has a side length of √2, how long is the diagonal of the square?
2
4√2
4
4-√2
2√2
2
A diagonal divides a square into two 45-45-90 triangles, which have lengths adhering to the ratio of x: x: x√2. Therefore, 2 is the correct answer as the diagonal represents the hypotenuse of the triangle. the Pythagorean theorem can also be used: √22+ √22 = c2.
Example Question #3 : How To Find The Length Of The Diagonal Of A Square
A square has a side of length 5. What is the length of its diagonal?
5√3
5
10
10√3
5√2
5√2
The diagonal separates the square into two 45-45-90 right triangles. The problem can be solved by using the Pythagorean Theorem, a2 + b2 = c2. It can also be solved by recognizing the 45-45-90 special triangles, which have side ratios of x : x : x√2.
Example Question #1 : How To Find The Length Of The Diagonal Of A Square
A square with width of 
We know that each side of the square is 6, so use the Pythagorean Theorem to solve for the diagonal of the square. The diagonal of the square is also the diameter of the circle.
Therefore the radius must be
Now let's find the area inside the circle using the radius.

Example Question #2 : How To Find The Length Of The Diagonal Of A Square
Quantity A:
The diagonal of a square with a side-length of 
Quantity B:
The side-length of a square with a diagonal of 
The relationship between the two quantities cannot be determined from the information given.
Quantity A is greater.
Both quantities are equal.
Quantity B is greater.
Both quantities are equal.
Quantity A: The diagonal of a square with a side-length of 7.
Quantity B: The side-length of a square with a diagonal of 14.
Both quantities can be determined, so can the relationship.
Quantity A:
To determine the diagonal of a square, it's important to remember that a diagonal directly bisects a square from corner to corner. In other words, it bisects the corners, creating two triangles with 45:45:90 proportions, with the diagonal serving as the hypotenuses . If you remember your special triangles, then the side-side-hypotenuse measurements have a ratio of 
In Quantity A, the side-length is 7. Following the proportion:
The diagonal equals 
Quantity B:
We can use the same ratio to figure out quantity B by substituting x for the unknown side-length quantity, which looks like this:
To find x in this ratio, just isolate x in the hypotenuse:
Divide by
Now, how does Quantity A and Quantity B match up?
On the surface, it looks like the two quanties are equal. But how do we prove it? Well, we know that 
Divide both sides by
Therefore, both quantities are equal.
Example Question #181 : Plane Geometry
Quantity A:
The side-length of a square with a perimeter of 
Quantity B:
The side-length of a square with an area of 
The relationship cannot be determined from the information given
Both quantities are equal
Quantity A is greater
Quantity B is greater
Quantity A is greater
The first step to a quantitative comparison is to determine whether it can be solved at all with the given knowledge. Since all you need to find the side-length of a square is the perimeter, the area, OR the diagonal and we have one of each for these two quantities, this relationship can be determined. Thus, "the relationship cannot be determined" is out.
Now, to solve both quantities. Quantity A can be solved by translating the perimeter into side lengths: the formula for the perimeter of a square is 

Thus, quantity A is 
Quantity B can be solved by translating the area into side lengths: the formula for the area of a square is 


Thus, quantity B is roughly 
Therefore quantity A is greater.
Example Question #182 : Plane Geometry

Circle 

If the area of Circle 


If the area of Circle 



Simplifying, we get:
We know that 





Notice that the diameter is the same length as a side of the square. Thus, 

Example Question #183 : Plane Geometry

Circle 

The line segment marked with length 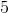

What is the length of side 
This cannot be computed from the given data
You can further fill in your diagram as follows:

Now, we know that the triangle 




For our data, that means:
Simplifying, we know:
Now, make both sides reciprocals:
Finally, solve:
Recall, this is both the length of the side and the diameter of the circle. Hence, you have your answer.
Example Question #1 : How To Find The Equation Of Parallel Lines
What is one possible equation for a line parallel to the one passing through the points (4,2) and (15,-4)?
y = -11/6x + 8.32
y = -6/11x + 57.4
y = 11/6x + 88
y = 15x + 12
y = 6/11x - 33
y = -6/11x + 57.4
(4,2) and (15,-4)
All that we really need to ascertain is the slope of our line. So long as a given answer has this slope, it will not matter what its y-intercept is (given the openness of our question). To find the slope, use the formula: m = rise / run = (y1 - y2) / (x1 - x2):
(2 - (-4)) / (4 - 15) = (2 + 4) / -11 = -6/11
Given this slope, our answer is: y = -6/11x + 57.4
Example Question #1 : Coordinate Geometry
Lines m and n are parallel
What is the value of angle 
115
180
130
145
125
145
By using the complementary and supplementary rules of geometry (due to lines m and n being parallel), as well as the fact that the sum of all angles within a triangle is 180, we can carry through the operations through stepwise subtraction of 180.
x = 125 → angle directly below also = 125. Since a line is 180 degrees, 180 – 125 = 55. Since right triangle, 90 + 55 = 145 → rightmost angle of triangle 180 – 145 = 35 which is equal to the reflected angle. Use supplementary rule again for 180 – 35 = 145 = y.
Once can also recognize that both a straight line and triangle must sum up to 180 degrees to skip the last step.
All GRE Math Resources




















































