GRE Math : Inequalities

Study concepts, example questions & explanations for GRE Math

Create An Account

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Find The Solution To An Inequality With Subtraction

Find the slope of the inequality equation $y-7< x+2y-14$

Possible Answers:

–1

–7

Correct answer:

–1

Explanation:

The answer is:

$y-7< x+2y-14$

$-y-7< x-14$

$-1(-y< x-7)$

$y > -x+7$

From the equation we can see that the slope is –1.

Report an Error

Example Question #11 : Inequalities

and are both integers.

If y-3x>21 , y>1 , and y<4 , which of the following is a possible value of ?

Possible Answers:

Correct answer:

Explanation:

Take the values of y that are possible, i.e. 2 and 3, and plug them into the first inequality. First, plug in 2. 2 – 3x > 21. Subtract 2 from both sides, and then divide by –3. Don't forget that when you divide or multiply by a negative number in an inequality you must flip the inequality sign. Thus, x < –19/3. Now plug in 3. We find, following the same steps, that when y=3, x < –6. Thus –7 is the correct answer.

Report an Error

Example Question #213 : Gre Quantitative Reasoning

Quantity A:

The value(s) for which the following function is undefined:

$f(x)=\sqrt{5x-135}$

Quantity B:

Which of the following is true?

Possible Answers:

The two quantities are equal.

Quantity A is larger.

A comparison cannot be detemined from the given information.

Quantity B is larger.

Correct answer:

Quantity B is larger.

Explanation:

This question is not as hard as it seems. Remember that for real numbers, square roots cannot be taken of negative numbers. Therefore, we know that this function is undefined for:

5x-135 < 0

This is simple to solve. Merely add 135 to both sides:

5x<135

Then, divide by :

x < 27

Therefore, quantity A is less than quantity B. This means that quantity B is greater than it.

Report an Error

Example Question #214 : Gre Quantitative Reasoning

|x+12|<42

Quantity A:

Quantity B:

Which of the following is true?

Possible Answers:

Quantity A is larger.

Quantity B is larger.

A comparison cannot be detemined from the given information.

The two quantities are equal.

Correct answer:

A comparison cannot be detemined from the given information.

Explanation:

Recall that when you have an absolute value and an inequality like

|x+12|<42 ,

this is the same as saying that x+12 must be between and . You can rewrite it:

-42 < x+12<42

To solve this, you merely need to subtract from all three values:

-54 < x <30

Since is between and , it could be both larger or smaller than . Therefore, you cannot determine the relationship based on the given information.

Report an Error

Example Question #12 : Inequalities

Quantitative Comparison

x > 0

Column A:

Column B: $\frac{5}{x}$

Possible Answers:

The relationship cannot be determined from the information provided.

Quantity B is greater.

Quantity A is greater.

The quantities are equal.

Correct answer:

The relationship cannot be determined from the information provided.

Explanation:

For quantitative comparison questions involving a shared variable between quantities, the best approach is to test a positive integer, a negative integer, and a fraction. Half of our work is eliminated, however, because the question stipulates that x > 0. We only need to check a positive integer and a positive fraction between 0 and 1. Plugging in 2, we see that quantity A is greater than quantity B. Checking 1/2, however, we find that quantity B is greater than quantity A. Thus the relationship cannot be determined.

Report an Error

Example Question #221 : Gre Quantitative Reasoning

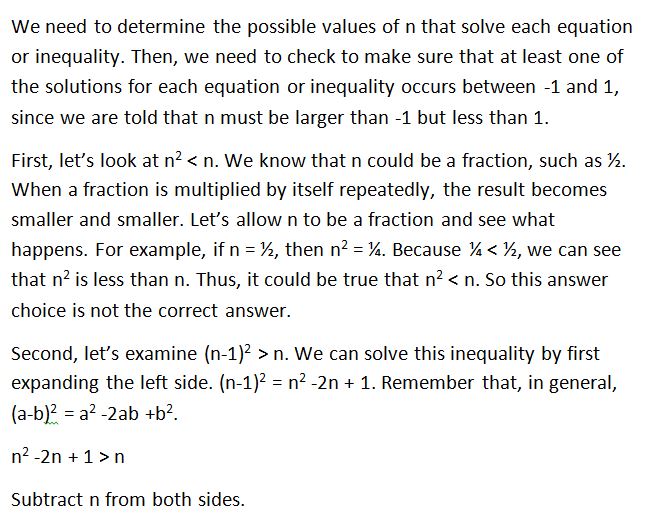
If –1 < n < 1, all of the following could be true EXCEPT:

Possible Answers:

16n² - 1 = 0

n² < n

n² < 2n

|n² - 1| > 1

(n-1)² > n

Correct answer:

|n² - 1| > 1

Explanation:

N_part_1

N_part_2

N_part_3

N_part_4

N_part_5

Report an Error

Example Question #222 : Gre Quantitative Reasoning

(√(8) / -x ) < 2. Which of the following values could be x?

Possible Answers:

All of the answers choices are valid.

-2

-1

-4

-3

Correct answer:

-1

Explanation:

The equation simplifies to x > -1.41. -1 is the answer.

Report an Error

Example Question #223 : Gre Quantitative Reasoning

Solve for x

$\small 3x+7 \geq -2x+4$

Possible Answers:

$\small x \geq \frac{3}{5}$

$\small x \leq -\frac{3}{5}$

$\small x \leq \frac{3}{5}$

$\small x \geq -\frac{3}{5}$

Correct answer:

$\small x \geq -\frac{3}{5}$

Explanation:

$\small 3x+7 \geq -2x+4$

$\small 3x \geq -2x-3$

$\small 5x \geq -3$

$\small x\geq -\frac{3}{5}$

Report an Error

Example Question #181 : Equations / Inequalities

We have x^2-4<0 , find the solution set for this inequality.

Possible Answers:

x=0

x>-2

x<2

x>2, x<-2

-2<x<2

Correct answer:

-2<x<2

Explanation:

$x^2-4<0 \Rightarrow x^2<4 \Rightarrow -2<x<2$

Report an Error

Example Question #1 : How To Find The Solution To An Inequality With Multiplication

Fill in the circle with either $<$ , $>$ , or $=$ symbols:

$(x-3)\circ\frac{x^2-9}{x+3}$ for $x\geq 3$ .

Possible Answers:

None of the other answers are correct.

The rational expression is undefined.

$(x-3)=\frac{x^2-9}{x+3}$

$(x-3)> \frac{x^2-9}{x+3}$

$(x-3)< \frac{x^2-9}{x+3}$

Correct answer:

$(x-3)=\frac{x^2-9}{x+3}$

Explanation:

$(x-3)\circ\frac{x^2-9}{x+3}$

Let us simplify the second expression. We know that:

$(x^2-9)=(x+3)(x-3)$

So we can cancel out as follows:

$\frac{x^2-9}{x+3}=\frac{(x+3)(x-3)}{(x+3)}=x-3$

$(x-3)=\frac{x^2-9}{x+3}$

Report an Error

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Chemistry Tutors in Boston, LSAT Tutors in Philadelphia, Algebra Tutors in Chicago, Reading Tutors in Atlanta, SAT Tutors in Atlanta, Physics Tutors in Boston, Chemistry Tutors in New York City, Reading Tutors in San Diego, MCAT Tutors in Philadelphia, MCAT Tutors in Denver

Popular Courses & Classes

SAT Courses & Classes in Phoenix, GRE Courses & Classes in Phoenix, GRE Courses & Classes in Dallas Fort Worth, LSAT Courses & Classes in San Diego, LSAT Courses & Classes in Chicago, GMAT Courses & Classes in Boston, SSAT Courses & Classes in Houston, GRE Courses & Classes in New York City, MCAT Courses & Classes in Washington DC, SAT Courses & Classes in New York City

Popular Test Prep

ISEE Test Prep in Phoenix, SSAT Test Prep in Philadelphia, SAT Test Prep in Miami, ACT Test Prep in Los Angeles, GRE Test Prep in Chicago, SSAT Test Prep in Washington DC, GMAT Test Prep in Chicago, ISEE Test Prep in San Diego, ACT Test Prep in San Diego, GMAT Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

Email address:
Your name:
Feedback:

GRE Math : Inequalities

Study concepts, example questions & explanations for GRE Math

All GRE Math Resources

Example Questions

Example Question #1 : How To Find The Solution To An Inequality With Subtraction

Example Question #11 : Inequalities

Example Question #213 : Gre Quantitative Reasoning

Example Question #214 : Gre Quantitative Reasoning

Example Question #12 : Inequalities

Example Question #221 : Gre Quantitative Reasoning

Example Question #222 : Gre Quantitative Reasoning

Example Question #223 : Gre Quantitative Reasoning

Example Question #181 : Equations / Inequalities

Example Question #1 : How To Find The Solution To An Inequality With Multiplication

All GRE Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details