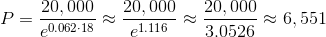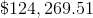College Algebra : Applications
Study concepts, example questions & explanations for College Algebra
All College Algebra Resources
Example Questions
Example Question #1 : Applications
On the day of a child's birth, a sum of money is to be invested into a certificate of deposit (CD) that draws 
If the amount of money invested is to be a multiple of 
If we let 
Substitute 
The minimum principal to be invested initially is $6,551. However, since we are looking for the multiple of $1,000 that guarantees a minimum final balance of $20,000, we round up to the nearest such multiple, which is $7,000 - the correct response.
Example Question #2 : Applications
Twelve years ago, your grandma put money into a savings account for you that earns 
$21,170
$8,103
$24,596
$10,778
$81,030
$24,596
1. Use 
2. Solve for
You currently have $24,596 in your account.
Example Question #77 : Exponential And Logarithmic Functions
Jeffrey has won a lottery and has elected to take a $10,000 per month payment.
At the beginning of the year, Jeffrey deposits the first payment of $10,000 in an account that pays 7.6% interest annually, compounded continuously. At the very beginning of each month, he deposits another $10,000. How much will he have at the very end of the year?
The continuous compound interest formula is
where 
We can go ahead and calculate 
The formula can be rewritten as
At the beginning of January, Jeffrey deposits $10,000. At the end of January, there is
in the account.
At the beginning of February, he again deposits $10,000, so there is now
in the account.
At the end of February, there is
in the account.
Repeat addition of $10,000, then multiplication by 1.006353, ten more times to get the amount of money in the account at the end of December. This will be $125,072.98.
Example Question #81 : Exponential And Logarithmic Functions
Sheila has won a lottery and has elected to take a $10,000 per month payment.
At the beginning of the year, Sheila deposits the first payment of $10,000 in an account that pays 7.6% interest annually, compounded monthly. At the very beginning of each month, she deposits another $10,000. How much will she have at the very end of the year?
The periodic compound interest formula is
where 
Since Sheila deposits $1,000 per month, we apply this formula twelve times, with 
We can go ahead and calculate 
The formula can be rewritten as
At the beginning of January, Sheila deposits $10,000. At the end of January, there is
in the account.
At the beginning of February, she again deposits $10,000, so there is now
in the account.
At the end of February, there is
in the account.
Repeat addition of $10,000, then multiplication by 1.006333, ten more times to get the amount of money in the account at the end of December. This will be $125.056.56
Example Question #3 : Applications
An investor places $5,000 into an account that has a 5% interest rate. If the he keeps his money in the account for 57 months, and it is compounded quarterly, how much interest will he earn?
Round your answer to the nearest dollar, if needed
Use the formula for compound interest to solve:
"A" is the amount of interest, "P" is the initial amount invested, "r" is the interest rate, "m" is the number of times per year the interest is compounded, and "t" is the number of years the money is invested.
Plug in the appropriate values for the equation:
Because "t" is measure in years, 57 months needs to be converted to years (i.e. 57 months=4.75 years) to solve this equation
Simplify the equation:
Solution:
All College Algebra Resources
