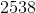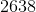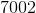Basic Arithmetic : Whole Numbers
Study concepts, example questions & explanations for Basic Arithmetic
All Basic Arithmetic Resources
Example Questions
Example Question #11 : Operations With Whole Numbers
For the following questions, please select the answer that most correctly completes the equation.
Ones digit:
Tens digit: 
Hundreds digit: 
Thousands digit:
Example Question #11 : Operations With Whole Numbers
Paul had 
This is a simple subtraction problem.
First, subtract the number of video games Paul gave to Chris on Monday.
Now, subtract the number of video games Paul sold from the number he has left.
Finally, subtract the number of video games Paul broke and threw away.
Example Question #12 : Operations With Whole Numbers
What is the solution of the above equation?
We can show this using objects:
We can see that only 7 objects are left.
Example Question #11 : Whole Numbers
What is the solution of the above equation?
We can see that by the objects.
We can see that there is only one object left.
Example Question #4 : Subtraction With Whole Numbers
Timmy has 
The problem can be solved as follows. First, calculate the number of sea monkeys left at the end of the first month:
Then, calculate the number of sea monkeys left at the end of the second month:
Example Question #16 : Operations With Whole Numbers
What is 
If you need to break it down, you can split it into smaller pieces:
Example Question #15 : Operations With Whole Numbers
Solve for 
To solve for n we need to isolate n on one side of the equation and all of the constants on the other side.
We do this by adding 15 to both sides.
Then we add 12 to both sides to get our final answer.
Example Question #14 : Operations With Whole Numbers
A city bus has five stops left on its route. At the first stop, 
To solve, create a linear equation:
We then subtract the amount of people that got off at each stop and set that equal to the number of people to get off at the final stop.
Example Question #15 : Operations With Whole Numbers
What is the solution of the above equation?
We can see this by implementing objects.
^ We can see that there are 21 objects.
Example Question #18 : Operations With Whole Numbers
Please choose the best answer for the question below.
What is
Use a calculator to check your work if allowed, but if not, then I'd reccommend the following method, breaking the problem down into smaller pieces.
And then add your results:
All Basic Arithmetic Resources
