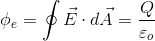Call Now to Set Up Tutoring:

(888) 888-0446

AP Physics C Electricity : Electricity

Study concepts, example questions & explanations for AP Physics C Electricity

Create An Account

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #11 : Electricity And Magnetism Exam

Two capacitors are in parallel, with capacitance values of 6mF and 10mF . What is their equivalent capacitance?

Possible Answers:

12mF

4mF

16mF

2.67mF

Correct answer:

16mF

Explanation:

The equivalent capacitance for capacitors in parallel is the sum of the individual capacitance values.

$C_{eq}=C_1+C_2$

Using the values given in the question, we can find the equivalent capacitance.

$C_{eq}=10mF+6mF=16mF$

Report an Error

Example Question #2 : Using Coulomb's Law

A proton moves in a straight line for a distance of . Along this path, the electric field is uniform with a value of $2*10^7 \frac{V}{m}$ . Find the force on the proton.

The charge of a proton is $1.61*10^{-19}\text{C}$ .

Possible Answers:

$1.61*10^{-12}N$

$6.44*10^{-12}N$

$3.22*10^{-12}N$

$0.8*10^{-12}N$

Correct answer:

$3.22*10^{-12}N$

Explanation:

The force of an electric field is given by the equation F=qE , where is the charge of the particle and is the electric field strength. We can use the given values from the question to solve for the force.

$F=qE=(1.61*10^{-19}C)(2*10^{7}\frac{V}{m})=3.22*10^{-12}N$

Report an Error

Example Question #3 : Using Coulomb's Law

Two point charges, Q_1 and Q_2 are separated by a distance .

Ps0_twochargeefield

The values of the charges are:

$Q_{1} = - \,2\;nC$

$Q_{2} = + \,5\;nC$

The distance is 4.0cm. The point lies 1.5cm away from Q_1 on a line connecting the centers of the two charges.

What is the magnitude and direction of the net electric field at point due to the two charges?

$k=9*10^9\frac{Nm^2}{C^2}$

Possible Answers:

$8000\ \frac{V}{m},\ \text{toward Q}_1$

$152000\ \frac{V}{m},\ \text{toward Q}_2$

$8000\ \frac{V}{m},\ \text{toward Q}_2$

Correct answer:

Explanation:

At point , the electric field due to Q_1 points toward Q_1 with a magnitude given by:

$E_{1}=\left| \frac{kQ_{1}}{r_{1}^{2}} \right| =\left| \frac{(9*10^9\frac{Nm^2}{C^2})(-2nC)}{(1.5cm)^{2}} \right| =80000 \;\frac{V}{m}$

At point P, the electric field due to Q₂ points away from Q₂ with a magnitude given by

$E_{2}=\left| \frac{kQ_{2}}{r_{2}^{2}} \right|= \left| \frac{(9*10^9\frac{Nm^2}{C^2})(5nC)}{(4.0-1.5cm)^{2}} \right| =72000 \;\frac{V}{m}$

The addition of these two vectors, both pointing in the same direction, results in a net electric field vector of magnitude 152000 volts per meter, pointing toward Q_1 .

$80000\frac{V}{m}+72000\frac{V}{m}=152000\frac{V}{m}$

Report an Error

Example Question #12 : Electricity And Magnetism Exam

A charge, , is enclosed by two spherical surfaces of radii r_1 and , with r_1<r_2 . The cross-sectional side view is shown.

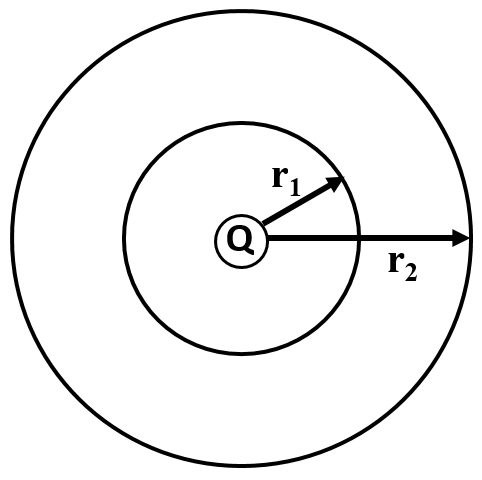
Ps0_gauss

Which is the correct relationship between the electric flux passing through the two spherical surfaces around the point charge?

Possible Answers:

$\phi _{1}>\phi _{2}$

$\phi _{1}=\phi _{2}$

$\phi _{1}=\phi _{2}=0$

$\phi _{1}<\phi _{2}$

Correct answer:

$\phi _{1}=\phi _{2}$

Explanation:

Electric flux is given by either side of the equation of Gauss's Law:

$\phi _{e} = \oint \vec{E}\cdot d\vec{A}=\frac{Q}{\varepsilon_{o} }$

Since the charge is the same for both spherical surfaces, even though these surfaces are of different radii, the amounts of electric flux passing through each surface is the same.

Report an Error

Example Question #1 : Calculating Electric Potential

A proton moves in a straight line for a distance of . Along this path, the electric field is uniform with a value of $2*10^7 \frac{V}{m}$ . Find the potential difference created by the movement.

The charge of a proton is $1.61*10^{-19}\text{C}$ .

Possible Answers:

1*10^9V

1*10^8V

1*10^7V

$1*10^{10}V$

Correct answer:

1*10^8V

Explanation:

Potential difference is given by the change in voltage

$V_a-V_b=\frac{W}{q}$

Work done by an electric field is equal to the product of the electric force and the distance travelled. Electric force is equal to the product of the charge and the electric field strength.

$\frac{W}{q}=\frac{Fd}{q}=\frac{qEd}{q}=Ed$

The charges cancel, and we are able to solve for the potential difference.

$\Delta V=Ed=(2*10^7\frac{V}{m})(5m)=1*10^8V$

Report an Error

Example Question #2 : Calculating Electric Potential

For a ring of charge with radius and total charge , the potential is given by $V=\frac{1}{4\pi\epsilon_0}\frac{q}{\sqrt{x^2+a^2}}$ .

Find the expression for electric field produced by the ring.

Possible Answers:

$\frac{1}{4\pi\epsilon_0}\frac{q}{(x^2+a^2)^{3/2}}}$

$\frac{1}{4\pi\epsilon_0}\frac{qx}{(x^2+a^2)^{3/2}}}$

$\frac{1}{4\pi\epsilon_0}\frac{qx}{(x^2+a^2)^{1/2}}}$

$\frac{1}{4\pi\epsilon_0}\frac{q}{(x^2+a^2)^{1/2}}}$

Correct answer:

$\frac{1}{4\pi\epsilon_0}\frac{qx}{(x^2+a^2)^{3/2}}}$

Explanation:

We know that $E=-\frac{dV}{dx}$ .

Using the given formula, we can find the electric potential expression for the ring.

$E=-\frac{d}{dx}(\frac{1}{4\pi\epsilon_0}\frac{q}{\sqrt{x^2+a^2}})$

Take the derivative and simplify.

$E=-(\frac{q}{4\pi\epsilon_0}\frac{1}{(x^2+a^2)^{3/2}}*-\frac{1}{2}*2x)=\frac{1}{4\pi\epsilon_0}\frac{qx}{(x^2+a^2)^{3/2}}$

Report an Error

Example Question #11 : Electricity And Magnetism Exam

The potential outside of a charged conducting cylinder with radius and charge per unit length is given by the below equation.

$V=\frac{l}{2\pi\epsilon_0}ln\frac{R}{r}$

What is the electric field at a point located at a distance from the surface of the cylinder?

Possible Answers:

$\frac{lr}{2\pi \epsilon_0}$

$\frac{l}{2\pi\epsilon_0r^3 }$

$\frac{l}{2\pi\epsilon_0r^2}$

$\frac{l}{2\pi\epsilon_0r}$

Correct answer:

$\frac{l}{2\pi\epsilon_0r}$

Explanation:

The radial electric field outside the cylinder can be found using the equation $E_r=-\frac{dV}{dr}$ .

Using the formula given in the question, we can expand this equation.

$E_r=-\frac{dV}{dr}=-\frac{d}{dr}(\frac{l}{2\pi\epsilon_0}(lnR-lnr))$

Now, we can take the derivative and simplify.

$E_r=-\frac{1}{2\pi \epsilon_0}(\frac{1}{R}-\frac{1}{r})$

$E_r=-(-\frac{l}{2\pi\epsilon_0r})=\frac{l}{2\pi\epsilon_0r}$

Report an Error

Example Question #12 : Electricity And Magnetism Exam

A proton moves in a straight line for a distance of . Along this path, the electric field is uniform with a value of $2*10^7 \frac{V}{m}$ . Find the work done on the proton by the electric field.

The charge of a proton is $1.61*10^{-19}\text{C}$ .

Possible Answers:

$0.8*10^{-11}J$

$6.44*10^{-11}J$

$3.22*10^{-11}J$

$1.61*10^{-11}J$

Correct answer:

$1.61*10^{-11}J$

Explanation:

Work done by an electric field is given by the product of the charge of the particle, the electric field strength, and the distance travelled.

W=qEd

We are given the charge ( $1.61*10^{-19}\text{C}$ ), the distance (), and the field strength ( $2*10^7 \frac{V}{m}$ ), allowing us to calculate the work.

$W=(1.61*10^{-19}C)(2*10^{7}\frac{V}{m})(5m)$

$W=(3.22*10^{-12}\frac{J}{m})(5m)$

$W=1.61*10^{-11}J$

Report an Error

Example Question #4 : Calculating Electric Potential

A negative charge of magnitude $9.0 \mu C$ is placed in a uniform electric field of $3.0 * 10^4 \frac{N}{C}$ , directed upwards. If the charge is moved 1.0m upwards, how much work is done on the charge by the electric field in this process?

Possible Answers:

$2.7 * 10^{4}J$

$-3.0 * 10^{-13} J$

$-2.7 * 10^{-4}J$

$3.0 * 10^{-13} J$

$-3.3 * 10^{-6}J$

Correct answer:

$-2.7 * 10^{-4}J$

Explanation:

Relevant equations:

$\Delta V = E * d$

$W = q \Delta V$

Given:

$E = 3.0 * 10^4 \frac{N}{C}$

d = 1.0 m

$q = -9.0 \mu C = -9.0 * 10 ^ {-9}C$

First, find the potential difference between the initial and final positions:

$\Delta V = (3.0 * 10^4 \frac{N}{C})(1.0m)= 3.0 * 10^4 \frac{N\cdot m}{C}$

2. Plug this potential difference into the work equation to solve for W:

$W = (-9.0 * 10^{-9}C)(3.0 * 10^4 \frac{N\cdot m}{C})$

$W = -2.7 * 10^{-4}J$

Report an Error

Example Question #11 : Electricity And Magnetism Exam

Three point charges are arranged around the origin, as shown.

Ps0_threechargepotential

$\begin{matrix} &\text{Charge} & \text{Location}\\ Q_1& +3C&(-2cm,0) \\ Q_2&-5C &(4cm,0) \\ Q_3&+1C & (0,5cm) \end{matrix}$

Calculate the total electric potential at the origin due to the three point charges.

$k=9*10^9\frac{Nm^2}{C^2}$

Possible Answers:

$3.41*10^{11}V$

$1.05*10^{12}V$

$2.65*10^{12}V$

$4.05*10^{11}V$

Correct answer:

$4.05*10^{11}V$

Explanation:

Electric potential is a scalar quantity given by the equation:

$V = \frac{kq_{i}}{r_{i}}$

To find the total potential at the origin due to the three charges, add the potentials of each charge.

$V_{total}= V_{1}+V_{2}+V_{3} = \frac{kQ_{1}}{r_{1}}+\frac{kQ_{2}}{r_{2}}+\frac{kQ_{3}}{r_{3}}$

$V_{total}=\frac{(9*10^9)(3C)}{0.02m}+\frac{(9*10^9)(-5C)}{0.04m}+\frac{(9*10^9)(1C)}{0.05m}$

$V_{total}=1.35*10^{12}V+(-1.125*10^{12}V)+1.8*10^{11}V$

$V_{total}=4.05*10^{11}V$

Report an Error

View Tutors

Magdy
Certified Tutor

Cairo University, Egypt, Bachelor of Science, Electrical Engineering. New Mexico State University-Main Campus, Doctor of Phil...

View Tutors

Christopher
Certified Tutor

Mansfield University of Pennsylvania, Bachelor of Science, Physics.

View Tutors

George
Certified Tutor

Northern Arizona University, Bachelors, Chemistry. Purdue University-Main Campus, PHD, Chemistry.

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Spanish Tutors in San Francisco-Bay Area, ACT Tutors in San Diego, Statistics Tutors in Phoenix, Spanish Tutors in Phoenix, ISEE Tutors in Houston, LSAT Tutors in Seattle, Physics Tutors in Houston, Chemistry Tutors in Washington DC, Chemistry Tutors in Dallas Fort Worth, French Tutors in New York City

Popular Courses & Classes

SSAT Courses & Classes in Chicago, SAT Courses & Classes in Houston, SSAT Courses & Classes in Phoenix, LSAT Courses & Classes in Houston, SAT Courses & Classes in Washington DC, GRE Courses & Classes in Seattle, Spanish Courses & Classes in New York City, GMAT Courses & Classes in Phoenix, Spanish Courses & Classes in Chicago, GRE Courses & Classes in Philadelphia

Popular Test Prep

ISEE Test Prep in San Francisco-Bay Area, GMAT Test Prep in San Diego, ACT Test Prep in Dallas Fort Worth, LSAT Test Prep in Houston, GMAT Test Prep in Philadelphia, GMAT Test Prep in Denver, LSAT Test Prep in Atlanta, SAT Test Prep in Atlanta, GMAT Test Prep in Chicago, MCAT Test Prep in Washington DC

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Physics C Electricity : Electricity

Study concepts, example questions & explanations for AP Physics C Electricity

All AP Physics C Electricity Resources

Example Questions

Example Question #11 : Electricity And Magnetism Exam

Example Question #2 : Using Coulomb's Law

Example Question #3 : Using Coulomb's Law

Example Question #12 : Electricity And Magnetism Exam

Example Question #1 : Calculating Electric Potential

Example Question #2 : Calculating Electric Potential

Example Question #11 : Electricity And Magnetism Exam

Example Question #12 : Electricity And Magnetism Exam

Example Question #4 : Calculating Electric Potential

Example Question #11 : Electricity And Magnetism Exam

All AP Physics C Electricity Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: