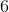Call Now to Set Up Tutoring:

(888) 888-0446

AP Calculus AB : Derivatives

Study concepts, example questions & explanations for AP Calculus AB

Create An Account

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 3 4 5 6 7 8 9 … 72 73 Next →

Example Question #31 : Derivatives

Differentiate the following function.

$f(x)=x^{7}$

Possible Answers:

$f'(x)=7x^{7}$

$f'(x)=7x^{6}$

$f'(x)=6x^{7}$

$f'(x)=6x^{7}+7x^{6}$

$f'(x)=6x^{6}$

Correct answer:

$f'(x)=7x^{6}$

Explanation:

If a function is in the form of $f(x)=x^{a}$ , then the derivative is $f'(x)= a\times x^{a-1}$ .

To solve $f(x)=x^{7}$ you solve bring the 7 down in front of the x and lower the exponent by 1.

This will bring you to the answer $f'(x)=7x^{6}$ .

The apostrophe (') denotes that this is the first derivative of the function.

Report an Error

Example Question #32 : Derivatives

Calculate the derivative of the following:

$x^{2}+12x-36$

Possible Answers:

x^2-12

2x-24

2x+12

Correct answer:

2x+12

Explanation:

Using the power rule which states,

$\frac{d}{dx}x^n=nx^{n-1}$

you can move the from $x^{2}$ to the front and decrease the exponent by which makes it .

For 12x , any term that has an exponent of , the coefficient is its derivative.

Thus, the derivative of 12x is .

Since does not have a variable attached, the derivative will be .

Add your derivatives to get 2x+12 .

Report an Error

Example Question #11 : Derivatives Of Functions

Calculate the derivative of the following:

$x^{3}+8x^{2}-16x+9$

Possible Answers:

$3x^{2}+8x+16$

$3x^{2}+16x-25$

$3x^3+8x^{2}-16$

$3x^{2}+16x-16$

$3x+16x^{2}-16$

Correct answer:

$3x^{2}+16x-16$

Explanation:

Use the power rule to move the exponent of each term to the front, and multiply it with the existing coefficient to create the new coefficient for the derivative.

In mathematical terms, the power rule states,

$\frac{d}{dx}x^n=nx^{n-1}$

Applying the power rule to the first term creates $3x^{2}$ .

Next, move the from $8x^{2}$ to the front and multiply it by , and decrease the exponent by 1 to get 16x .

Next, since 16x does not have an exponent, the derivative of that will be .

Lastly, has a derivative of because there is not variable attached to it.

Therefore the derivative becomes,

$3x^{2}+16x-16$ .

Report an Error

Example Question #12 : Derivatives Of Functions

Calculate the derivative of the following:

$\sqrt{x}$

Possible Answers:

$x^{\frac{1}{2}}$

$\frac{1}{2\sqrt{x}}$

$\frac{1}{2}x^2$

$\frac{1}{2}x$

$x^{2}$

Correct answer:

$\frac{1}{2\sqrt{x}}$

Explanation:

To find the derivative, use the power rule.

In mathematical terms, the power rule states,

$\frac{d}{dx}x^n=nx^{n-1}$

$\sqrt{x}$ is the same as $x^{\frac{1}{2}}$ .

Therefore, move the exponent to the front, and then decrease it by one to get

$\frac{1}{2}x^{-\frac{1}{2}}$ .

After simplifying, you get

$\frac{1}{2\sqrt{x}}$ .

Report an Error

Example Question #35 : Derivatives

Calculate the derivative of the following:

$\left ( x^2-4\right )$

Possible Answers:

2x-4

$2x^{2}$

Correct answer:

Explanation:

Having a binomial does not change the rules for the power rule. You still move the exponent to the front, and decrease the exponent by .

In mathematical terms, the power rule states,

$\frac{d}{dx}x^n=nx^{n-1}$

Constants still have a derivative of

Thus, giving you a final answer of

$\\f(x)=x^2-4 \\f'(x)=2x^{2-1}-0 \\f'(x)=2x$

Report an Error

Example Question #36 : Derivatives

Calculate the derivative of the following:

$\left ( x^2-2\right )^2$

Possible Answers:

$2\left ( x^2-2\right )^2$

$4x\left ( x^2-2\right )^2$

$4x\left ( x^2-2\right )$

$2x\left ( x^2-2\right )$

$2\left ( x^2-2\right )$

Correct answer:

$4x\left ( x^2-2\right )$

Explanation:

Use the chain rule to move the exponent of the binomial to the front, and decrease the exponent by 1. Next, take the derivative of what is on the inside and multiply it with what is one the outside.

In mathematical terms the chain rule is,

$\frac{d}{dx}(f(x))^n\rightarrow n(f(x))\cdot f'(x)$

Identify f(x) and its derivative first.

$\\f(x)=x^2-2 \\f'(x)=2x^{2-1}=2x$

Substituting the function and its derivative into the chain rule formula, the final derivative becomes

$\\f(x)=(x^2-2)^2 \\f'(x)=2(x^2-2)(2x) \\f'(x)=4x(x^2-2)$

Thus, giving you an answer of $4x\left ( x^2-2\right )$ .

Report an Error

Example Question #37 : Derivatives

Calculate the derivative of the following:

$\frac{x^2}{2}$

Possible Answers:

$\frac{1}{2}x^2$

$\frac{x}{2}$

2x^2

Correct answer:

Explanation:

To find the derivative, use the quotient rule.

The quotient rule requires you to do the following:

$\frac{(Denominator)(Deriv. Numerator)-(Numerator)(Deriv. Denominator)}{(Denominator^2)}$

When you apply it to this problem, you get a final answer of,

$\\\text{Numerator}=x^2 \\\text{Deriv. of Numerator}=2x \\\text{Denominator}=2 \\\text{Deriv. of Denominator}=0$

$\\f'(x)=\frac{2(2x)-x^2(0)}{2^2} \\\\f'(x)=\frac{4x}{4} \\\\f'(x)=x$

Report an Error

Example Question #38 : Derivatives

Calculate the derivative of the following:

$12x^{5}-4x^4+10x^3$

Possible Answers:

60x^4+16x^3-30x^2

60x^5-16x^4+30x^3

60x^4-16x^3+30x^2

60x^4+16x^3+30x^2

12x^5-4x^3+10x^2

Correct answer:

60x^4-16x^3+30x^2

Explanation:

Use the power rule to multiply the exponent of each term with its coefficient, to get the derivative of each separate term.

Then, decrease the exponent of each term by

Keep all the signs the same, and your final answer will be

$\\f(x)=12x^{5}-4x^4+10x^3 \\f'(x)=5\cdot 12x^{5-1}-4\cdot 4x^{4-1}+3\cdot 10x^{3-1}$

f'(x)=60x^4-16x^3+30x^2

Report an Error

Example Question #21 : Computation Of The Derivative

Calculate the derivative of the following:

$\sin(x)$

Possible Answers:

$-\sin(x)$

$-\cos(x)$

$-\csc(x)$

$\cos(x)$

$\csc(x)$

Correct answer:

$\cos(x)$

Explanation:

This is a trigonometry identity.

$\frac{d}{dx}\sin(x)=\cos(x)$

The derivative of $\sin(x)$ will always be $\cos(x)$ .

Report an Error

Example Question #21 : Computation Of The Derivative

Calculate the derivative of the following:

$\cos(x)$

Possible Answers:

$-\sec(x)$

$\sin(x)$

$-\sin(x)$

$\sec(x)$

$-\cos(x)$

Correct answer:

$-\sin(x)$

Explanation:

This is a trigonometry identity.

$\frac{d}{dx}\cos(x)=-\sin(x)$

The derivative of $\cos(x)$ will always be $-\sin(x)$ .

Report an Error

← Previous 1 2 3 4 5 6 7 8 9 … 72 73 Next →

View AP Calculus AB Tutors

Edris
Certified Tutor

Boston College, Bachelors, Economics, Mathematics and Biology Minor.

View AP Calculus AB Tutors

Khac Anh Tuan
Certified Tutor

University of British Columbia, Bachelor of Engineering, Engineering, General.

View AP Calculus AB Tutors

Noah
Certified Tutor

Vanderbilt University, Bachelor of Science, Biochemistry.

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Physics Tutors in Seattle, SSAT Tutors in Philadelphia, English Tutors in Atlanta, LSAT Tutors in Chicago, Calculus Tutors in Dallas Fort Worth, Reading Tutors in Chicago, SSAT Tutors in Los Angeles, Spanish Tutors in Chicago, Computer Science Tutors in Denver, Physics Tutors in Washington DC

Popular Courses & Classes

SSAT Courses & Classes in Denver, SSAT Courses & Classes in Boston, LSAT Courses & Classes in Denver, ISEE Courses & Classes in Atlanta, MCAT Courses & Classes in Phoenix, ACT Courses & Classes in Boston, Spanish Courses & Classes in Houston, MCAT Courses & Classes in Chicago, LSAT Courses & Classes in Philadelphia, ACT Courses & Classes in Atlanta

Popular Test Prep

MCAT Test Prep in Houston, ACT Test Prep in Atlanta, ACT Test Prep in Boston, ISEE Test Prep in Dallas Fort Worth, ISEE Test Prep in Denver, LSAT Test Prep in Houston, SSAT Test Prep in Los Angeles, SAT Test Prep in San Diego, MCAT Test Prep in Chicago, ACT Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Calculus AB : Derivatives

Study concepts, example questions & explanations for AP Calculus AB

All AP Calculus AB Resources

Example Questions

Example Question #31 : Derivatives

Example Question #32 : Derivatives

Example Question #11 : Derivatives Of Functions

Example Question #12 : Derivatives Of Functions

Example Question #35 : Derivatives

Example Question #36 : Derivatives

Example Question #37 : Derivatives

Example Question #38 : Derivatives

Example Question #21 : Computation Of The Derivative

Example Question #21 : Computation Of The Derivative

All AP Calculus AB Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: