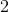All Advanced Geometry Resources
Example Questions
Example Question #51 : Plane Geometry
Find the area of a trapezoid with bases of 


The formula for the area of a trapezoid is:
Where 


Example Question #2 : How To Find The Area Of A Trapezoid

Find the area of the above trapezoid.
The formula for the area of a trapezoid is:
Where 


Example Question #3 : How To Find The Area Of A Trapezoid

Find the area of the above trapezoid.
The formula for the area of a trapezoid is:
Where 


Example Question #1 : How To Find The Area Of A Trapezoid
A trapezoid has a base of length 4, another base of length s, and a height of length s. A square has sides of length s. What is the value of s such that the area of the trapezoid and the area of the square are equal?
In general, the formula for the area of a trapezoid is (1/2)(a + b)(h), where a and b are the lengths of the bases, and h is the length of the height. Thus, we can write the area for the trapezoid given in the problem as follows:
area of trapezoid = (1/2)(4 + s)(s)
Similarly, the area of a square with sides of length a is given by a2. Thus, the area of the square given in the problem is s2.
We now can set the area of the trapezoid equal to the area of the square and solve for s.
(1/2)(4 + s)(s) = s2
Multiply both sides by 2 to eliminate the 1/2.
(4 + s)(s) = 2s2
Distribute the s on the left.
4s + s2 = 2s2
Subtract s2 from both sides.
4s = s2
Because s must be a positive number, we can divide both sides by s.
4 = s
This means the value of s must be 4.
The answer is 4.
Example Question #1 : Trapezoids
What is the area of this regular trapezoid?

26
45
20
32
32
To solve this question, you must divide the trapezoid into a rectangle and two right triangles. Using the Pythagorean Theorem, you would calculate the height of the triangle which is 4. The dimensions of the rectangle are 5 and 4, hence the area will be 20. The base of the triangle is 3 and the height of the triangle is 4. The area of one triangle is 6. Hence the total area will be 20+6+6=32. If you forget to split the shape into a rectangle and TWO triangles, or if you add the dimensions of the trapezoid, you could arrive at 26 as your answer.
Example Question #2 : Trapezoids

What is the area of the trapezoid above if a = 2, b = 6, and h = 4?
16
64
24
8
32
16
Area of a Trapezoid = ½(a+b)*h
= ½ (2+6) * 4
= ½ (8) * 4
= 4 * 4 = 16
Example Question #51 : Plane Geometry
Find the area of a trapezoid if the height is 


Write the formula to find the area of a trapezoid.
Substitute the givens and evaluate the area.
Example Question #11 : How To Find The Area Of A Trapezoid
A right triangle and rectangle are placed adjacent to one another such that the composite figure formed by the triangle and rectangle is a trapezoid.
Find the area of the trapezoid given that the base of the triangle is 7 ft and the hypotenuse of the triangle is 25ft. The base of the rectangle is 9 feet.
Example Question #352 : Advanced Geometry
The average of the lengths of the top and bottom of trapezoid

What is the height of the trapezoid, if the area is 
Example Question #11 : Trapezoids
What is the area of this trapezoid?

The area of a trapezoid is

In this particular case the known values are as follows.
Substituting in the given values into the area formula we arrive at the following solution.
Certified Tutor
Certified Tutor
All Advanced Geometry Resources