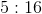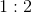All ACT Math Resources
Example Questions
Example Question #1 : Proportion / Ratio / Rate
The ratio of a to b is 9:2, and the ratio of c to b is 5:3. What is the ratio of a to c?
3:1
3:5
14:5
20:3
27:10
27:10
Set up the proportions a/b = 9/2 and c/b = 5/3 and cross multiply.
2a = 9b and 3c = 5b.
Next, substitute the b’s in order to express a and c in terms of each other.
10a = 45b and 27c = 45b --> 10a = 27c
Lastly, reverse cross multiply to get a and c back into a proportion.
a/c = 27/10
Example Question #3 : Proportion / Ratio / Rate
There is a shipment of 50 radios; 5 of them are defective; what is the ratio of non-defective to defective?
50 : 5
9 : 1
1 : 9
1 : 5
5 : 50
9 : 1
Since there are 5 defective radios, there are 45 nondefective radios; therefore, the ratio of non-defective to defective is 45 : 5, or 9 : 1.
Example Question #4 : Proportion / Ratio / Rate
A bag contains 3 green marbles, 5 red marbles, and 9 blue marbles.
What is the ratio of green marbles to blue marbles?
The ratio of green to blue is 
Without simplifying, the ratio of green to blue is 
Since 3 and 9 are both divisible by 3, this ratio can be simplified to 
Example Question #10 : Proportion / Ratio / Rate
A small company's workforce consists of store employees, store managers, and corporate managers in the ratio 10:3:1. How many employees are either corporate managers or store managers if the company has a total of 
Let 








Therefore, the number of employees who are either store managers or corporate managers is 
Example Question #21 : Proportion / Ratio / Rate
The ratio of the number of financial employees who remained in the same role for 2 to 9 years to the number of construction employees who remained in the same role for 0 to 4 years is closest to which of the following?
For this problem, we need to find the number of employees who fall into the categories described, keeping in mind that multiple portions of the pie chart must be accommodated for. Then, we can fit them into a ratio:
For the "2 to 9 years" portion of the financial industry, include
(0.2 + 0.18)(12,000,000) = 4,560,000 workers.
For the "0 to 4 years" portion of the construction industry, include
(0.15 + 0.2)(8,000,000) = 2,800,000 workers.
Now divide and simplify to find the ratio:
4,560,000/2,800,000 = 8/5.
Example Question #1 : How To Find A Ratio A1
The ratio of 







What is the ratio of 

Since the ratios are fixed, regardless of the actual values of 



In order to convert to a form where we can relate 







Thus, we now have 

Setting the 

Example Question #2 : How To Find A Ratio A1
There are thirty cups and fifteen saucers on a shelf. If three saucers are broken and five cups are added, what will be the ratio of cups to saucers?
First, begin by calculating the total number of each item that there will be at the end of the process.
Cups:
Saucers:
The ratio of cups to saucers will thus be:
Example Question #11 : Proportion / Ratio / Rate
Joe needs to repair the roof of his house. He finds two companies that can complete the job. Company A charges an initial cost of $120, plus $15 per hour of labor, while Company B charges an initial cost of $95, plus $20 per hour of labor. After how many hours of labor does Company A cost less than Company B to repair the roof?
6
5
3
4
2
5
In order to solve this problem, create an equation that summarizes the roof repair cost for each company. Begin by composing a formula for Company A, which charges 120 dollars upfront and 15 dollars per hour of labor.
Now, Company B charges 95 dollars upfront and 20 dollars per hour of labor. We can write the following equation:
The question asks us to find how many hours of labor that a repair must take in order for Company A to be cheaper than Company B. In other words, we need to compose an inequality in which the cost of Company A is less than the cost of Company B. We will substitute the variable 
Subtract 
Subtract 95 from both sides of the inequality.
Divide both sides of the inequality by 5.
If the repair will take more than 5 hours, Company A will be cheaper.
Example Question #11 : Proportion / Ratio / Rate
On her birthday in 2013, Molly was three times older than Steve. On her birthday in 2016, Molly was 2 times older than Steve. How old was Steve on Molly's birthday in 2013?
3
2
9
10
12
3
First, let's assign variables to the names of the individuals to represent their age in 2013.
In 2013, Molly was three times older than Steve; therefore, we can write the following expression:
We are also told that in 2016, Molly will be two times older than Steve; thus, we can write another expression:

We can then substitute 

Example Question #1443 : Act Math
The ratio of 





10 to 27
9 to 5
27 to 10
2 to 3
3 to 2
27 to 10
Using the given information we can generate the following two proportions:

Next, cross-multiply each proportion to come up with the following two equations:


Each equation shares a term with the 

Now, we will perform a similar operation on the second equation.
Now, we can set these equations equal to one another.
Drop the equivalent 
The proportion then becomes the following:

Certified Tutor
Certified Tutor
All ACT Math Resources