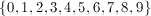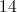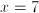All SAT Math Resources
Example Questions
Example Question #1907 : Psat Mathematics
Which set represents all the single-digits integers (0-9) that are either prime, a perfect square, or found in the number 68?
The prime digits are 2, 3, 5, and 7.
The perfect square digits are 0, 1, 4, and 9.
The only digits not represent in these two groups are 6 and 8, which are, coincidentally, found in the number 68.
Example Question #1 : Sets
If four different integers are selected, one from each of the following sets, what is the greater sum that these four integers could have?
W = {4, 6, 9, 10}
X = {4, 5, 8, 10}
Y = {3, 6, 7, 11}
Z = {5, 8, 10, 11}
41
40
39
42
38
38
By observing each of these sets, we can easily determine the largest number in each; however, the problem is asking for us to find the greatest possible sum if we select four different integers, one from each set. The largest number is 11. We will either select this number from set Y or set Z.
We will select set
Y for the 11
because the next larger number in set
Z is 10, which is greater than the next largest number is set Y, 7.
We can use set Z for 10.
Because the 10 has already been selected, choosing the integer from W and X should be easy because 9 is the next largest integer from set W
and
8 is the next largest integer from set X.
11 + 10 + 9 + 8 = 38
Example Question #1 : How To Find The Missing Number In A Set
A team has a win-loss ratio of 

If a team's ratio of wins to losses increases from 

Example Question #821 : Arithmetic
Find the missing number in the following set:
The rule is as follows: add 2 to the previous number then multiply times 2.
The answer is (16 + 2) * 2 = 36.
Example Question #1 : Sets
You have the following data set:

Find the missing number 
In order to find the missing number (X), you must first identify the pattern in the set of numbers. Upon a glance, you can determine that the pattern starts with 4 and then the next number is 2n+1, with n being the preceding number in the set.
For example, let's start with 4. Multiplying 2*4+1 gives us 9, the next number in the set.
Therefore, the missing number in the set is 
Example Question #3 : How To Find The Missing Number In A Set

A sequence of boxes is constructed, the first six of which are seen above. What number is in the lower right corner of the ninth box?
The lower right number of each box is the sum of the other three numbers, which are found as follows:
The upper left numbers are simply the natural numbers in order;
The upper right numbers are the powers of 2, beginning with 
The lower left numbers are the terms of the Fibonacci sequence, which is formed by setting the first two terms equal to 1 and each subsequent term equal to its predecessors.
Therefore, the ninth box in the sequence includes the number 9 as its upper left entry, and 
The lower right entry is the sum of the other entries:
Example Question #1 : How To Find The Number Of Integers Between Two Other Integers
A custom-made ruler is 

There will be 15 gaps of 
Example Question #1 : Counting
Four consecutive odd integers sum to 40. How many of these numbers are prime?
Let x equal the smallest of the four numbers. Therefore:
Therefore the four odd numbers are 7, 9, 11, and 13. Since all are prime except 9, three of the numbers are prime.
Example Question #2 : Counting
The positive integer 
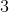
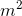





We know that the remainder, 




If 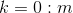

If 





If 




Thus, 
Example Question #1706 : Sat Mathematics
How many integers lie between 

Let's look at a small example, the numbers between 1 and 3 including all endpoints. We have 1, 2, and 3. If we subtract our numbers, we get 2. We need to add one more to get all the desired numbers. We can follow the same process with our larger numbers.
Take,

Then, adding 1,

Therefore, our answer is
All SAT Math Resources