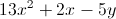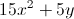We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

HSPT Math : How to simplify expressions

Study concepts, example questions & explanations for HSPT Math

Create An Account

All HSPT Math Resources

6 Diagnostic Tests 130 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Simplify Expressions

You are given that A,B,C are whole numbers.

Which of the following is true of AB +C if and are both odd?

Possible Answers:

AB +C is always odd if is odd, and always even if is even.

None of the other statements are true.

AB +C is always even.

AB +C is always odd if is even, and always even if is odd.

AB +C is always odd.

Correct answer:

AB +C is always odd if is even, and always even if is odd.

Explanation:

If is odd, then is odd, since the product of two odd whole numbers must be odd. When the odd number is added, the result, AB +C , is even, since the sum of two odd numbers must be even.

If is even, then is even, since the product of an odd number and an even number must be even. When the odd number is added, the result, AB +C , is odd, since the sum of an odd number and an even number must be odd.

Report an Error

Example Question #1 : How To Simplify Expressions

Simplify the expression:

3x^2+9x+10x^2+5y+(-11x)

Possible Answers:

11x^2+5y

13x^2-2x+5y

13x^2+2x-5y

15x^2+5y

Correct answer:

13x^2-2x+5y

Explanation:

Combine all the like terms.

The x^2 terms can be combined together, which gives you 13x^2 .

When you combine the terms together, you get -2x .

There is only one term so it doesn't get combined with anything. Put them all together and you get

13x^2-2x+5y .

Report an Error

Example Question #3 : How To Simplify Expressions

Simplify the following expression:

$\dpi{100} \small 2(4x-3x)-6t+5x$

Possible Answers:

$\dpi{100} \small 6x+11x$

$\dpi{100} \small 1x-6t+5$

$\dpi{100} \small 7x-6t$

$\dpi{100} \small 6t-7x$

$\dpi{100} \small 14x - 11xt$

Correct answer:

$\dpi{100} \small 7x-6t$

Explanation:

$\dpi{100} \small 2(4x-3x)-6t+5x$

First distribute the 2: $\dpi{100} \small 8x-6x-6t+5x$

Combine the like terms: $\dpi{100} \small 7x-6t$

Report an Error

Example Question #2 : How To Simplify Expressions

Simplify the expression:

$\frac{(x^2+2x+1)(2x)}{2x^2+2x}$

Possible Answers:

x²+ 2x + 1

2x + 1

x + 1

Correct answer:

x + 1

Explanation:

Factor out a (2x) from the denominator, which cancels with (2x) from the numerator. Then factor the numerator, which becomes (x + 1)(x + 1), of which one of them cancels and you're left with (x + 1).

Report an Error

Example Question #3 : How To Simplify Expressions

Simplify the following expression: x³ - 4(x² + 3) + 15

Possible Answers:

x^3-12x^2+15

x^3-3x^2+15

x^3-12x^2+15

x^3-4x^2+3

x^5+3

Correct answer:

x^3-4x^2+3

Explanation:

To simplify this expression, you must combine like terms. You should first use the distributive property and multiply -4 by x²and -4 by 3.

x³ - 4x² -12 + 15

You can then add -12 and 15, which equals 3.

You now have x³ - 4x² + 3 and are finished. Just a reminder that x³ and 4x²are not like terms as the x’s have different exponents.

Report an Error

Example Question #13 : Simplifying Expressions

Simplify the following expression:

2x(x² + 4ax – 3a²) – 4a²(4x + 3a)

Possible Answers:

–12a^3 – 14a²x + 2x³

–12a³– 14ax² + 2x³

12a³– 16a²x + 8ax² + 2x³

–12a³– 22a²x + 8ax² + 2x³

12a³– 22a²x + 8ax² + 2x³

Correct answer:

–12a³– 22a²x + 8ax² + 2x³

Explanation:

Begin by distributing each part:

2x(x² + 4ax – 3a²) = 2x * x² + 2x * 4ax – 2x * 3a² = 2x³ + 8ax² – 6a²x

The second:

–4a²(4x + 3a) = –16a²x – 12a³

Now, combine these:

2x³ + 8ax² – 6a²x – 16a²x – 12a³

The only common terms are those with a²x; therefore, this reduces to

2x³ + 8ax² – 22a²x – 12a³

This is the same as the given answer:

–12a³– 22a²x + 8ax² + 2x³

Report an Error

Example Question #1002 : Algebra

Which of the following does not simplify to ?

Possible Answers:

All of these simplify to

5x-(6x-2x)

(3-3)x

(x - 1)(x + 2) - x^2 + 2

$\frac{x(4x)}{4x}$

Correct answer:

(3-3)x

Explanation:

5x – (6x – 2x) = 5x – (4x) = x

(x – 1)(x + 2) - x² + 2 = x² + x – 2 – x² + 2 = x

x(4x)/(4x) = x

(3 – 3)x = 0x = 0

Report an Error

Example Question #2 : How To Simplify Expressions

Simplify: x^5(x^2+1)

Possible Answers:

$x^{10} + 1$

x^7 + x^5

x^7 + x

x^7 + 1

$x^{10} + x^5$

Correct answer:

x^7 + x^5

Explanation:

In order to simplify this expression, distribute and multiply the outer term with the two inner terms.

x^5(x^2+1) = x^5(x^2)+x^5(1) = x^7 + x^5

Report an Error

Example Question #5 : How To Simplify Expressions

Simplify: $\frac{c^2 e}{c^{-2}e^{-3}}$

Possible Answers:

$\frac{1}{e^2}$

c^4e^4

$\frac{1}{e^3}$

c^4e^3

$\frac{c^2}{e^2}$

Correct answer:

c^4e^4

Explanation:

When the same bases are multiplied, their exponents can be added. Similarly, when the bases are divided, their exponents can be subtracted. Apply this rule for the given problem.

$\frac{c^2 e}{c^{-2}e^{-3}}=c^{2-(-2)}e^{1-(-3)} = c^4e^4$

Report an Error

Example Question #6 : How To Simplify Expressions

Simplify: $\left (\frac{3}{12} \right )^{-1}$

Possible Answers:

$\frac{1}{9}$

$\frac{1}{4}$

Correct answer:

Explanation:

To simplify this expression, reduce the term inside the parenthesis.

$\left (\frac{3}{12} \right )^{-1}=\left (\frac{1}{4} \right )^{-1}$

Rewrite the negative exponent as a fraction.

$\left (\frac{1}{4} \right )^{-1} = \frac{1}{\left (\frac{1}{4} \right )^{1}} =4$

Report an Error

View Tutors

Omar
Certified Tutor

Arizona State University, Bachelors, Civil Engineering.

View Tutors

Ingrid
Certified Tutor

Northern Illinois University, Bachelor of Science, Elementary Education. Northern Illinois University, Master of Science, Cur...

View Tutors

Ana
Certified Tutor

Grand Canyon University, Bachelors, Education. Grand Canyon University, Masters, Education.

All HSPT Math Resources

6 Diagnostic Tests 130 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

MCAT Tutors in Boston, Statistics Tutors in New York City, Spanish Tutors in Phoenix, Reading Tutors in Atlanta, GRE Tutors in New York City, Algebra Tutors in Chicago, GRE Tutors in Phoenix, Calculus Tutors in New York City, Statistics Tutors in San Diego, SAT Tutors in Boston

Popular Courses & Classes

Spanish Courses & Classes in San Diego, LSAT Courses & Classes in Washington DC, Spanish Courses & Classes in Los Angeles, GMAT Courses & Classes in Houston, LSAT Courses & Classes in Chicago, GRE Courses & Classes in San Diego, LSAT Courses & Classes in Miami, ACT Courses & Classes in San Francisco-Bay Area, SSAT Courses & Classes in Philadelphia, SSAT Courses & Classes in Washington DC

Popular Test Prep

GRE Test Prep in New York City, GMAT Test Prep in Phoenix, GRE Test Prep in Chicago, SSAT Test Prep in San Diego, SSAT Test Prep in Atlanta, SAT Test Prep in San Francisco-Bay Area, MCAT Test Prep in Boston, ACT Test Prep in San Francisco-Bay Area, LSAT Test Prep in Chicago, SAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

HSPT Math : How to simplify expressions

Study concepts, example questions & explanations for HSPT Math

All HSPT Math Resources

Example Questions

Example Question #1 : How To Simplify Expressions

Example Question #1 : How To Simplify Expressions

Example Question #3 : How To Simplify Expressions

Example Question #2 : How To Simplify Expressions

Example Question #3 : How To Simplify Expressions

Example Question #13 : Simplifying Expressions

Example Question #1002 : Algebra

Example Question #2 : How To Simplify Expressions

Example Question #5 : How To Simplify Expressions

Example Question #6 : How To Simplify Expressions

All HSPT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: