GRE Math : Rectangles

Study concepts, example questions & explanations for GRE Math

Create An Account

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 Next →

Example Question #1 : Rectangles

A rectangle has an area of 48 and a perimeter of 28. What are its dimensions?

Possible Answers:

2 x 24

16 x 3

6 x 8

0.25 x 192

1 x 48

Correct answer:

6 x 8

Explanation:

We can set up our data into the following two equations:

(Area) LH = 48

(Perimeter) 2L + 2H = 28

Solve the area equation for one of the two variables (here, length): L = 48 / H

Place that value for L into ever place you find L in the perimeter equation: 2(48 / H) + 2H = 28; then simplify:

96/H + 2H = 28

Multiply through by H: 96 + 2H² = 28H

Get everything on the same side of the equals sign: 2H² - 28H + 96 = 0

Divide out the common 2: H² - 14H + 48 = 0

Factor: (H - 6) (H - 8) = 0

Either of these multiples can be 0, therefore, consider each one separately:

H - 6 = 0; H = 6

H - 8 = 0; H = 8

Because this is a rectangle, these two dimensions are the height and width. If you choose 6 for the "height" the other perpendicular dimension would be 8 and vice-versa. Therefore, the dimensions are 6 x 8.

Report an Error

Example Question #2 : How To Find The Length Of The Side Of A Rectangle

The length of a rectangle is three times its width, and the perimeter is . What is the width of the rectangle?

Possible Answers:

Correct answer:

Explanation:

For any rectangle, 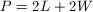$\dpi{100} \small P=2L+2W$ , where $\dpi{100} \small P=perimeter$ , $\dpi{100} \small L=length$ , and $\dpi{100} \small W=width$ .

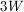
In this problem, we are given that $\dpi{100} \small L=3W$ (length is three times the width), so replace $\dpi{100} \small L$ in the perimeter equation with $\dpi{100} \small 3W$ : $\dpi{100} \small P=2(3W)+2W$

Plug in our value for the perimeter, 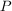$\dpi{100} \small P$ :

$\dpi{100} \small 16=2(3W)+2W$

Simplify:

$\dpi{100} \small 16=6W+2W$

$\dpi{100} \small 16=8W$

$\dpi{100} \small W=2$

Report an Error

Example Question #3 : How To Find The Length Of The Side Of A Rectangle

The area of a rectangle is $70\:in^2$ . Its perimeter is $38\:in$ . What is the length of its shorter side?

Possible Answers:

$8\:in$

$14\:in$

$7\:in$

$5\:in$

$12\:in$

Correct answer:

$5\:in$

Explanation:

We know that the following two equations hold for rectangles. For area:

A=WL

For perimeter:

P=2W+2L

Now, for our data, we know:

70=WL

38=2W+2L

Now, solve the first equation for one of the variables:

$W=\frac{70}{L}$

Now, substitute this value into the second equation:

$38=2(\frac{70}{L})+2L$

Solve for :

$38=\frac{140}{L}+2L$

Multiply both sides by :

38L=140+2L^2

Solve as a quadratic. Divide through by :

19L=70+L^2

Now, get the equation into standard form:

L^2-19L+70=0

Factor this:

(L-14)(L-5)=0

This means that 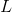(or ) would equal either $5\:in$ or $14\:in$ . Therefore, your answer is $5\:in$ .

Report an Error

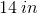
Example Question #1 : How To Find The Length Of The Diagonal Of A Rectangle

Given Rectangle ABCD.

Quantity A: The length of diagonal AC times the length of diagonal BD

Quantity B: The square of half of ABCD's perimeter

Possible Answers:

Quantity B is greater.

The relationship cannot be determined from the information given.

The two quantities are equal.

Quantity A is greater.

Correct answer:

Quantity B is greater.

Explanation:

Suppose ABCD has sides a and b.

The length of one of ABCD's diagonals is given by a²+ b² = c^2,where c is one of the diagonals.

Note that both diagonals are of the same length.

Quantity A: The length of diagonal AC times the length of diagonal BD

This is c * c = c^2.

Quantity A = c² = a²+ b²

Now for Quantity B, remember that the perimeter of a rectangle with sides a and b is Perimeter = 2(a + b).

Half of Perimeter = (a + b)

Square Half of Perimeter = (a + b)²

Use FOIL: (a + b)² = a²+ 2ab + b²

Quantity B = (a + b)² = a²+ 2ab + b²

The question is asking us to compare a²+ b² with a²+ 2ab + b^2.

Note that as long as a and b are positive numbers (in this case a and b are dimensions of a rectangle, so they must be positive), the second quantity will be greater.

Report an Error

Example Question #2 : How To Find The Length Of The Diagonal Of A Rectangle

If rectangle ABCD has a perimeter of , and the longer edge is 2.4 times longer than the shorter edge, then how long is the diagonal ?

Possible Answers:

Correct answer:

Explanation:

Lets call our longer side L and our shorter side W.

If the perimeter is equal to 68, then

2L+2W=68 .

We also have that

L=2.4W .

If we then plug this into our equation for perimeter, we get 6.8W=68 .

Therefore, W=10 and L=24 . Using the Pythagorean Theorem, we have 10^2+24^2=676=D^2 so D=26 .

Report an Error

Example Question #1 : How To Find The Area Of A Rectangle

If a rectangle's width is increased by 20%, and its length is decreased by 20%, which statement most accurately reflects the rectangle's change in area?

Possible Answers:

The rectangle's area will be decreased by 10.%

The rectangle's area will be increased by 4%.

The rectangle's area will be reduced by 4%.

The rectangle's area will be increased by 10%.

The rectangle's area will not change.

Correct answer:

The rectangle's area will be reduced by 4%.

Explanation:

If l x w = A, then we will call the new area B. B results when l has been decreased by 20% and w has been increased by 20%: in other words, 80% of l and 120% of w.

This is expressed as (0.8) l * (1.2) w = B

The simplest way to determine the result is to create rectangles with actual integers and see what happens. Let's say our first rectangle is a square 10 units by 10 units.

10 * 10 = 100 for our A.

Plugging these numbers into our new equation gives us:

(0.8)10 * (1.2)10 = 96.

So the final step is to compare the areas. Because we used a 10 x 10 rectangle as our example, this step will be easy, since 100% = 100.

96/100 = 0.96

0.96 * 100 = 96%

Which reflects a 4% reduction in the size of the rectangle.

Any other length and width used for this rectangle will result in a 4% reduction in size when the above parameters are applied.

Report an Error

Example Question #2 : How To Find The Area Of A Rectangle

Amy is building a rectangular patio in her backyard. She plans on making the patio 8 feet wide by 12 feet long. The rectangular bricks she is using are 6 inches long and 4 inches wide. How many bricks will Amy need to build her patio?

Possible Answers:

384

576

782

424

Correct answer:

576

Explanation:

Because Amy is building her patio in a rectangle, and the bricks are rectangular, the simplest way to find this solution is to determine how many bricks long and how many bricks wide her patio will be.

To do this, begin by converting the patio's dimensions into inches, since the bricks Amy is using are measured in inches.

Length:

12 feet * 12 inches in a foot = 144 inches long.

Width:

8 feet * 12 inches in a foot = 96 inches wide.

Now, all that's left to do is figure out how many bricks will fit in these spaces. Let's be intuitive and assume the long sides of the bricks will align with the long sides of the patio.

144 inches long / bricks that are 6 inches long = 24 bricks long

Amy will need columns of 24 bricks to cover the length of her patio. Now, for the width.

96 inches wide / bricks that are 4 inches wide = 24 bricks wide

Amy will need rows of 24 bricks to cover the width of her patio.

To determine how many bricks Amy will need in total, the last step is to multiply how many rows of bricks she will need by how many columns.

24 bricks to a row * 24 bricks to a column = 576

Amy needs 576 bricks to build her patio.

Report an Error

Example Question #3 : How To Find The Area Of A Rectangle

Quantity A: The area of a rectangle with a perimeter of 40

Quantity B: The area of a triangle with a perimeter of 40

Possible Answers:

The relationship cannot be determined from the information given

The two quantities are equal

Quantity A is greater

Quantity B is greater

Correct answer:

The relationship cannot be determined from the information given

Explanation:

The area of the two shapes depends on their dimensions, which can vary greatly among different combinations. A rectangle with a perimeter of 40 could have dimensions of 19*1 (smaller area) or 10*10 (larger area), for instance. Similarly, a triangle could have sides of 10--11--19 (smaller area) or 13--13--14 (larger area). Thus, the relationship cannot be determined without more specific details.

Report an Error

Example Question #1 : How To Find The Area Of A Rectangle

The perimeter of a rectangle is $130\:in$ . One side is $15\:in$ . What is the area of this rectangle?

Possible Answers:

$575\:in^2$

$\frac{932}{3}\:in^2$

$750\:in^2$

$\frac{410}{3}\:in^2$

$441\:in^2$

Correct answer:

$750\:in^2$

Explanation:

Recall that perimeter is defined as:

P=2W+2L

For our data, this is:

130=2*15+2L

Solve for :

130=30+2L

100=2L

Therefore, $L=50 \:in$

This means that the area of the rectangle is equal to:

$A=WL=50*15=750\:in^2$

Report an Error

Example Question #1 : How To Find The Area Of A Rectangle

Rectangle $\dpi{100} \small A$ has a length of 20 inches and a width of 3 inches. Rectangle $\dpi{100} \small B$ has a length of 9 inches and a width of 10 inches. By what number must the area of rectangle $\dpi{100} \small A$ be multiplied by to equal the area of rectangle $\dpi{100} \small B$ ?

Possible Answers:

$\dpi{100} \small 1.5$

$\dpi{100} \small 3.5$

$\dpi{100} \small 2$

$\dpi{100} \small 1$

$\dpi{100} \small 5$

Correct answer:

$\dpi{100} \small 1.5$

Explanation:

Area of rectangle $\dpi{100} \small A$ is $\dpi{100} \small 20\times 3=60$ .

Area of rectangle $\dpi{100} \small B$ is $\dpi{100} \small 9\times 10=90$

$\dpi{100} \small 60x=90$

$\dpi{100} \small x=1.5$

Report an Error

← Previous 1 2 Next →

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in Dallas Fort Worth, Math Tutors in Dallas Fort Worth, Physics Tutors in San Francisco-Bay Area, GRE Tutors in Atlanta, ACT Tutors in Houston, English Tutors in Atlanta, GRE Tutors in Washington DC, SSAT Tutors in Houston, GMAT Tutors in Phoenix, LSAT Tutors in Atlanta

Popular Courses & Classes

ISEE Courses & Classes in Atlanta, GMAT Courses & Classes in Philadelphia, GRE Courses & Classes in Phoenix, ACT Courses & Classes in Chicago, SSAT Courses & Classes in San Diego, SAT Courses & Classes in Boston, Spanish Courses & Classes in Atlanta, Spanish Courses & Classes in Chicago, MCAT Courses & Classes in Phoenix, ISEE Courses & Classes in Washington DC

Popular Test Prep

GRE Test Prep in Denver, MCAT Test Prep in Houston, SSAT Test Prep in Houston, ISEE Test Prep in Denver, SAT Test Prep in Washington DC, GMAT Test Prep in Phoenix, ACT Test Prep in Chicago, SAT Test Prep in Houston, ACT Test Prep in Dallas Fort Worth, ACT Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

Email address:
Your name:
Feedback:

GRE Math : Rectangles

Study concepts, example questions & explanations for GRE Math

All GRE Math Resources

Example Questions

Example Question #1 : Rectangles

Example Question #2 : How To Find The Length Of The Side Of A Rectangle

Example Question #3 : How To Find The Length Of The Side Of A Rectangle

Example Question #1 : How To Find The Length Of The Diagonal Of A Rectangle

Example Question #2 : How To Find The Length Of The Diagonal Of A Rectangle

Example Question #1 : How To Find The Area Of A Rectangle

Example Question #2 : How To Find The Area Of A Rectangle

Example Question #3 : How To Find The Area Of A Rectangle

Example Question #1 : How To Find The Area Of A Rectangle

Example Question #1 : How To Find The Area Of A Rectangle

All GRE Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details