GRE Math : How to find the length of the side of a square

Study concepts, example questions & explanations for GRE Math

Create An Account

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Find The Length Of The Side Of A Square

Quantity A:

The side-length of a square with a perimeter of .

Quantity B:

The side-length of a square with an area of .

Possible Answers:

Both quantities are equal

Quantity B is greater

Quantity A is greater

The relationship cannot be determined from the information given

Correct answer:

Quantity A is greater

Explanation:

The first step to a quantitative comparison is to determine whether it can be solved at all with the given knowledge. Since all you need to find the side-length of a square is the perimeter, the area, OR the diagonal and we have one of each for these two quantities, this relationship can be determined. Thus, "the relationship cannot be determined" is out.

Now, to solve both quantities. Quantity A can be solved by translating the perimeter into side lengths: the formula for the perimeter of a square is P = 4s , with being the side-length, so you just need to divide the perimeter by four.

30=4s

$\frac{30}{4}=s$

Thus, quantity A is 7.5 .

Quantity B can be solved by translating the area into side lengths: the formula for the area of a square is $A=l \times w$ , or $A=s^{2}$ , with being the side-length, so you just need to find the square root of the area.

30=s^2

$\sqrt30=s$

Thus, quantity B is roughly 5.48 .

Therefore quantity A is greater.

Report an Error

Example Question #1 : How To Find The Length Of The Side Of A Square

Inscribedsquare

Circle has a center in the center of Square ABCD .

If the area of Circle is $324\pi$ , what is the length of ?

Possible Answers:

324

162

Correct answer:

Explanation:

If the area of Circle is $324\pi$ , we know that the area can be computed using the standard area formula:

$A = \pi r^2$ , using $324\pi$ for

$324\pi = \pi r^2$

Simplifying, we get:

324=r^2

We know that must be less than . By choosing for , we find out that this is the radius of our circle. Thus, we know that the diameter of the circle is double this, or . Now, consider the following diagram:

Inscr18

Notice that the diameter is the same length as a side of the square. Thus, is equal to .

Report an Error

Example Question #1 : How To Find The Length Of The Side Of A Square

Prb11 1

Circle has a center in the center of square ABCD .

The line segment marked with length 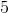lies on the diagonal of the square ABCD .

What is the length of side ?

Possible Answers:

This cannot be computed from the given data

$\frac{1}{\sqrt{2} + 1}$

$\frac{10}{\sqrt{2} - 1}$

$\frac{3\sqrt{2}-4}{5}$

$\sqrt{2}+7$

Correct answer:

$\frac{10}{\sqrt{2} - 1}$

Explanation:

You can further fill in your diagram as follows:

Prb11 2

Now, we know that the triangle BCD is a 45-45-90 triangle. We also know that the length of and must also be equal to the diameter of the circle. (The diameter of the circle will run across the circle horizontally if you draw it that way. This will provide you with a complete side length.) Now, we know that the ratio of the hypotenuse of BCD to the side must be the same as:

$\frac{\sqrt{2}}{1}$

For our data, that means:

$\frac{5+5+d}{d} = \frac{\sqrt{2}}{1}$

Simplifying, we know:

$\frac{10+d}{d} = \frac{\sqrt{2}}{1}$

$\frac{10}{d}+1 = \sqrt{2}$

$\frac{10}{d} = \sqrt{2} - 1$

Now, make both sides reciprocals:

$\frac{d}{10} = \frac{1}{\sqrt{2} - 1}$

Finally, solve:

$d = \frac{10}{\sqrt{2} - 1}$

Recall, this is both the length of the side and the diameter of the circle. Hence, you have your answer.

Report an Error

All GRE Math Resources

13 Diagnostic Tests 452 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Computer Science Tutors in Boston, ISEE Tutors in Dallas Fort Worth, ISEE Tutors in Denver, GRE Tutors in San Francisco-Bay Area, Math Tutors in Los Angeles, ACT Tutors in Denver, ISEE Tutors in Seattle, GMAT Tutors in Los Angeles, GRE Tutors in Denver, Statistics Tutors in Dallas Fort Worth

Popular Courses & Classes

MCAT Courses & Classes in Chicago, GMAT Courses & Classes in Miami, MCAT Courses & Classes in Phoenix, SSAT Courses & Classes in Washington DC, GMAT Courses & Classes in New York City, SSAT Courses & Classes in Miami, SAT Courses & Classes in Chicago, SSAT Courses & Classes in Phoenix, GMAT Courses & Classes in Seattle, ISEE Courses & Classes in Denver

Popular Test Prep

MCAT Test Prep in Los Angeles, MCAT Test Prep in Denver, ACT Test Prep in San Francisco-Bay Area, GRE Test Prep in Los Angeles, GRE Test Prep in San Diego, SAT Test Prep in Washington DC, ACT Test Prep in San Diego, ISEE Test Prep in Atlanta, LSAT Test Prep in New York City, SSAT Test Prep in Seattle

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

Email address:
Your name:
Feedback:

GRE Math : How to find the length of the side of a square

Study concepts, example questions & explanations for GRE Math

All GRE Math Resources

Example Questions

Example Question #1 : How To Find The Length Of The Side Of A Square

Example Question #1 : How To Find The Length Of The Side Of A Square

Example Question #1 : How To Find The Length Of The Side Of A Square

All GRE Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details