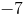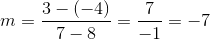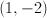GRE Math : Geometry
Study concepts, example questions & explanations for GRE Math
All GRE Math Resources
Example Questions
Example Question #1 : How To Find The Slope Of A Line
What is the slope of the line:
First put the question in slope intercept form (y = mx + b):
–(1/6)y = –(14/3)x – 7 =>
y = 6(14/3)x – 7
y = 28x – 7.
The slope is 28.
Example Question #2 : How To Find The Slope Of A Line
What is the slope of a line that passes though the coordinates 
The slope is equal to the difference between the y-coordinates divided by the difference between the x-coordinates.
Use the give points in this formula to calculate the slope.
Example Question #41 : Coordinate Geometry
What is the slope of a line running through points 
The slope is equal to the difference between the y-coordinates divided by the difference between the x-coordinates.
Use the give points in this formula to calculate the slope.
Example Question #11 : Other Lines
Find the point where the line y = .25(x – 20) + 12 crosses the x-axis.
(0,–28)
(–28,0)
(0,0)
(–7,0)
(12,0)
(–28,0)
When the line crosses the x-axis, the y-coordinate is 0. Substitute 0 into the equation for y and solve for x.
.25(x – 20) + 12 = 0
.25x – 5 = –12
.25x = –7
x = –28
The answer is the point (–28,0).
Example Question #41 : Lines
On a coordinate plane, two lines are represented by the equations 
You can solve for the 
Solve for 
Example Question #42 : Lines
If the two lines represented by 
Solve for 
Plugging 
These are the coordinates for the intersection of the two lines.
Example Question #43 : Lines
Determine the greater quantity:
or
The relationship cannot be determined.
The quantities are equal.
The quantities are equal.
Example Question #3 : How To Find Out If A Point Is On A Line With An Equation
Which of the following set of points is on the line formed by the equation 
The easy way to solve this question is to take each set of points and put it into the equation. When we do this, we find the only time the equation balances is when we use the points 
For practice, try graphing the line to see which of the points fall on it.
Example Question #2 : How To Find Out If A Point Is On A Line With An Equation
Consider the lines described by the following two equations:
4y = 3x2
3y = 4x2
Find the vertical distance between the two lines at the points where x = 6.
44
48
12
21
36
21
Since the vertical coordinates of each point are given by y, solve each equation for y and plug in 6 for x, as follows:
Taking the difference of the resulting y -values give the vertical distance between the points (6,27) and (6,48), which is 21.
Example Question #3 : Other Lines
For the line
Which one of these coordinates can be found on the line?
(3, –6)
(9, 5)
(6, –12)
(6, 5)
(3, 7)
(3, –6)
To test the coordinates, plug the x-coordinate into the line equation and solve for y.
y = 1/3x -7
Test (3,-6)
y = 1/3(3) – 7 = 1 – 7 = -6 YES!
Test (3,7)
y = 1/3(3) – 7 = 1 – 7 = -6 NO
Test (6,-12)
y = 1/3(6) – 7 = 2 – 7 = -5 NO
Test (6,5)
y = 1/3(6) – 7 = 2 – 7 = -5 NO
Test (9,5)
y = 1/3(9) – 7 = 3 – 7 = -4 NO
All GRE Math Resources
