GMAT Math : GMAT Quantitative Reasoning
Study concepts, example questions & explanations for GMAT Math
All GMAT Math Resources
Example Questions
Example Question #1 : Calculating The Length Of The Side Of A Quadrilateral
A trapezoid has bases of length one mile and 4,000 feet and height one-half mile. What is the length of its midsegment?
The length of the midsegment of a trapezoid is the mean of the lengths of its bases; the height is irrelevant. The bases are of length 4,000 feet and 5,280 feet; their mean is
Example Question #3 : Calculating The Length Of The Side Of A Quadrilateral
A given trapezoid has an area of 
The area 
Plugging in our values for 
Example Question #124 : Quadrilaterals
A given trapezoid has an area of 
The area 
Plugging in our values for 
Example Question #125 : Quadrilaterals
A given trapezoid has an area of 
The area 
Plugging in our values for 
Example Question #1 : Calculating An Angle In A Quadrilateral
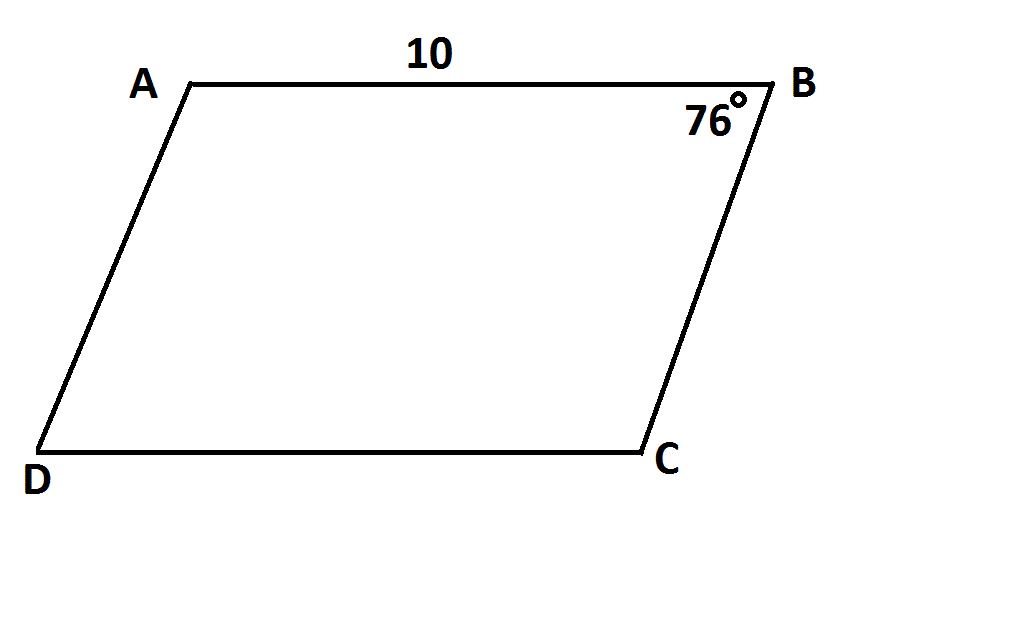
Note: Diagram is NOT drawn to scale.
Refer to the above diagram.
Any of the following facts alone would be enough to prove that 
Any one of these facts alone would prove that 
Opposite sides of a parallelogram are congruent; if 
Consecutive angles of a parallelogram are supplementary; if 
Opposite angles of a parallelogram are congruent; if 
Adjacent sides of a parallelogram, however, may or may not be congruent, so the condition that 
Example Question #1 : Calculating An Angle In A Quadrilateral
Which of the following can not be the measures of the four interior angles of a quadrilateral?
All four of the other choices fit the conditions.
The four interior angles of a quadrilateral measure a total of 
This last group does not have the correct sum, so it is the correct choice.
Example Question #3 : Calculating An Angle In A Quadrilateral
A circle can be circumscribed about each of the following figures except:
A right scalene triangle
A rhombus with a 
A rectangle twice as long as it is wide
An isosceles trapezoid with one base three times as long as the other
An isosceles triangle with its base one-half as long as either leg
A rhombus with a
A circle can be circumscribed about any triangle regardless of its sidelengths or angle measures, so we can eliminate the two triangle choices.
A circle can be circumscribed about a quadrilateral if and only if both pairs of its opposite angles are supplementary. An isosceles trapezoid has this characteristic; this can be proved by the fact that base angles are congruent, and by the Same-Side Interior Angles Statement. For a parallelogram to have this characteristic, since opposite angles are congruent also, all angles must measure 
Example Question #4 : Calculating An Angle In A Quadrilateral
Rhombus 
What is 
The diagonals of a rhombus always intersect at right angles, so 
Example Question #241 : Geometry
Quadrilateral 
Two opposite angles of a quadrilateral inscribed inside a circle are supplementary, so
Example Question #241 : Geometry
Note: Figure NOT drawn to scale.
The above figure is of a rhombus and one of its diagonals. What is 
Not enough information is given to answer the question.
The four sides of a rhombus are congruent, so a diagonal of the rhombus cuts it into two isosceles triangles. The two angles adjacent to the diagonal are congruent, so the third angle, the one marked, measures:
All GMAT Math Resources
