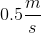All AP Physics 2 Resources
Example Questions
Example Question #82 : Fluids
Water flows through a tube with a diameter of 2m at a rate of 
The velocity of the water can be determined from the following formula:
We need to calculate the volumetric flow rate and the cross-sectional area. For the flow rate:
Rearrange to solve for volumetric flow rate:
Next, calculate cross-sectional area:
Now we can solve for the velocity:
Example Question #2 : Flow Rate
Suppose that water flows from a pipe with a diameter of 1m into another pipe of diameter 0.5m. If the speed of water in the first pipe is 
To find the answer to this question, we'll need to use the continuity equation to determine the flow rate, which will be the same in both pipes.
We'll also need to calculate the area of the pipe using the equation:
Solve the combined equation for 
Example Question #3 : Flow Rate
A diameter garden hose with a diameter of 3cm sprays water travels through a hose at 
Use the continuity equation for incompressible fluids.
The cross sectional area of the garden hose at both ends are circular regions. Rewrite the equation replacing areas with the formula for an area of a circle and solve for the velocity at the second point.
Example Question #4 : Flow Rate
An civil engineer is designing the outflow of a pond. The pond has a radius of 


This is a volume flow rate problem. Because the water is an incompressible fluid, we can apply the flow rate equation:
Find the surface area of the pond:
Substitute into the flow rate equation:
Example Question #1 : Flow Rate
An incompressible fluid flows through a pipe. At location 1 along the pipe, the volume flow rate is 
When the area halves, the velocity of the fluid will double. However, the volume flow rate (the product of these two quantities) will remain the same. In other words, the volume of water flowing through location 1 per second is the same as the volume of water flowing through location 2 per second.
Example Question #91 : Fluids
What is the volumetric flow rate of oil in a 

The volumetric flow rate of fluid is found using the equation:
Where 

In this problem the cross-section of the pipe is a circle. The area of the cross-section is:
The volumetric flow rate is:
Example Question #92 : Fluids
What is the volumetric flow rate of ethanol flowing through a square pipe with sidelength 4m? The velocity of the ethanol is 
The volumetric flow rate of fluid is found using the equation:
Where 

In this problem the cross-section of the pipe is a square. The area of the cross-section is:
The volumetric flow rate is:
Example Question #21 : Fluid Dynamics
A pipe narrows from a 



The volumetric flow rate of fluid is found using the equation:
Where 


In this problem, the cross-section of the pipe is a circle, which is
The area of the exit cross-section is:
Plug in these variables into the continuity equation and solve:
Example Question #2 : Flow Rate
Fluid enters a pipe at 


The volumetric flow rate of fluid is found using the equation:
Where 

Use the continuity equation, we see that 
In this problem,
The cross-section of the pipe is a circle, which is:
Plugging our variables into the continuity equation gives us
Example Question #1 : Flow Rate
Fluid flows through a pipe whose diameter goes from 

The fluid in both sections of the pipe have the same velocity
The fluid in both sections of the pipe have the same cross-sectional area
The fluid in both sections of the pipe have the same volumetric flow rate
The fluid in both sections of the pipe are not related
The fluid in both sections of the pipe have the same volumetric flow rate
Based on the continuity equation, both parts of the flow must have the same volumetric flow rate.
All AP Physics 2 Resources