All AP Calculus AB Resources
Example Questions
Example Question #2 : Calculus 3
Evaluate the following limit:
Does not exist.
First, let's multiply the numerator and denominator of the fraction in the limit by 
As 




The answer is 
Example Question #12 : Calculus 3
Let 


What is the value of 
Since 




We are asked to find 




The given information tells us that
The equation then becomes 
We can now solve for 

The answer is 
Example Question #11 : Fundamental Theorem Of Calculus
Using the fundamental theorem of calculus, find the integral of the function 


The fundamental theorem of calculus is, 
We can use the inverse power rule to solve the integral, which is 
Example Question #1 : Use Of The Fundamental Theorem To Evaluate Definite Integrals
Use the fundamental theorem of Calculus to evaluate the definite integral
2
Here we use the fundamental theorem of Calculus:

Here we do not worry about adding a constant c because we are evaluating a definite integral.
Example Question #2 : Use Of The Fundamental Theorem To Evaluate Definite Integrals
Evaluate 
We can integrate this without too much trouble


![=[-2t^{-1}]_1^x](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/877151/gif.latex)


Note that we were not asked to evaluate 

Example Question #122 : Integrals
Using the Fundamental Theorem of Calculus and simplify completely solve the integral.
To solve the integral, we first have to know that the fundamental theorem of calculus is

Since 
To find the anti-derivative, we have to know that in the integral, 

The anti-derivative of the function 


According to rules of logarithms, when subtracting two logs is the same as taking the log of a fraction of those two values:

Then, we can simplify to a final answer of
Example Question #123 : Integrals
Using the Fundamental Theorem of Calculus solve the integral.
To solve the integral using the Fundamental Theorem, we must first take the anti-derivative of the function. The anti-derivative of 


When we do this,


The next step is to find the difference between the values at each limit of integration, because the Fundamental Theorem states

Thus, we subtract 

Example Question #124 : Integrals
Solve 
To solve the integral, we first have to know that the fundamental theorem of calculus is

Since 
The anti-derivative of the function 


When we plug 3 into the anti-derivative, the solution is 
To find the final answer, we must take the difference of these two solutions, so the final answer is 
Example Question #125 : Integrals
Solve 
To solve the integral, we first have to know that the fundamental theorem of calculus is

Since 
The anti-derivative of the function

is

so we must evaluate 
When we plug 3 into the anti-derivative, the solution is 
To find the final answer, we must take the difference of these two solutions, so the final answer is 
Example Question #7 : Use Of The Fundamental Theorem To Evaluate Definite Integrals
Evaluate the indefinite integral:
First compute the indefinite integral:
Note that the 

Now the the integral can be written in terms of
Therefore:
When we go to compute the indefinite integral the constant of integration 
We can precede by either going back to the original variable 

Solution 1)



Solution 2)
We could have also solved without converting back to the original variable. Instead, we could just change the limits of integration. Use the definition assigned to the variable 




All AP Calculus AB Resources

















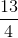





![=\left[ \frac{1^4}{4}+1^2+9(1)\right]-\left[\frac{0^4}{4}+0^2+9(0) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/1002842/gif.latex)





![2-\frac{\pi]}{2}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/860884/gif.latex)






























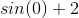













![\int_0^{\frac{\pi}{4}}\tan(x)\sec^2(x)dx=\left[ \frac{1}{2}\tan^2(x)\right ]_{x=0}^{x=\frac{\pi}{4}}\: \: =\: \: \: \: \frac{1}{2}\tan^2\left(\frac{\pi}{4}\right) -\frac{1}{2}\tan^2\left(0\right)](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/933356/gif.latex)



![\int_0^1 udu =\left[\frac{1}{2}u^2 \right ]_{u=0}^{u=1} =\frac{1}{2}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/926667/gif.latex)



