All GMAT Math Resources
Example Questions
Example Question #1 : How To Graph A Function
The chord of a 

The radius 

The circle, the central angle, and the chord are shown below:

By way of the Isosceles Triangle Theorem, 

Example Question #1 : How To Graph A Function
The chord of a 

The radius 

The circle, the central angle, and the chord are shown below, along with 

We concentrate on 
and
The chord 
Example Question #1 : Chords
The chord of a 

A circle with circumference 

The circle, the central angle, and the chord are shown below, along with 


We concentrate on 


and
The chord 
Example Question #341 : Problem Solving Questions
The chord of a 

The radius 

The circle, the central angle, and the chord are shown below:

By way of the Isosceles Triangle Theorem, 


This is the correct response.
Example Question #102 : Geometry
The chord of a 

A circle with circumference 

The circle, the central angle, and the chord are shown below:

By way of the Isosceles Triangle Theorem, 


Example Question #103 : Geometry
Consider the Circle 

(Figure not drawn to scale.)
If 


This is a triangle question in disguise. We have a ninety-degree triangle with two sides made up of the radii of the circle. This means the other two angles (


Use the 45/45/90 triangle ratios to find the final side. Additionally, you could use Pythagorean Theorem to find the missing side.
45/45/90 side length ratios:
Segment
Or, using the Pythagorean Theorem, 


Example Question #342 : Problem Solving Questions
Calculate the length of a chord in a circle with a radius of 

We are given the radius of the circle and the perpendicular distance from its center to the chord, which is all we need to calculate the length of the chord. Using the formula for chord length that involves these two quantities, we find the solution as follows, where 


Example Question #343 : Problem Solving Questions
The chord of a 

A circle with circumference 

The circle, the central angle, and the chord are shown below:

By way of the Isosceles Triangle Theorem, 

Example Question #9 : Calculating The Length Of A Chord
The arc 



The figure referenced is below.
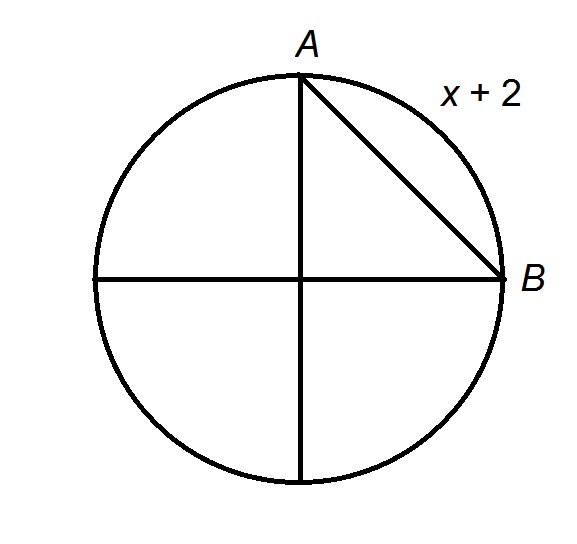
The arc is 

The radius is this circumference divided by 



All GMAT Math Resources











































































