All GMAT Math Resources
Example Questions
Example Question #21 : Quadrilaterals
The ratio of square A to square B is 3:1. Find the perimeter of square B.
- The area of square A is
.
- The length of the diagonal of square B is
.
Statement 2 alone is sufficient, but statement 1 alone is not sufficient to answer the question.
Statement 1 alone is sufficient, but statement 2 alone is not sufficient to answer the question.
Statements 1 and 2 are not sufficient, and additional data is needed to answer the question.
Both statements taken together are sufficient to answer the question, but neither statement alone is sufficient.
Each statement alone is sufficient to answer the question.
Each statement alone is sufficient to answer the question.
Statement 1: We can use the area to find the length of square A's side.
Keep in mind that the ratio of square A to square B is 3:1.
Now that we know the length of the side, we can find the perimeter of square B.
Statement 2: We can use the diagonal to find the length of the side.
We can easily see the side measures 
Example Question #2 : Dsq: Calculating The Perimeter Of A Square
What is the perimeter of the square?
- A side measures
.
- The area of the square is
.
Each statement alone is sufficient to answer the question.
Statement 1 alone is sufficient, but statement 2 alone is not sufficient to answer the question.
Statement 2 alone is sufficient, but statement 1 alone is not sufficient to answer the question.
Both statements taken together are sufficient to answer the question, but neither statement alone is sufficient.
Statements 1 and 2 are not sufficient, and additional data is needed to answer the question.
Each statement alone is sufficient to answer the question.
Recall the formula for perimeter of a square:

where 
Statement 1: We're given 
Statement 2: We're given the area 

With 
Each statement alone is sufficient to answer the question.
Example Question #66 : Geometry
Find the perimeter of the square.
- The diagonal measures
inches.
- The diagonal is found by
where
represents a square's side length.
Statement 1 alone is sufficient, but statement 2 alone is not sufficient to answer the question.
Each statement alone is sufficient to answer the question.
Statements 1 and 2 are not sufficient, and additional data is needed to answer the question.
Statement 2 alone is sufficient, but statement 1 alone is not sufficient to answer the question.
Both statements taken together are sufficient to answer the question, but neither statement alone is sufficient.
Statement 1 alone is sufficient, but statement 2 alone is not sufficient to answer the question.
Statement 1: We can find the length of the square's side using the information given.

so
We can now find the perimeter of the square: 
Statement 2: In order to find the length of the square's side using the information provided in Statement 1, we need to use this equation.
Statement 1 alone is sufficient, but statement 2 alone is not sufficient to answer the question.
Example Question #181 : Data Sufficiency Questions
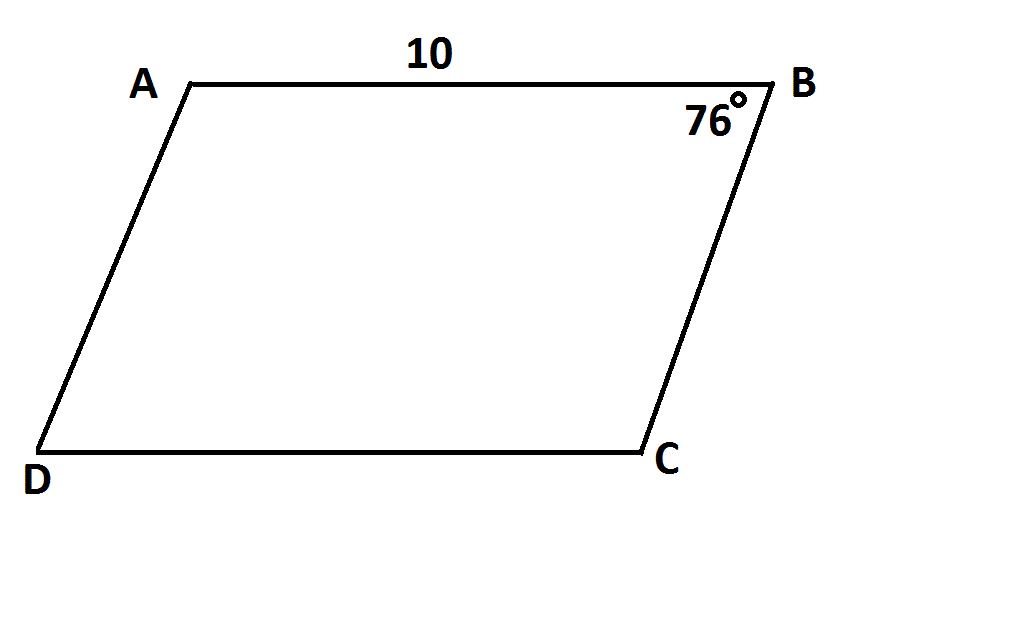
NOTE: Figure NOT drawn to scale.
Is the above figure a parallelogram?
Statement 1:
Statement 2:
EITHER statement ALONE is sufficient to answer the question.
BOTH statements TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient to answer the question.
BOTH statements TOGETHER are insufficient to answer the question.
Statement 2 ALONE is sufficient to answer the question, but Statement 1 ALONE is NOT sufficient to answer the question.
Statement 1 ALONE is sufficient to answer the question, but Statement 2 ALONE is NOT sufficient to answer the question.
BOTH statements TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient to answer the question.
Knowing one pair of sides of a quadrilateral to be congruent is not alone sufficient to prove that figure to be a parallelogram, nor is knowing one pair of sides of a quadrilateral to be parallel. But knowing both about the same pair of sides is sufficient by a theorem of parallelograms.
Example Question #181 : Data Sufficiency Questions

Notes: 
Calculate 
Statement 1:
Statement 2: The area of the trapezoid is 7,000.
Statement 2 ALONE is sufficient to answer the question, but Statement 1 ALONE is NOT sufficient to answer the question.
BOTH statements TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient to answer the question.
Statement 1 ALONE is sufficient to answer the question, but Statement 2 ALONE is NOT sufficient to answer the question.
EITHER statement ALONE is sufficient to answer the question.
BOTH statements TOGETHER are insufficient to answer the question.
BOTH statements TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient to answer the question.
Consider the area formula for a trapezoid:


So, if you know both the area and 
Example Question #181 : Data Sufficiency Questions
Consider parallelogram 
I) The perimeter of 

II) Side 


Find the length of side 
Both statements are needed to answer the question.
Statement I is sufficient to answer the question, but statement II is not sufficient to answer the question.
Neither statement is sufficient to answer the question. More information is needed.
Either statement is sufficient to answer the question.
Statement II is sufficient to answer the question, but statement I is not sufficient to answer the question.
Both statements are needed to answer the question.
We can work backwards from the perimeter to find the length of the unknown side.
I) Gives us the perimeter.
II) Gives us two of the sides.
In a parallelogram there are two sets of corresponding sides. We can use I) and II) to write the following equaiton, where l is the length of our wanted side.
Solve for l to find our final side:
Example Question #4 : Dsq: Calculating The Length Of The Side Of A Quadrilateral
Calculate the side of a square.
Statement 1: A circle with an area of 
Statement 2: A circle with a circumference of 
Statement 1: A circle with an area of 
Write the formula for the area of the circle.
After substituting the value of the area and solve for radius, the radius is 1. The side length of the square is double the length of the radius.
Statement 2: A circle with a circumference of 
Write the formula for the circumference of the circle.
After substituting the value of the circumference and solve for diameter, the diameter of the circle is 1. This diameter represents the diagonal of the square. Because the square length and width are equal dimensions, it is possible to solve for the lengths of the square by Pythagorean Theorem knowing just the length of the square diagonal.
Therefore:
Example Question #182 : Data Sufficiency Questions
Consider isosceles trapezoid 
I) 

II) The larger base of 
Find the length of the two legs of 
Statement I is sufficient to answer the question, but Statement II is not sufficient to answer the question.
Statement II is sufficient to answer the question, but Statement I is not sufficient to answer the question.
Either statement is sufficient to answer the question.
Neither statement is sufficient to answer the question. More information is needed.
Both statements are needed to answer the question.
Neither statement is sufficient to answer the question. More information is needed.
Consider isosceles trapezoid 
I) 

II) The larger base of 
Find the length of the two legs of 
To find the length of the legs of a trapezoid, we need the perimeter and the two bases. Then, we can subtract the sum of the two bases from the perimeter and divide by two to find the lengths of the sides we are looking for.
Statement I gives us the perimeter of 
Statement II relates the two bases of 
We are told that 
We still have three unknowns and two equations, so we cannot solve this system of equations.
Example Question #21 : Quadrilaterals
What is the perimeter of Rhombus 
Statement 1: 

Statement 2: 
Statement 1 ALONE is sufficient to answer the question, but Statement 2 ALONE is NOT sufficient to answer the question.
Statement 2 ALONE is sufficient to answer the question, but Statement 1 ALONE is NOT sufficient to answer the question.
EITHER statement ALONE is sufficient to answer the question.
BOTH statements TOGETHER are insufficient to answer the question.
BOTH statements TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient to answer the question.
BOTH statements TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient to answer the question.
Each diagonal divides Rhombus 
Statement 1 alone establishes the perimeter of one such triangle. However, it does not make it clear what equal side lengths 




Statement 2 alone gives no information about the actual lengths of the sides.
Assume both statements are true. Since 






Since 


Example Question #183 : Data Sufficiency Questions

What is the perimeter of quadrilateral 
(1) Diagonal 


(2)
Each statement alone is sufficient
Statement 1 alone is sufficient
Statements 1 and 2 together are not sufficient
Both statements together are sufficient
Statement 2 alone is sufficient
Both statements together are sufficient
To find the perimeter of the quadrilateral, we need to know whether it is of a special type of quadrilaterals and we need to know the length of the sides.
Statement 1 tells us only that the quadrilateral is a rhombus. Indeed, a quadrilateral with perpendicular diagonals intersecting at their midpoint must be a rhombus. However we don't know any length of the sides.
Statement 2 says gives us the length us two consecutive sides. It could be tempting to answer that it is sufficient, however, we can't conclude that the quadrilateral has equal lengths. Therefore this statement alone is insufficient.
Both statements together are sufficient since we can conclude that the quadrilateral is a rhombus, and twice 
All GMAT Math Resources




































